Complete Solutions to Level 3 of chapter CURRENT ELECTRICITY of Class 12 book with complete answers and questions
| Class 12 | PHYSICS |
| Chapter | CURRENT ELECTRICITY |
| NARAYANA | Level 3 |
| Number Of Questions | 46 |
| Academic Year | 2023-24 |
The electron of hydrogen atom is considered to be revolving around the proton in circular orbit of radius `(h^(2))/(me^(2))` with velocity `(e^(2))/(h)`, where `h=(h)/(2pi)`. The curret I is
View SolutionIn a straight conductor of uniform cross-section charege q is flowing for time t. Let s be the specific charge of an electron. The momentum of all the free electrons per unit length of the free electrons per unit length of the conductor, due to their drift velocity only is
View SolutionPotential difference of 100 V is applied to the ends of a copper wire one metre long. Calculate the average drift velocity of the electrons ? Compare it with thermal velocity at `27^@C`. Consider there is one conduction electron per atom. The density of copper is `9.0xx10^(3)kg//m^(3)`, Atomic mass of copper is 63.5 g. Avogadro's number `= 6.0 xx 10^(23)` per gram-mole. Conductivity of copper is `5.81 xx 10^(7)Omega^(-1)m^(-1)`. Boltzmann constant `= 1.38 xx10^(23)JK^(-1)`.
View SolutionThe sides of rectangular block are 2 cm, 3 cm ad 4 cm. The ratio of the maximum to minimum resistance between its parallel faces is
View SolutionFind the equivalent resistance across `AB` :
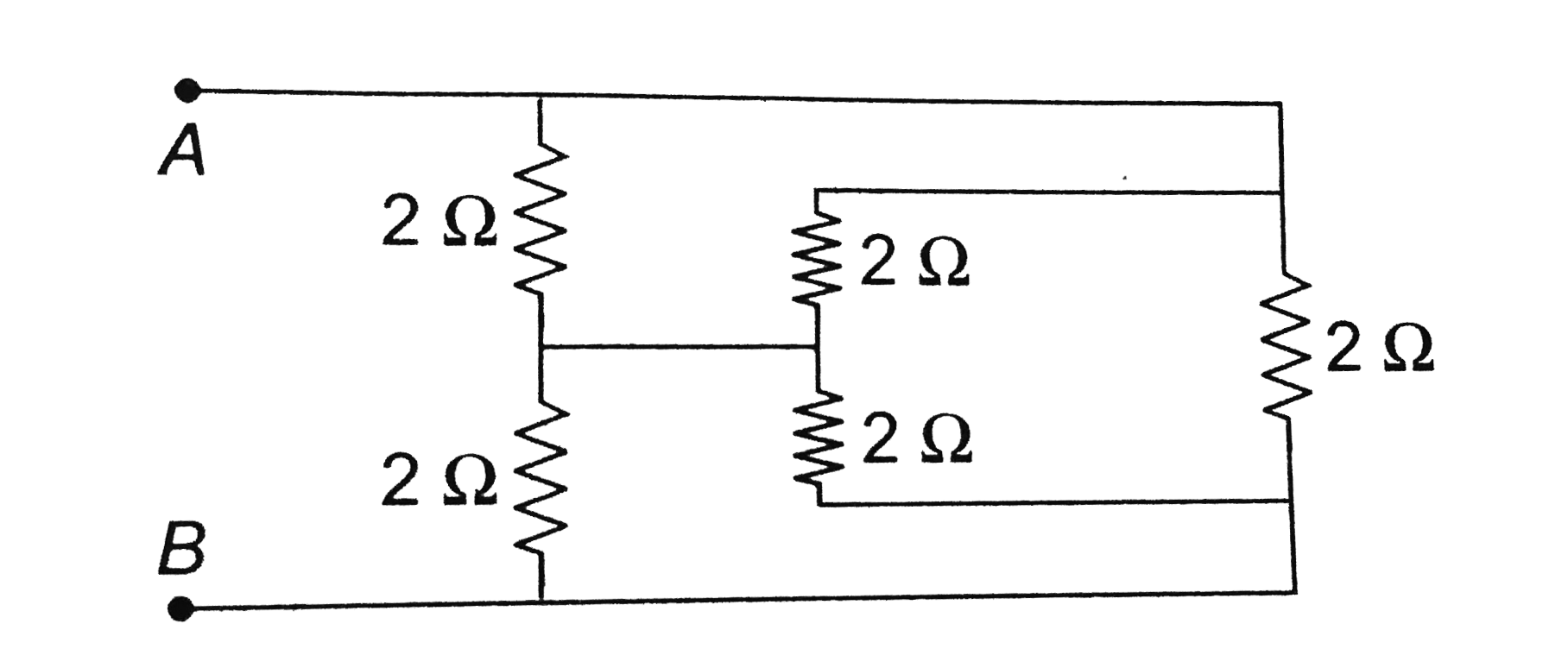
View SolutionTwo wires of the same material have length 6 cm and 10 cm and radii 0.5 mm and 1.5 mm respectively. They are connected in series across a battery of 16 V. the p.d. across the shorter wire is
View SolutionThree ammeters P,Q and R with internal resistances r, 1.5r, 3r respectively. Q and R parallel and this combination is in series with P, the whole combination is in series with P, T the whole combination connected between X and Y. When the battery connected between X and Y, the ratio of the readings of P, Q and R is
View Solution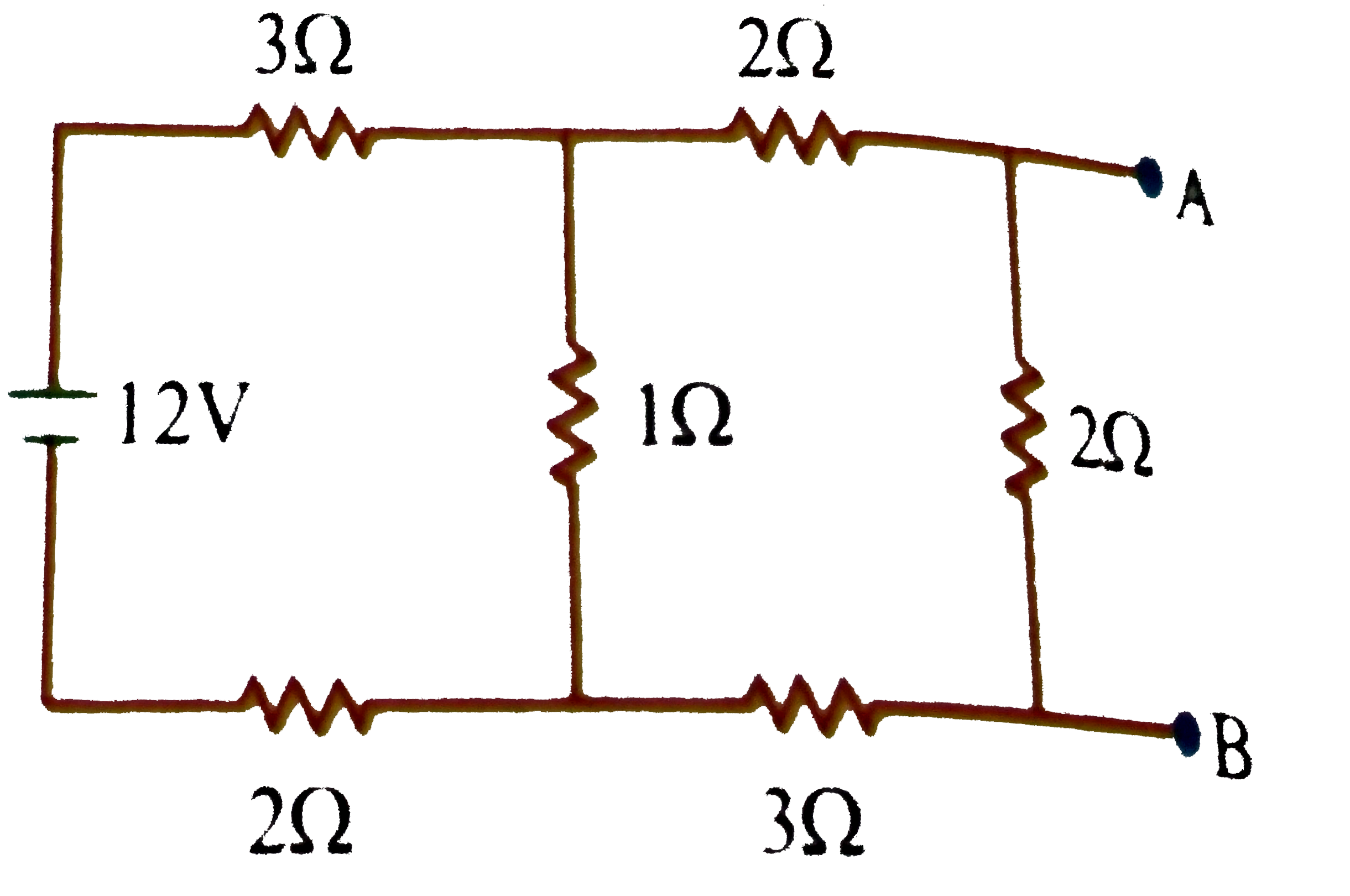
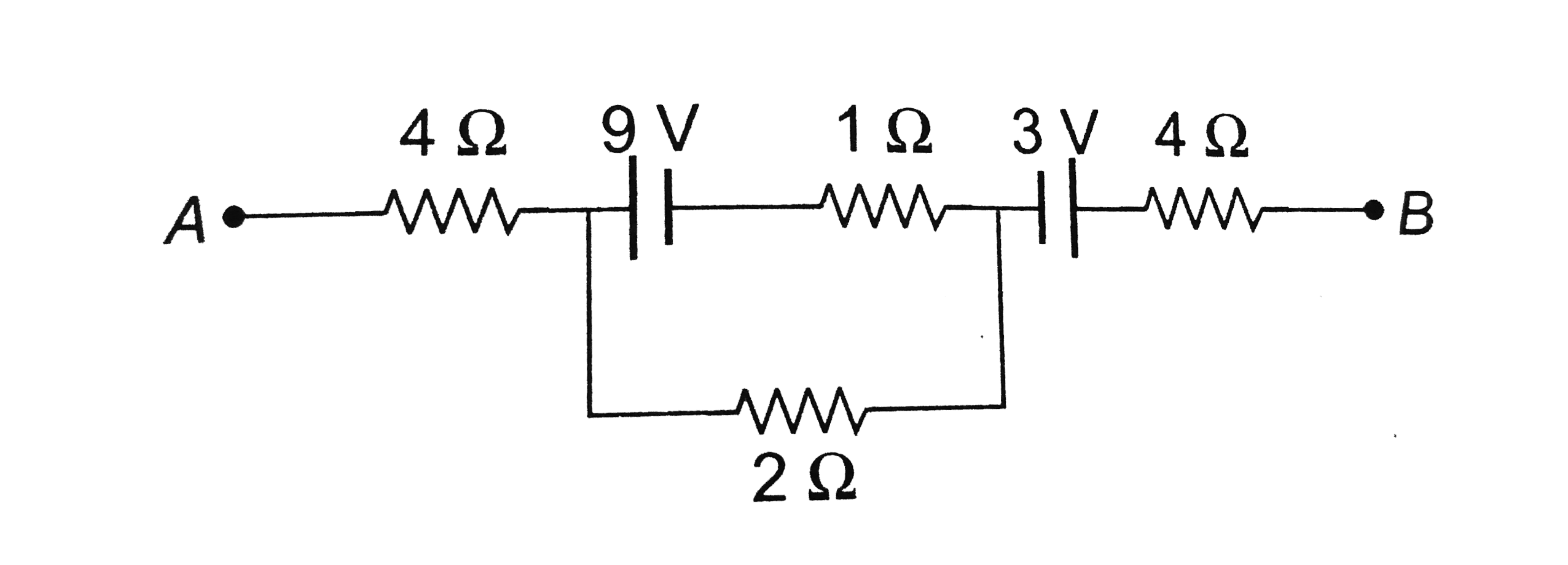
The potential difference between points A and B is
View Solution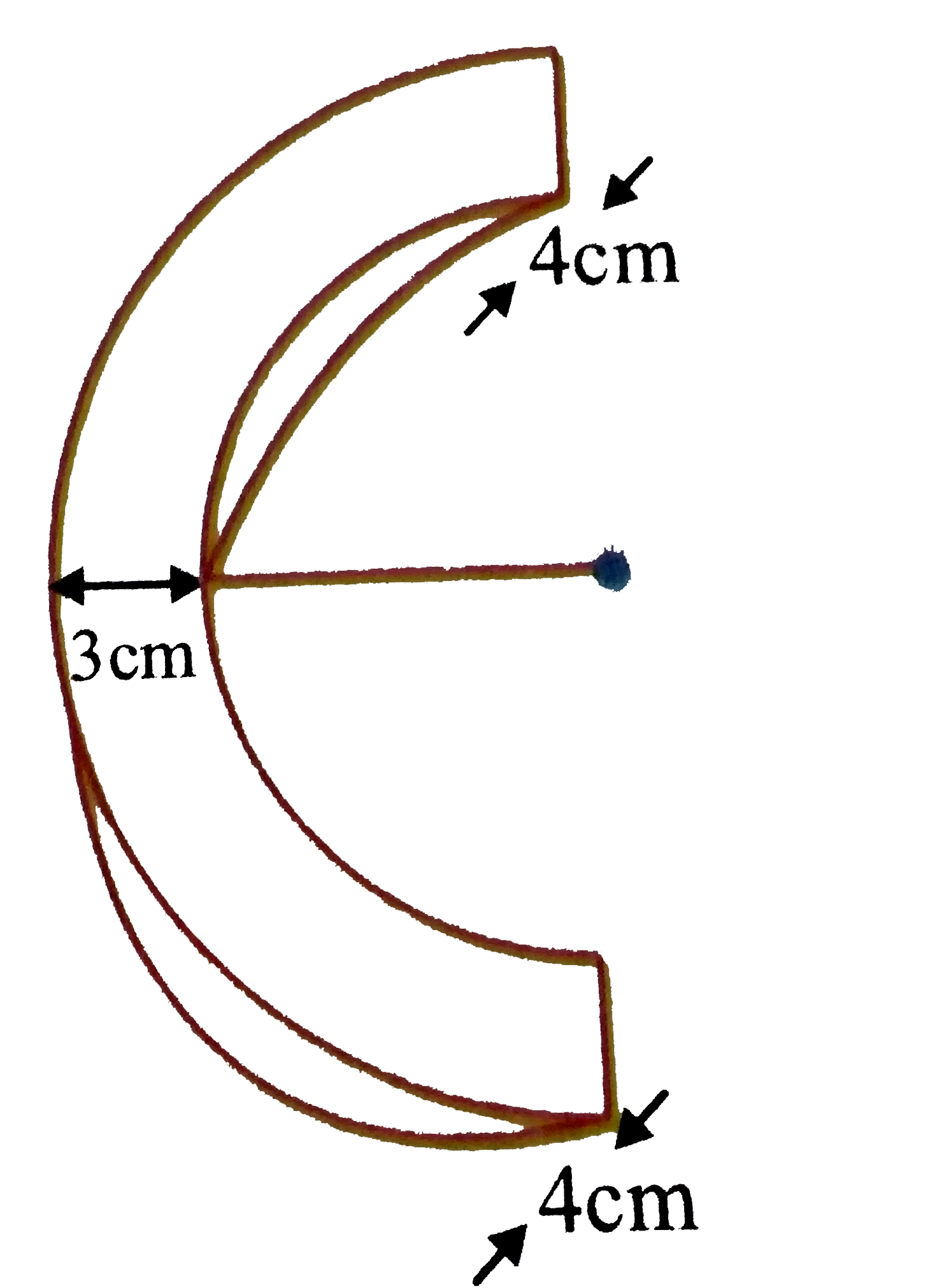
The resistance of a semicircle shown in fig. Between its two end faces is (Given that radial thickness `=3cm`, axial thickness `=4cm`, inner radius `=6cm` and resistivity `=4xx10^(-6)Omegacm`)
View SolutionABCD is a square where each side is a uniform wire of resistance `1Omega.` A point E lies on CD such that if a uniform wire of resistance `1Omega` is connected across AE and constant potential difference is applied across A and C, then B and E are equipotential. Then,

View SolutionA heating element using nichrome connected to a 230 V supply draws an initial current of 3.2 A which settles after a few seconds to a steady value of 2.8 A. What is the steady temperature of the heating element if the room temperature is `27^(@)C` ? Temperature coefficient of resistance of nichrome averaged over the temperature range involved is `1.70xx10^(-4) C^(-1)`.
View Solution
A group of N cells where e.m.f. varies directly with the internal resistance as per the equation `E_(N)=1.5r_(N)` are connected as shown in the figure. The current I in the circuit is:
View SolutionCell A has emf 2 E ad internal resistance 4 r. Cell B has emf E and internal resistance r. The negative of A is connected to the positive of B and a load resistance of R is connected across the battery formed. If the terminal potential difference across A is zero, then R is equal to
View SolutionIn the circuit shown in figure potential difference between point `A` and `B` is `16 V`. Find the current passing through `2 Omega` resistance.
View SolutionFind the minimum number of cells required to produce a current of `1.5A` through a resistance of `30Omega.` Given that the emf of each cell is `1.5V` and the internal resistance is `1Omega`.
View Solution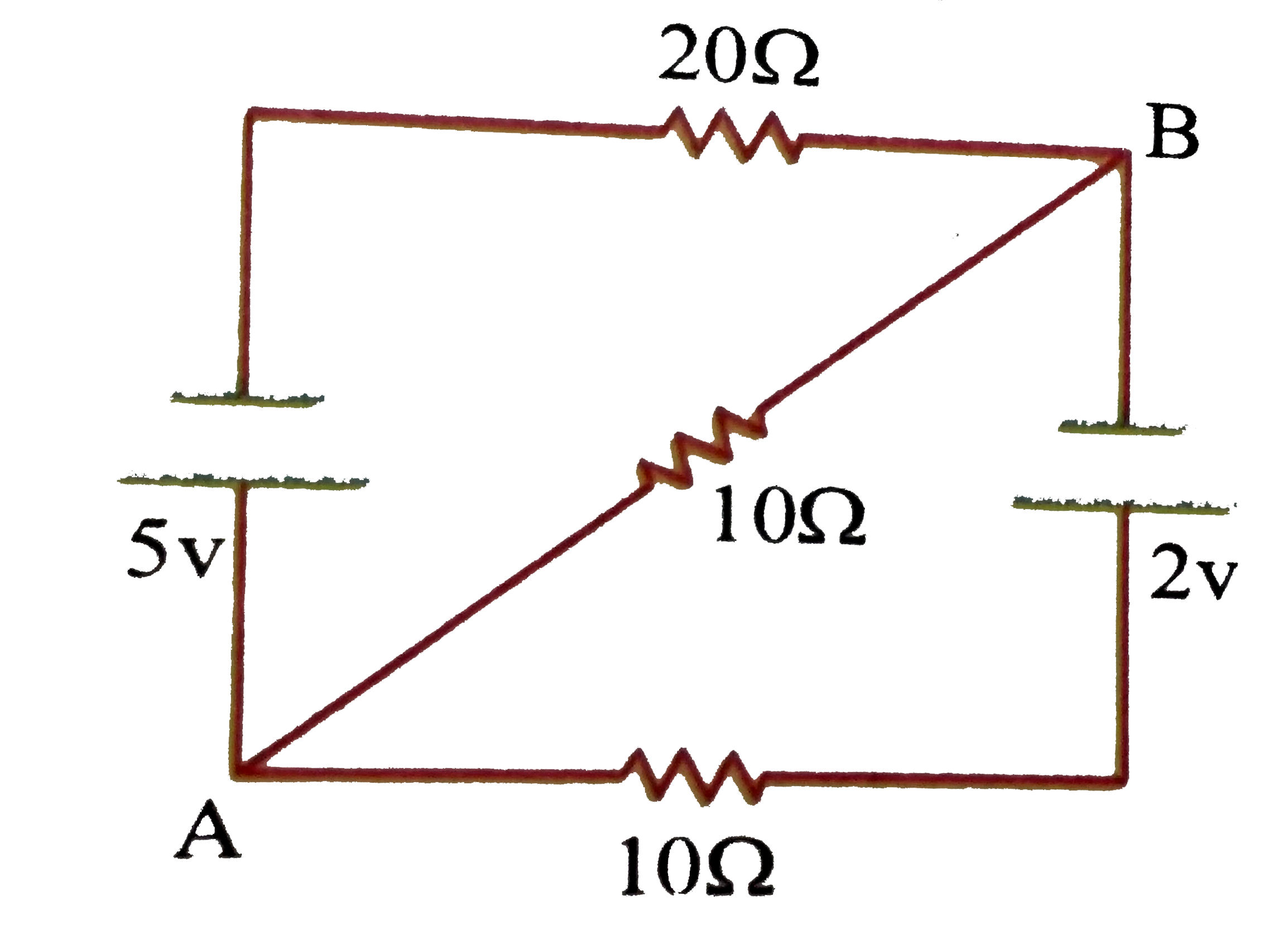
The p.d between the terminals A & B is
View Solution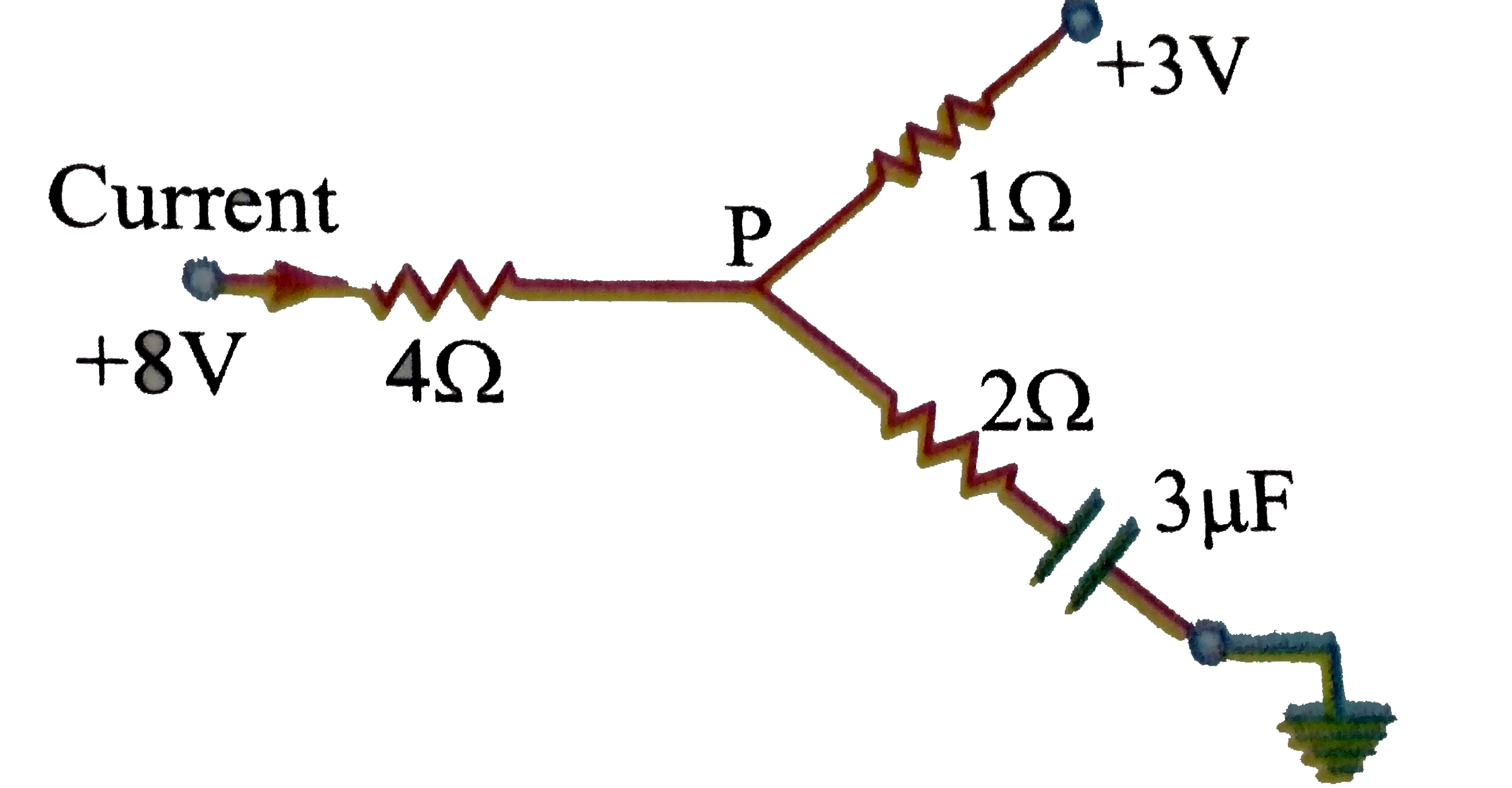
the energy stored in the capacitor is
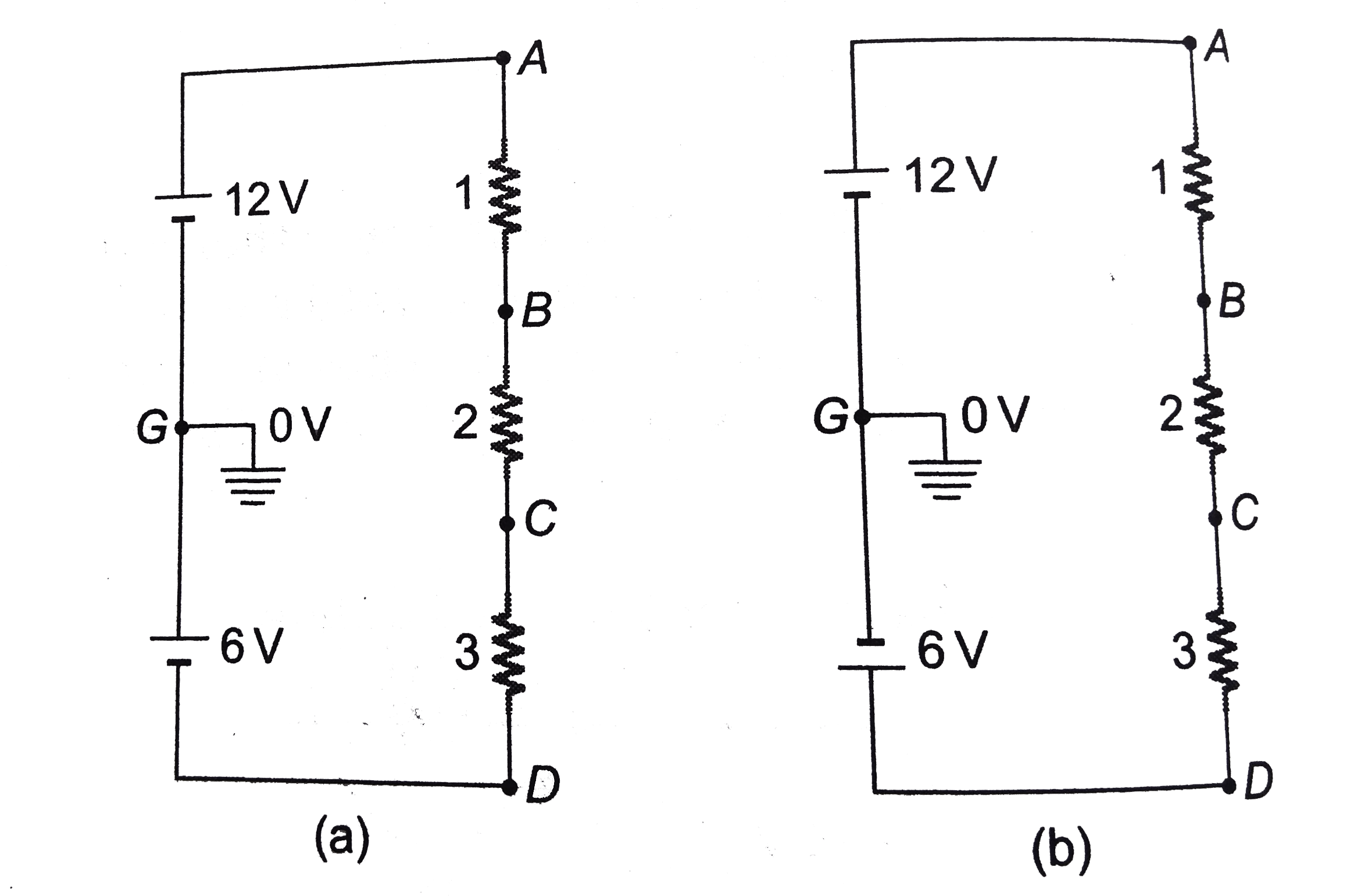
View SolutionCalculate the potentials of points `A, B,C` and `D` as shown in Fig. a. What would be the new potential values if connections of `6 V` battery are reversed as shown in fig b. All resistance are on ohm.
View SolutionIn the circuit in fig. If no current flows through the galvanometer when the key k is closed, the bridge is balanced. The balancing condition for bridge is
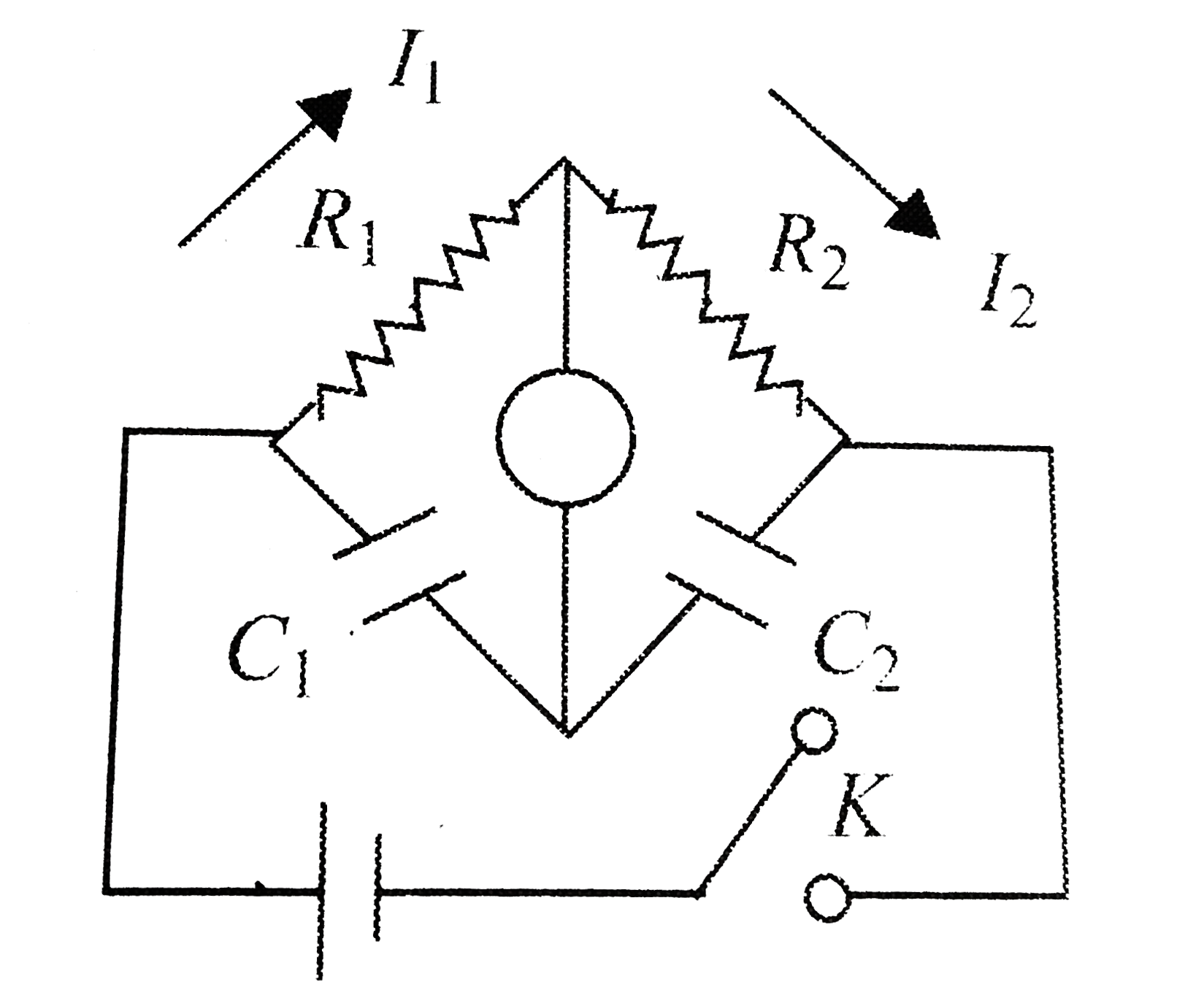
View Solution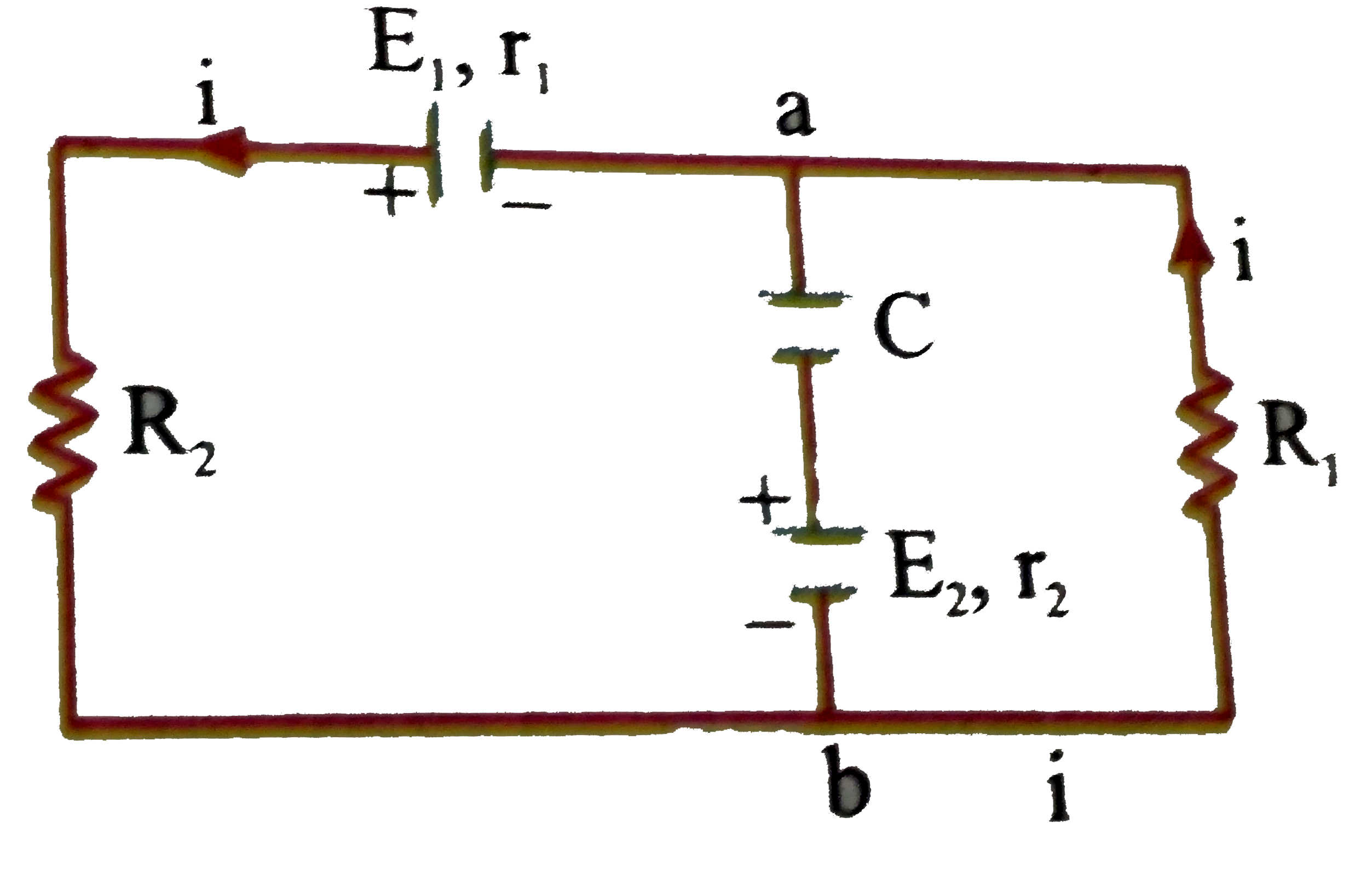
In the steady state, the energy stored in the capacitor is:
View SolutionA part of circuit in a steady state along with the currents flowing in the branches, the values of resistance etc., is shown in the figure. Calculate the energy stored in the capacitor C (4muF)`
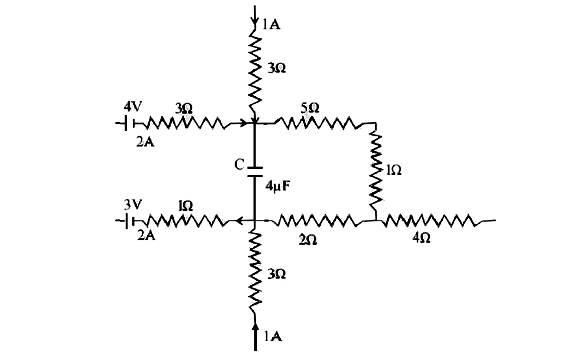
View Solution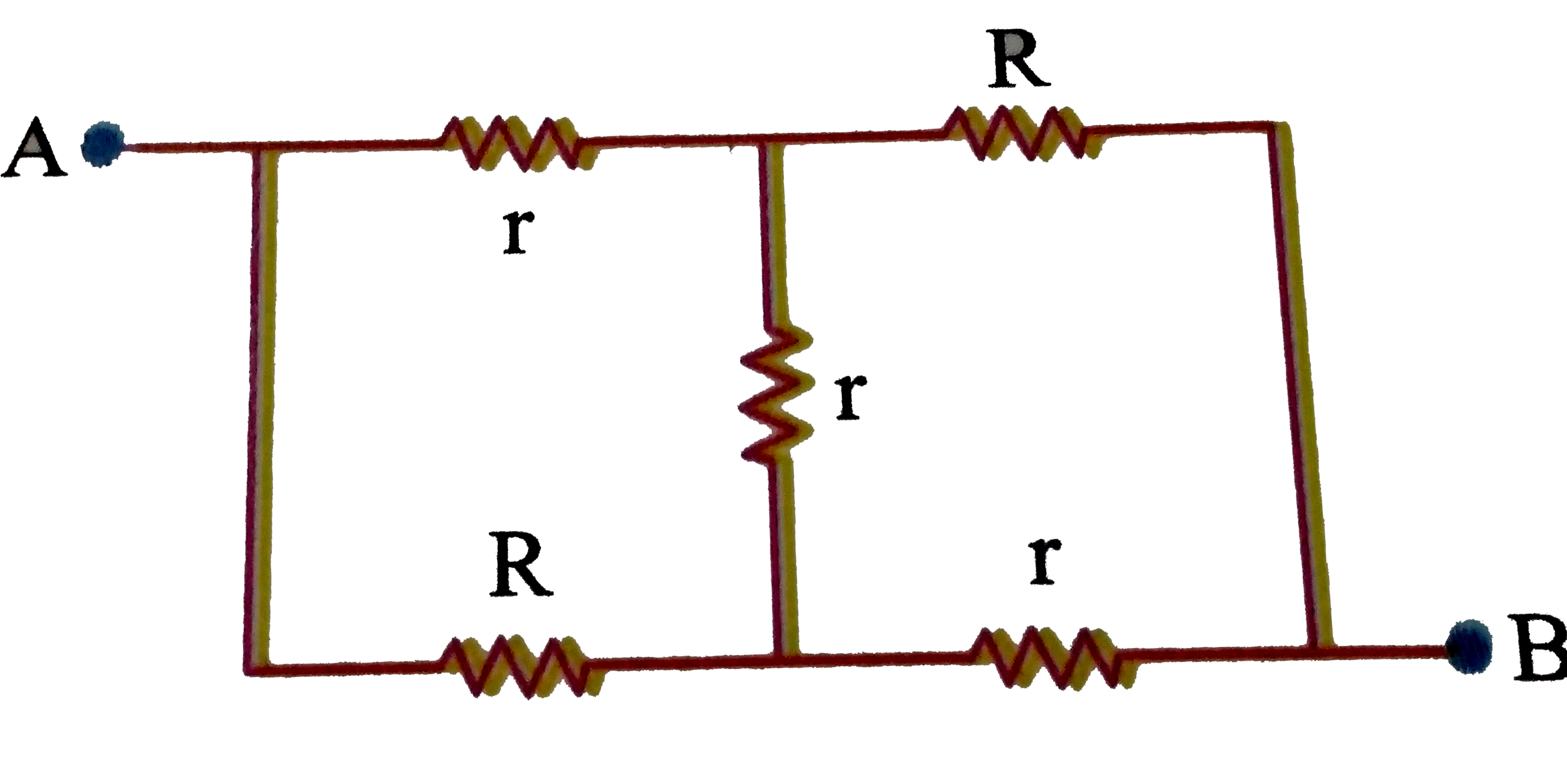
Equivalent resistace across A and B in the given circuit if `r=10Omega,R=20Omega` is
View Solutionfor a cell, the graph between the p.d. (V) across the terminals of the cell ad te current I drawn from the cell is shown in the fig. the emf and the internal resistance of the cell is E and r respectively.
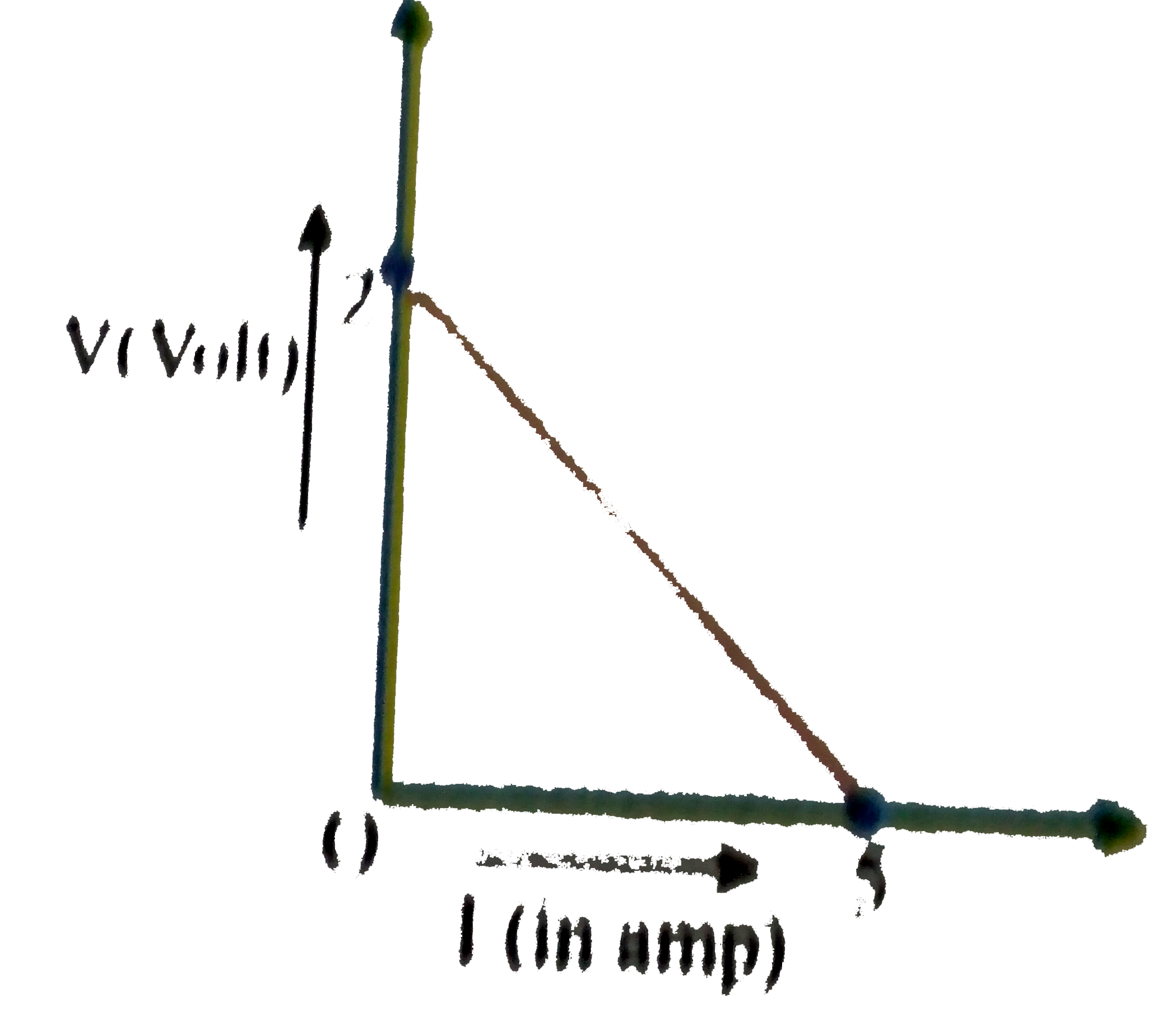
View Solution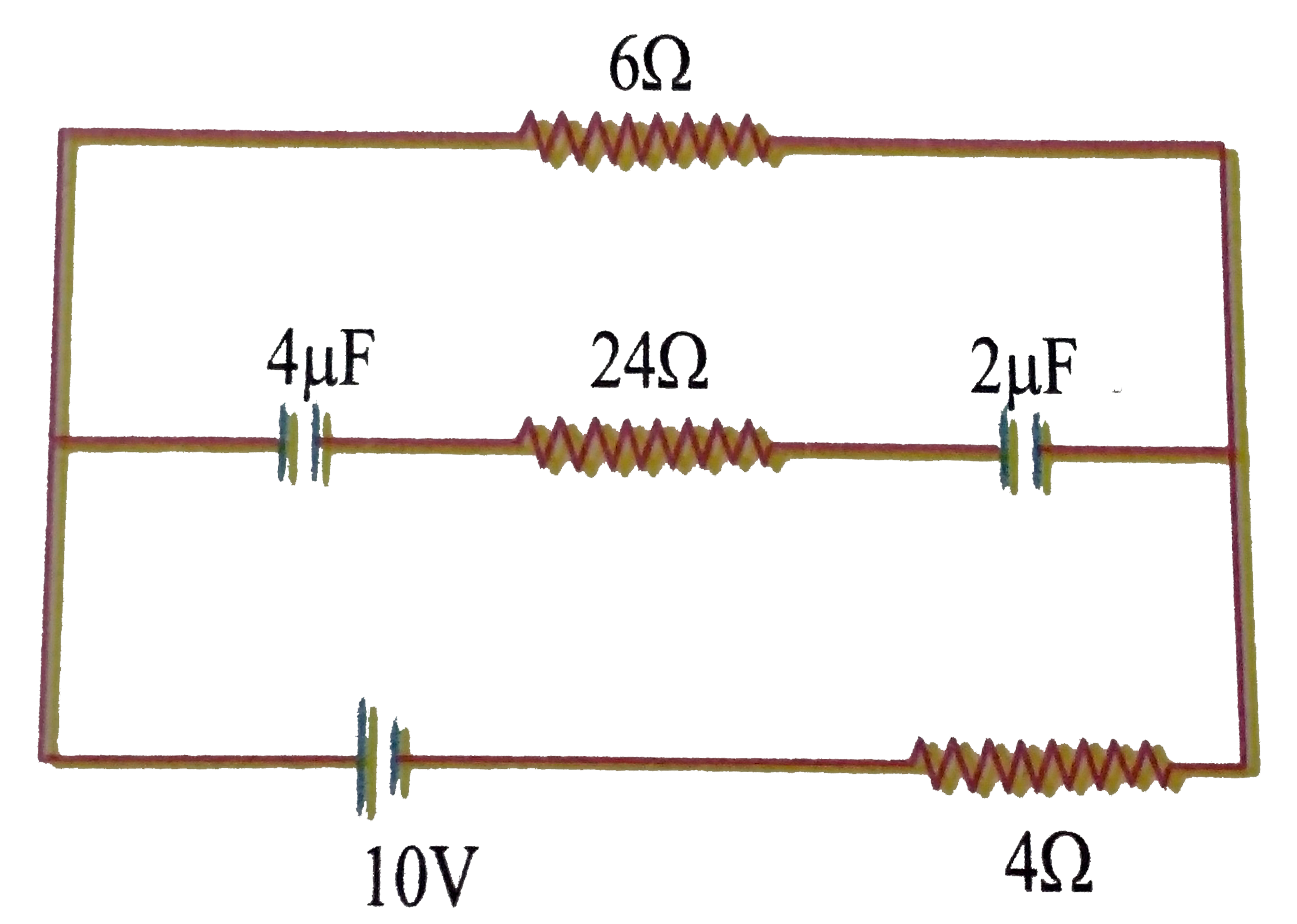
The charge developed on `4muF` condenser is
View SolutionFind out the value of current through `2Omega` resistance for the given circuit.
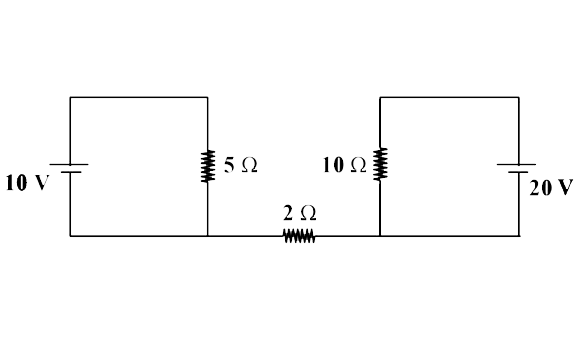
View SolutionSame mass of copper is drawn into 2 wires of 1 mm thick ad 3 mm thick. Two wires are connected in series and current is passed. Heat produced in the wires is the ratio of
View SolutionMasses fo three are in the ratio `1:3:5` their lengths are in the ratio `5:3:1` when they are connected in series to an external source, te amounts of heats produced in them are in the ratio
View SolutionA heater coil rated at 1000 W s connected to a 110 V mains. How much time will take to melt 625 gm of ice at `0^(@)C`. (for ice `L=80(Cal)/(gm)`)
View Solution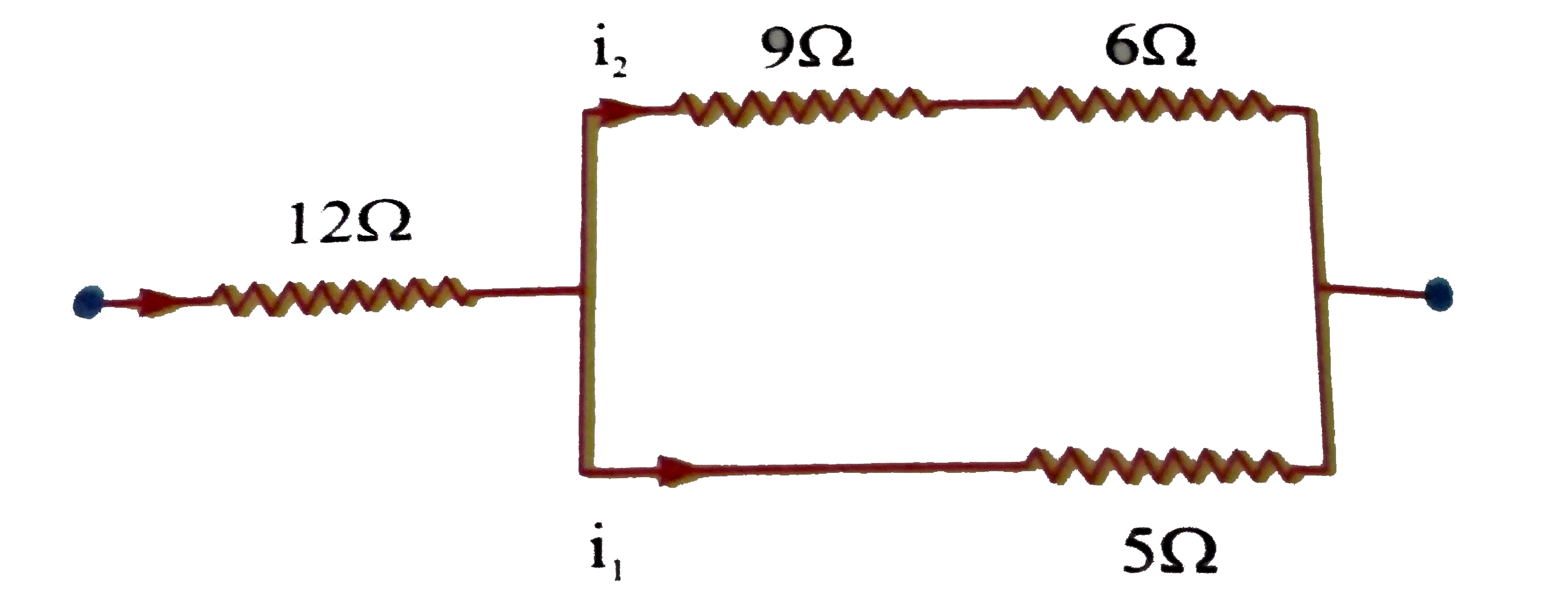
In the following circuit, `5Omega` resistor develops 45 J/s due to current flowing through it. The power developed across `12Omega` resistor is
View SolutionTwo wires `'A'` and `'B'` of the same material have their lengths in the ratio `1 : 2` and radii in the ratio `2 : 1` The two wires are connected in parallel across a battery. The ratio of the heat produced in `'A'` to the heat produced in `'B'` for the same time is
View SolutionAn electric motor operating on 50 volt D.C. supply draws a current of 10 amp. If the efficiency of motor is `40%` then the resistance of the winding of the motor is
View SolutionFind the resistance of `240 V - 200` watt electric bulb when glowing. If this resistance is `10` times the resistance at `0^(@)C` and the temperature of the glowing filament is `2000^(@)C`, then find the temperature coefficient of resistance of the filament.
View SolutionA wire of length L and 3 identical cells of negligible internal resistance are connected in series. Due to the current, the temperature of the wire is raised by `DeltaT` in a time t. A number N of similar cells is now connected in series with a wire of the same material and cross-section but of length 2L. The temperature of the wire is raised by the same amount `DeltaT` in the same time t. the value of N is
View Solution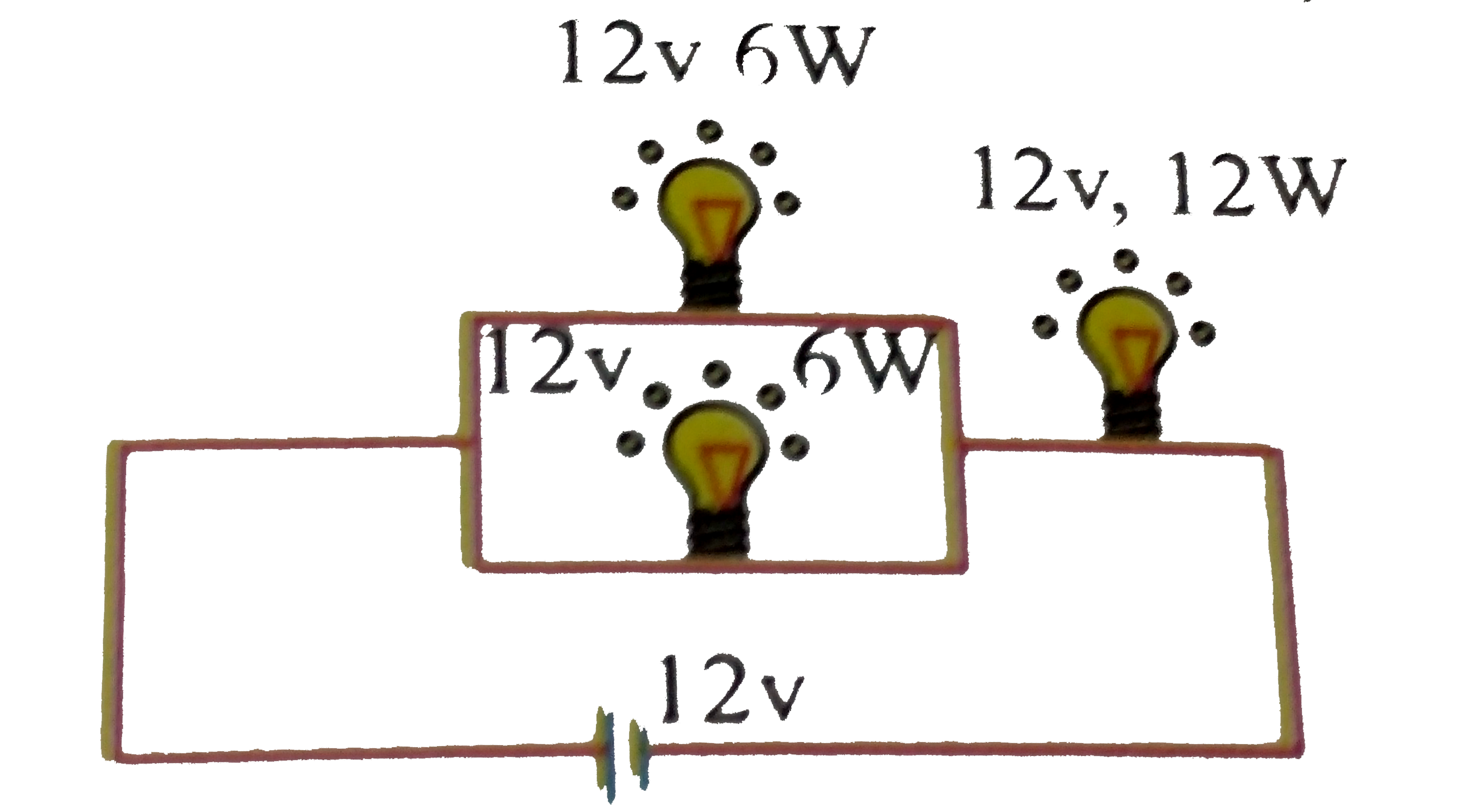
Three bulbs with their power and working voltage are connected as shown in the circuit diagram to a 12 V battery. The total power consumed by the bulbs is (ignore the internal resistance of the battery shown)
View SolutionA cell of emf 12 V and internal resistance `6Omega` is connected in parallel with another cell of emf 6 V ad internal resistance `3Omega`, such that the positive of the first cell joins the positive to the second cell and similarly the negative of first cell joins the negative of the second cell. A bulb of filament resistance `14Omega` is connected across the combination. The power delivered to be bulb is
View SolutionA cell develops the same power across two resistances `R_(1)` and `R_(2)` separately. The internal resistance of the cell is
View SolutionA metallic conductor at `10^(@)C` connected in the left gap of meter bridge gives balancing length 40 cm. When the conductor is at `60^(@)C`, the balancing point shifts by --- cm, (temperature coefficient of resistance of the material of the wire is `((1)/(220))/(.^(@)C))`
View SolutionWhen a conducting wire is connected in the right gap and known resistance in the left gap, the balancing length is 60 cm. the balancing length becomes 42.4 cm when the wire is stretched so that its length increases by
View Solution`n` identical resistors are taken `(n)/(2)` resistors are connected in series and the remaining are connected in paralle. The series connected group is kept in the left gap of a meter bridge and the parallel connected group in the right gap. The distance of te balance point from the left end of the wire is
View SolutionIn a metere bridge, the balance length from left end (standard resistance of `1Omega` is in the right gap) is found to be 20 cm, the length of resistance wire in left gap) is found to be 20 cm the length of resistance wire in left gap is `(1)/(2)` m and radius is 2mm its specific resistance is
View SolutionIn an experiment with a potentiometer to measure the internal resistance of a cell. when the cell in the secondary circuit is by shounted by `5 Omega`, the null point is at `220 cm`. When the cell is shunted by `20 Omega ` the null point is at `300 cm`. Find the internal resistance of the cell.
View SolutionA potentiometer wire of lengthh 100 cm has resistance `5Omega`. It is connected in series with a resistance and a cell of emf 2 v and of negligible internal resistance. A source of emf 5 mv balanced by 10 cm length of potentiometer wire. The value of external resistance is___
View Solution1 ohm resistance is in series with a Ammeter which is balanced by 75 cm of potentiometer wire. A standard cell of 1.02 V is balanced by 50 cm.t he ammeter shows a reading of 1.5 A. The error in the ammeter reading is
View SolutionA potentiometer wire of lengthh 100 cm has resistance `5Omega`. It is connected in series with a resistance and a cell of emf 2 v and of negligible internal resistance. A source of emf 5 mv balanced by 10 cm length of potentiometer wire. The value of external resistance is___
View SolutionIn an experiment for calibration of voltmeter, standard cell of emf 1.5 V is balanced at 300 cm length of potentiometer wire. The P.D. Across a resistace in the circuit is balanced at 1.25 m. if a voltmeter, it reads 0.65 V. The error I te volt meter is
View Solution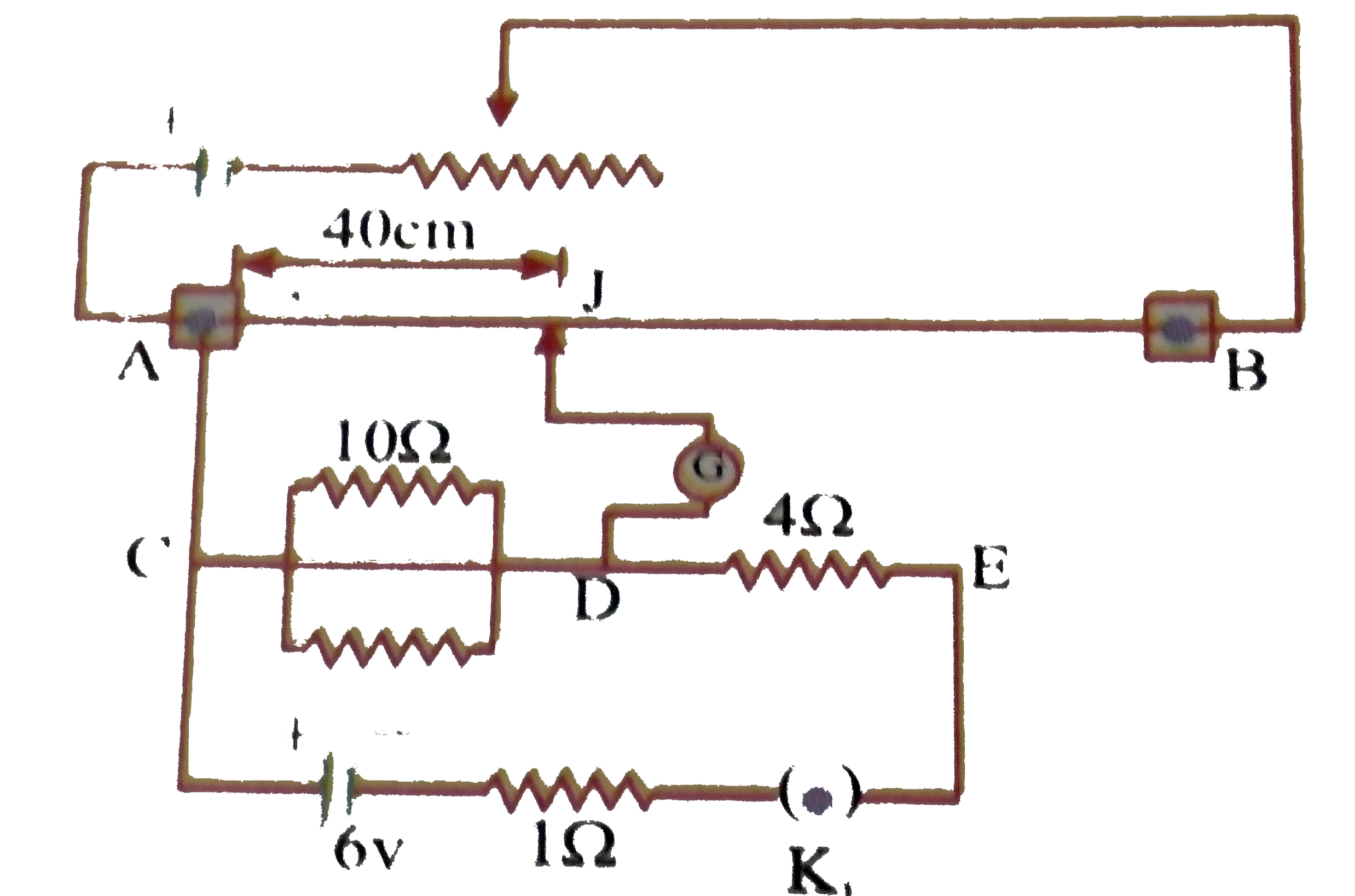
In the circuit shown in fig. the potential difference between the points C and D is balanced against 40 cm length of potentiometer wire of total length 100 cm. In order to balance the potential difference between the points D and E The jockey to be pressed on potentiometer wire at a distance of
View Solution
Level 3 questions and complete solutions for chapter CURRENT ELECTRICITY of NARAYANA PHYSICS (HINGLISH) of Class 12