Complete Solutions to SAMPLE PROBLEM of chapter ELECTRIC CHARGES AND FIELDS of Class 12 book with complete answers and questions
| Class 12 | PHYSICS |
| Chapter | ELECTRIC CHARGES AND FIELDS |
| RESNICK AND HALLIDAY | SAMPLE PROBLEM |
| Number Of Questions | 20 |
| Academic Year | 2023-24 |
Two positively charged particles fixed in place on an x axis. The charges are `q_(1)=1.60 xx 10^(-19)C and q_(2)=3.20xx10^(-19)C`, and the particle separation is R = 0.0200m. What are teh magnitude and direction of the electrostatic force `vecF_(12)` on particle 1 from particle 2?
View SolutionFigure 22-8c is identical to Fig. 22-8a except that particle 3 now lies on the x axis between particles 1 and 2. Particle 3 has charge `q_(2)= -3.20 xx 10^(-19)C` and is at a distance `(3//4)`R from particle 1. What is the net electrostatic force `vecF_("1.net")` on particle 1 due to particles 2 and 3?
View SolutionFigure 22-8e is identical to Fig 22-8a except that particle 4 is now included. It has charge `q_(4)= -3.20 xx 10^(-19)C`, is at a distance `(3)/(4)` R from particle 1, and lies on a line that makes an angle `theta=60^(@)` with the x axis. What is the net electrostatic electrostatic electrostatic force `vecF_("1, net")` on particle 1 due to particle 2 and 4?
View SolutionTwo charges +8q and -2q are fixed on X-axis at origin and `x=+a` locations. A third charge +q is to be located on X-axis (other than infinitely far away) so that it is in equilibrium. The location of the third charge is correctly represented by
View SolutionTwo identical, small, charged spheres, each having a mass of `4.0xx10^(-2)`kg hang in equilibrium as shown in Fig. 22-10a. The length of each string is 1.5m and the angle `theta` is `37.0^(@)`. Find the magnitude of the charge on each sphere.
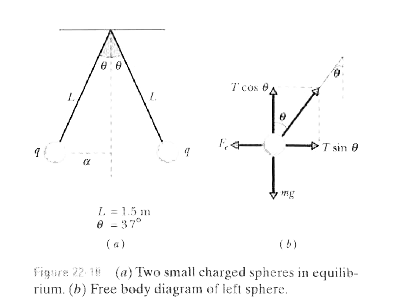
View SolutionThe nucleus in an iron atom has a radius of about `4.0xx10^(-15)`m and contains 26 protons.
What is the magnitude of the repulsive electrostatic force between two of the protons that are separated by `4.0xx10^(-15)m`?
View SolutionThe nucleus in an iron atom has a radius of about `4.0xx10^(-15)`m and contains 26 protons.
What is the magnitude of the gravitational force between those same two protons?
View SolutionFigure 22-18a shows there particles with charges `q_(1)=+2Q, q_(2)=-2Q, and q_(3)=-2Q`, each a distance d from the origin. What net electric field `vecE` is produced at the origin?
View SolutionIn Fig. 22-19, particle 1 of charge `q_(1)=5.00q` and a particle 2 of charge `q_(2)+2.00q` are fixed on the x-axis.
As a multiple of distance L, at what coordinate on the axis is the net electric field of the particles zero?
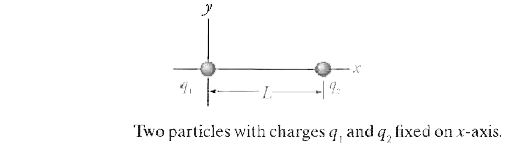
View SolutionSketch the net electric lines between and around the particles.
View SolutionFigure 22-24 shows a plastic rod having a uniformly distributed charge. The charge per unit length of the rod is `lambda`. Find the electric field due to the rod at a point P which is at a distance r from the rod.
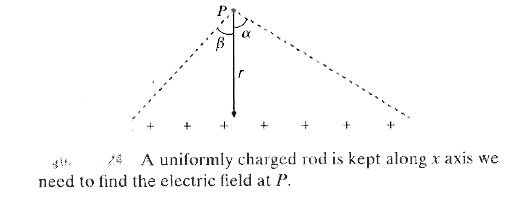
View SolutionFigure 22-26a shows a plastic rod with a uniform charge -Q. It is bent in a `120^(@)` circular arc of radius r and symmetrically paced across an x axis with the origin at the center of curvature P of the rod. In terms of Q and r, what is the electric field `vecE` due to the rod at point P?
View SolutionFind the electric field at the center of the sphere due to the induced charges on the surface of the sphere, as shown in Fig. 22-30.

View SolutionBeams of high-speed protons can be produced in "guns" using electric fields to accelerate the protons.
What acceleration would a proton experience if the gun's electric field were `2.00xx10^(4)N//C`.
View SolutionBeams of high-speed protons can be produced in "guns" using electric fields to accelerate the protons.
What speed would the proton attain if the field accelerated the proton through a distance of 1.00 cm?
View SolutionFigure shows an assembly of deflecting plates A and B ofan ink-jet printer which causes moving ink droplets to deflect at desired displacements by continuously varying electric field between the plates. An ink drop with a mass `m = 1.3 xx 10^(-10)kg` and a negative charge of magnitude `q = 1.5x x 10^(-13)C` enters the region between the plates, initially moving along the x-axis with speed `v_(x), = 10m//s`. The length of plates is `L = 1.6cm`. The plates are connected with a varying voltage and thus produce an electric field at all points between them. Assume that field `vec(E)` for some duration is constant and it is acting in downward direction as shown and has a magnitude of `E = I .4 xx 10^(6) N//C`, find the vertical deflection of the drop at the far edge ofthe plate? As the gravitational force on the drop is very small relative to the electrostatic force acting on the drop, it can be neglected for this analysis. `[6.4 xx 10^(-4)m]`

View SolutionA neutral water molecule `(H_(2)O)` in its vapor state has an electric dipole moment of magnitude `6.2xx10^(-30)C*m`.
How far apart are the molecule's centers of positive and negative charge?
View SolutionA neutral water molecule `(H_(2)O)` in its vapor state has an electric dipole moment of magnitude `6.2xx10^(-30)C*m`.
If the molecule is placed in a electric field of `1.5xx10^(4)N//C`, what maximum torque can the field exert on it? (Such a field can easily be set up in the laboratory.)
View SolutionA neutral water molecule `(H_(2)O)` in its vapor state has an electric dipole moment of magnitude `6.2xx10^(-30)C*m`.
How much work must an external agent do to rotate this molecule by `180^(@)` in this field, starting from its fully aligned position , for which `theta=0`?
View SolutionFind the electric field at (A) (a) due to q, (b) due to charges induced on the inner surface of the shell and (c ) due to charges induced on the outer surface of the shell (Fig. 22-41).
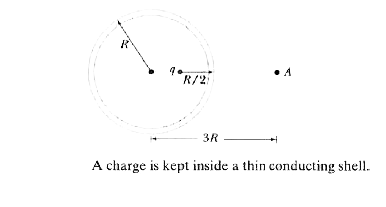
View Solution
SAMPLE PROBLEM questions and complete solutions for chapter ELECTRIC CHARGES AND FIELDS of RESNICK AND HALLIDAY PHYSICS (HINGLISH) of Class 12