Complete Solutions to Sample problem of chapter GEOMETRICAL OPTICS : REFRACTION of Class 12 book with complete answers and questions
| Class 12 | PHYSICS |
| Chapter | GEOMETRICAL OPTICS : REFRACTION |
| RESNICK AND HALLIDAY | Sample problem |
| Number Of Questions | 29 |
| Academic Year | 2023-24 |
Lateral displacement of ray passing through a glass slab
A ray of light is incident on a parallel sided glass slab of refractive index n and thickness t as shown in Fig. 34-2. Find the lateral displacement of the ray as it passes through the slab.
View SolutionRefraction of ray passing through tank filled with water
An observer is viewing along the line shown in Fig. 34-4. When there is no water filled in the tank, he can see only wall AB and no other part of the base of the tank. Then water is filled in till height h and he is just able to see the point E as shown in Fig. 34-3. Find the angle of refraction into air `(phi)` and angle of incidence from water `(phi)`. Also find the depth to which water has been filled.
View SolutionRefraction of ray incident on a boundary of another plane
A ray given by `-hati-2hatj` is incident on xz plane as shown in Fig. 34-4. Above the xz plane, refractive index is `n_(1)=2` and below the xz plane refractive index is `n_(2)=sqrt(5//2)`. Find a unit vector in the direction of the refracted ray.
View SolutionIf a fish lies at the bottom of a 4 m deep water tank `(mu = 4//3)` and a bird is flying at a height of 6m above the water surface, then apparent distance at which the fish appears to the bird is
View SolutionApparent distance of the image formed by a concave mirror dipped in water
Find the location of the final image of O formed by the system (Fig. 34-8). Assume that O is on the principal axis of the concave mirror.
View SolutionApparent velocity of fish floating in tank
A tank is filled with water up to a height of `15cm` and a fish is floating inside at a depth of 10cm. The tank is now being drained so that the surface moves down with a velocity of 1cm/s. An observer is viewing the fish from a height of 10cm.
(a) Find the apparent velocity of the fish as seen by the observer.
Find the apparent velocity of the observer as seen by the fish.
View SolutionLocation of image after multiple events
Find the location of final image as shown in Fig.34-13. Take the refractive index of the slab as 4/3.
View SolutionSparkle in the diamond
The purpose of a diamond is, of course, to sparkle . Part of the art of cutting a diamond is to ensure that all the light entering through the top face or side facets leaves through those surfaces, to participate in the sparkle. Figure 34-18 shows part of a cross-sectional slice through a brilliant-cut diamond, with a ray entering at point A on the top face. In this type of cut, the top and bottom surfaces have normal lines that intersect at the indicated `48.84^(@)`. At point B, at least part of the light reflects and leaves the diamond properly , but part could refract and thus leak out of the diamond. Consider a light ray incident at angle `theta_(1)=40^(@)` at A. Does light leak at B if air `(n_(4)=1.00)` lies next to the bottom surface ? Does light leak if greasy grime `(n_(4)=1.63)` coats the surface? The index of refraction of diamond is `n_(din)=2.419`.
View SolutionTotal internal reflection of light ray from cylindrical optical fiber
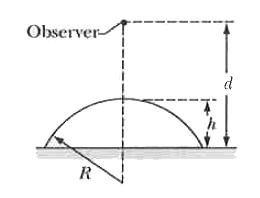
An optical fiber consists of a cylindrical core of unknown refractive index kept in air. A ray of light is incident on the flat face of the core so that it gets totally reflected at the curved surface (Fig.34-19). What is the possible refractive index if the ray is totally reflected at any angle of incidence `phi` from air ?
View SolutionA point source of light is placed `4m` below the surface of a liquid of refractive index `5//3`. The minimum diameter of a disc, which should be placed over the source, on the surface of the liquid to cut off all light out of water, is
View SolutionA ray of light travelling in air is incident at grazing angle (incident angle=`90^(@))` on a long rectangular slab of a transparent medium of thickness `t=1.0` (see figure). The point of incidence is the origin `A(O,O)` .The medium has a variable index of refraction n(y) given by :`n(y)=[ky^(3//2)+1]^(1//2)` ,where k=`1.0m^(-3//2)`.the refractive index of air is 1.0`

(i) Obtain a relation between the slope of the trajectory of the ray at a point `B(x,y)`in the medium and the incident angle at that point
(ii) obtain an equation for the trajectory `y(x)`of the ray in the medium.
(ii) Determine the coordinates (`x_(1),y_(1))` of the point `P`.where the ray the ray intersects upper surface of the slab -air boundary.
Indicate the path of the ray subsequently.
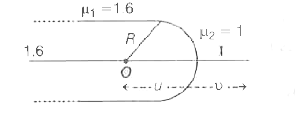
View SolutionA point object O is placed on the axis of a cylindrical piece of glass of refractive index 1.6 as shown in the figure. One surface of the glass piece is convex with radius of curvature 3mm. The point appeared to be at 5 mm on the axis when viewed along the axis and from right side of convex surface. The distance of the point object from the convex surface is :
View SolutionImage of the fish in a spherical aquarium
Assume that a boy views a fish in a spherical aquarium of radius `10cm` kept at a distance of `2cm` from the center toward the left of center. The boy is viewing from the left (Fig.34-27). Where will the image of fish be formed?
View SolutionRefraction through eye of Anableps anabelps fish
Figure 34-28 shows a vertical cross section through an eye of the Anableps anableps fish that swims with each eye half in and half out of the water, with a pigment band separating the two halves at the water surface. The front of the eye (the cornea) is a spherically convex refracting surface of radius `r=1.95mm` and index of refraction `n_(2)=1.335`. The refraction at the cornea is the first step in the eye's focusing of a real image into the back of the eye (the retina), where visual processing begins. If the cornea faces an insect (lunch) at object distance `p=0.20m`, what is the image distance i of that refraction for the cornea in air `(n_(1)=1.000)` and in water `(n_(1)=1.333)` ?
View SolutionImage of an object kept on the principal axis of transparent sphere with silvered rear surface
A sphere of radius 1m and n=1.5 is silvered at its back. A point objet is kept at a distance of 1m from the front face (Fig. 34-30). Where will the final image be formed ?
View SolutionFocal length of concavo-convex lens
A concavo-convex lens has radii of its faces 20cm and 60cm. If the refractive index of the material of the lens is `1.5`, find its focal length.
View SolutionDeviation of a ray incident on a converging lens
Find the point at which the first ray crosses the principal axis after refraction (Fig. 34-39). Also find its deviation.
View SolutionFormation of image by a combination of converging and a diverging lens
Find the location of the final image after all the refrections in Fig. 34-41. The radius of both the plano-convex lens and plano-concave lens is 10cm and refrective index is 1.5.
View SolutionCondition for autocollimation
Find the distance between the convex lens and convex mirror, both of focal length 20cm (Figs. 34-42a and b ), so that for an object kept at 30cm from the lens, the final image is at the object itself. (This is commonly known as condition for autocollimation that we discussed in Section 33.5)
View SolutionFind the radius of the light spot on the screen as shown in Fig. 34-44. Consider only the light rays refracted from the lens. Find the average intensity on the screen. The source is point source of power `100W`. The lens has an aperture of `6cm`.
View SolutionBeam expander
Assume that a parallel beam is incident on two convex lens of focal length 20cm and 40cm. The beam has a diameter of 2cm. What should be the distance between them so that the final beam emerges parallel to the principal axis( Fig. 34-46) ?
View SolutionFinding focal length by displacement method
In an experiment for finding focal length of a convex lens the object and screen are kept fixed. There are two position of lens between the object and screen for which an image is obtained on the screen. These positions are separated by 20cm. Also, the magnification in first situation is -2. Find the distance between the object and the screen, that is the focal length of the lens.
View SolutionFinding equivalent focal length
Two equiconcave lens of focal length -20cm each having a refractive index of 1.50 are kept together and the space between them is filled with water as shown in Fig. 34-49. Find the equivalent focal length of the arrangement thus formed.
View SolutionA convex lens of radii of curvature 20cm and 30 cm respectively. It is silvered at the surface which has smaller radius of curvature. Then it will behave as `(mu_(g) = 1.5)`
View SolutionRefractive index of a prism
A ray is incident on an equilateral prism such that the angle of deviation is `30^(@)` . It is seen that if the angle of incidence is increased by `30^(@)`, then the deviation is again `30^(@)`. Find the refractive index of the prism.
View SolutionMinimum and maximum deviation in a prism
A `60^(@)` prism has a refractive index of `1.5`.
(a) Calculate the angle of incidence for minimum deviation.
View SolutionTotal internal reflection in a prism
What is the relationship between A and n so, that no rays come out of second face ?
View SolutionImage formation by a system of thin prism and lens
A beam parallel to the principal axis is incident on a thin prism (Fig. 34-60). Find the location of final image.
View SolutionDeviation without dispersion and disperison without deviation
What should be the condition on a combination of prism for the following ?
(a) Angle of dispersion is zero but angle of deviation is not zero.
View Solution
Sample problem questions and complete solutions for chapter GEOMETRICAL OPTICS : REFRACTION of RESNICK AND HALLIDAY PHYSICS (HINGLISH) of Class 12