Complete Solutions to NCERT SECTION ( EXERCISE 9.4) of chapter AREAS OF PARALLELOGRAMS AND TRIANGLES of Class 9 book with complete answers and questions

Parallelogram ABCD and rectangle ABEF are on the same base AB and have equal areas. Show that the perimeter of the parallelogram is greater than that of the rectangle.

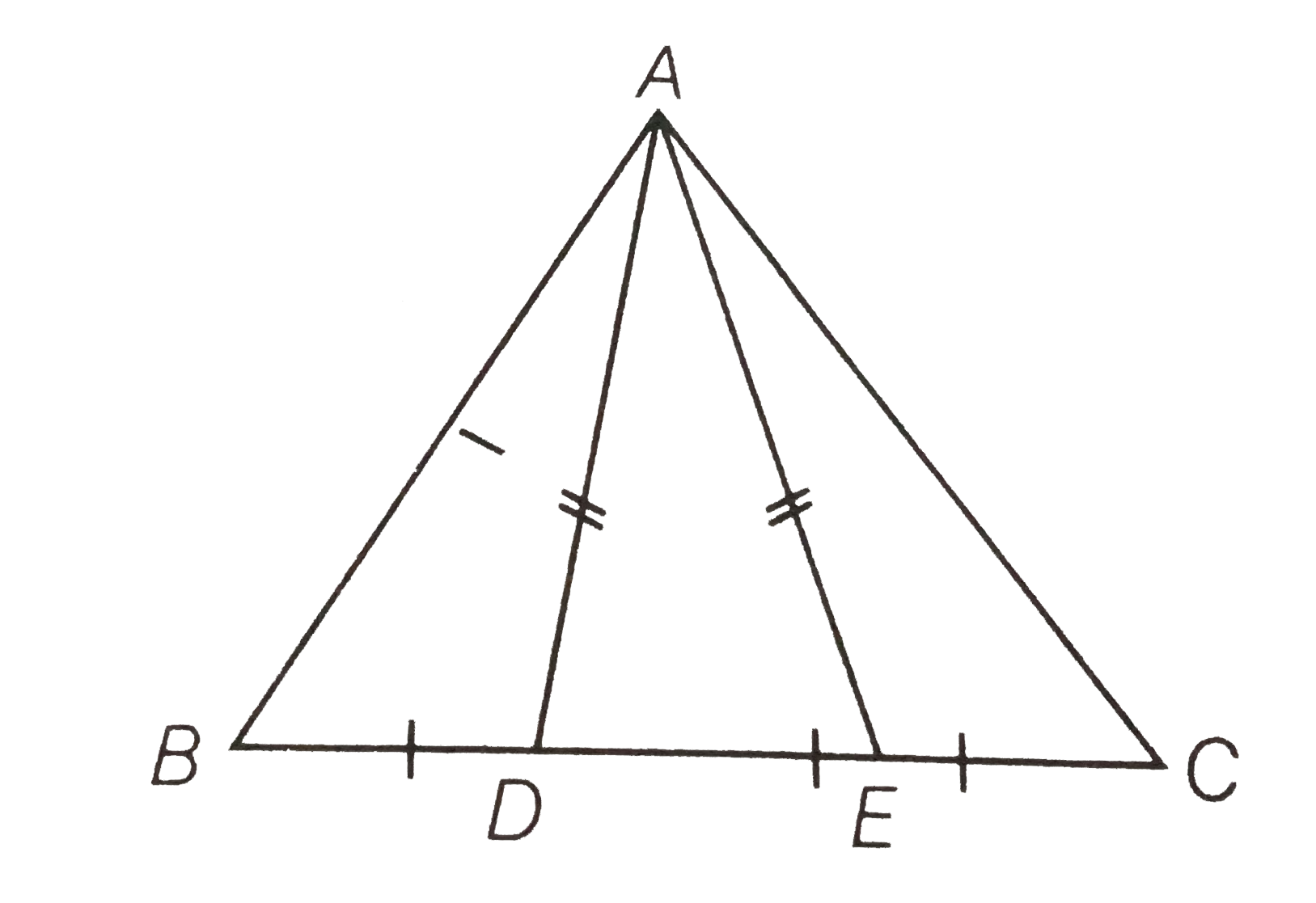
In figure ,D and E are Points on side BC of a `Delta ABC` such that BD=CE and AD=AE.Show that `Delta ABD cong Delta ACE.`

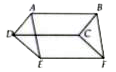
In the figure, ABCD, DCFE and ABFE are parallelograms. Show that ar(`Delta`ADE) = ar(`Delta`BCF).

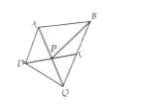
In the figure, ABCD is a parallelogram and BC is produced to a point Q such that AD = CQ. If AQ intersect DC at P, show that ar(`Delta`BPC) = ar(`Delta`DPQ).

In figure, ABC and BDE are two equilateral triangles such that D is the mid-point of BC. If AE intersects BC at F, show that

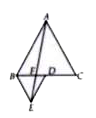
ar(`Delta`BDE) = 1/4 ar(`Delta`ABC)

In figure, ABC and BDE are two equilateral triangles such that D is the mid-point of BC. If AE intersects BC at F, show that

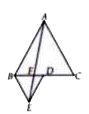
ar(`Delta`BDE) = 1/2 ar(`Delta`BAE)

In figure, ABC and BDE are two equilateral triangles such that D is the mid-point of BC. If AE intersects BC at F, show that

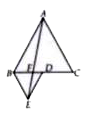
ar(`Delta`ABC) = 2 ar(`Delta`BEC)

In figure, ABC and BDE are two equilateral triangles such that D is the mid-point of BC. If AE intersects BC at F, show that

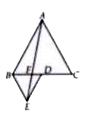
ar(`Delta`BFE) = 2 ar(`Delta`FED)

Diagonals AC and BD of `square ABCD` intersect each other at point P. Show that `ar(triangle APB)xxar(triangle CPD)=ar(triangle APD)xxar(triangle BPC)`

P and Q are respectively the mid-points of sides AB and BC of a triangle ABC and R is the mid-point of AP, show that
ar(`Delta`PRQ) = 1/2 ar(`Delta`ARC)

In `Delta ABC`, P is a point on BC such that `BP:PC=4:5` and Q is the mid - point of BP. Then area `(Delta ABQ)` : area `(Delta ABC)` is equal to :

ABC and BDE are two equilateral triangles such that D is the mid-point of BC. Then, `ar (DeltaBDE) = (1)/(2) ar (DeltaABC)`.

ABC is a right triangle right angled at A. BCED, ACFG and ABMN aresquares on the sides BC, CA and AB respectively. Line segment `A X_|_D E`meets BCat Y. Show that:(i) `DeltaM B C~=DeltaA B D`(ii) `a r\ (B Y X D)\ =\ 2\

ABC is a right triangle right angled at A. BCED, ACFG and ABMN aresquares on the sides BC, CA and AB respectively. Line segment `A X_|_D E`meets BCat Y. Show that:(i) `DeltaM B C~=DeltaA B D`(ii) `a r\ (B Y X D)\ =\ 2\

ABC is a right triangle right angled at A. BCED, ACFG and ABMN aresquares on the sides BC, CA and AB respectively. Line segment `A X_|_D E`meets BCat Y. Show that:(i) `DeltaM B C~=DeltaA B D`(ii) `a r\ (B Y X D)\ =\ 2\

ABC is a right triangle right angled at A. BCED, ACFG and ABMN aresquares on the sides BC, CA and AB respectively. Line segment `A X_|_D E`meets BCat Y. Show that:(i) `DeltaM B C~=DeltaA B D`(ii) `a r\ (B Y X D)\ =\ 2\

In Figure, `A B C` is a right triangle right angled at `A ,\ B C E D ,\ A C F G\ a n d\ A M N` are square on the sides `B C ,\ C A\ a n d\ A B` respectively. Line segment `A X\ _|_D E` meets `B C` at `Ydot` Show that: `a r\ (C Y X E)=2a r(\ F C B)`

In Figure, `A B C` is a right triangle right angled at `A ,\ B C E D ,\ A C F G\ a n d\ A M N` are square on the sides `B C ,\ C A\ a n d\ A B` respectively. Line segment `A X\ _|_D E` meets `B C` at `Ydot` Show that: `a r\ (B Y X D)=a r\ (A B M N)`

In Figure, `A B C` is a right triangle right angled at `A ,\ B C E D ,\ A C F G\ a n d\ A M N` are square on the sides `B C ,\ C A\ a n d\ A B` respectively. Line segment `A X\ _|_D E` meets `B C` at `Ydot` Show that: `\ F C B~=\ A C E`