Complete Solutions to EXERCISE ( Subjective Problems ) (Short Answer Type ) of chapter AREAS OF PARALLELOGRAMS AND TRIANGLES of Class 9 book with complete answers and questions
| Class 9 | MATHS |
| Chapter | AREAS OF PARALLELOGRAMS AND TRIANGLES |
| MTG IIT JEE FOUNDATION | EXERCISE ( Subjective Problems ) (Short Answer Type ) |
| Number Of Questions | 4 |
| Academic Year | 2023-24 |
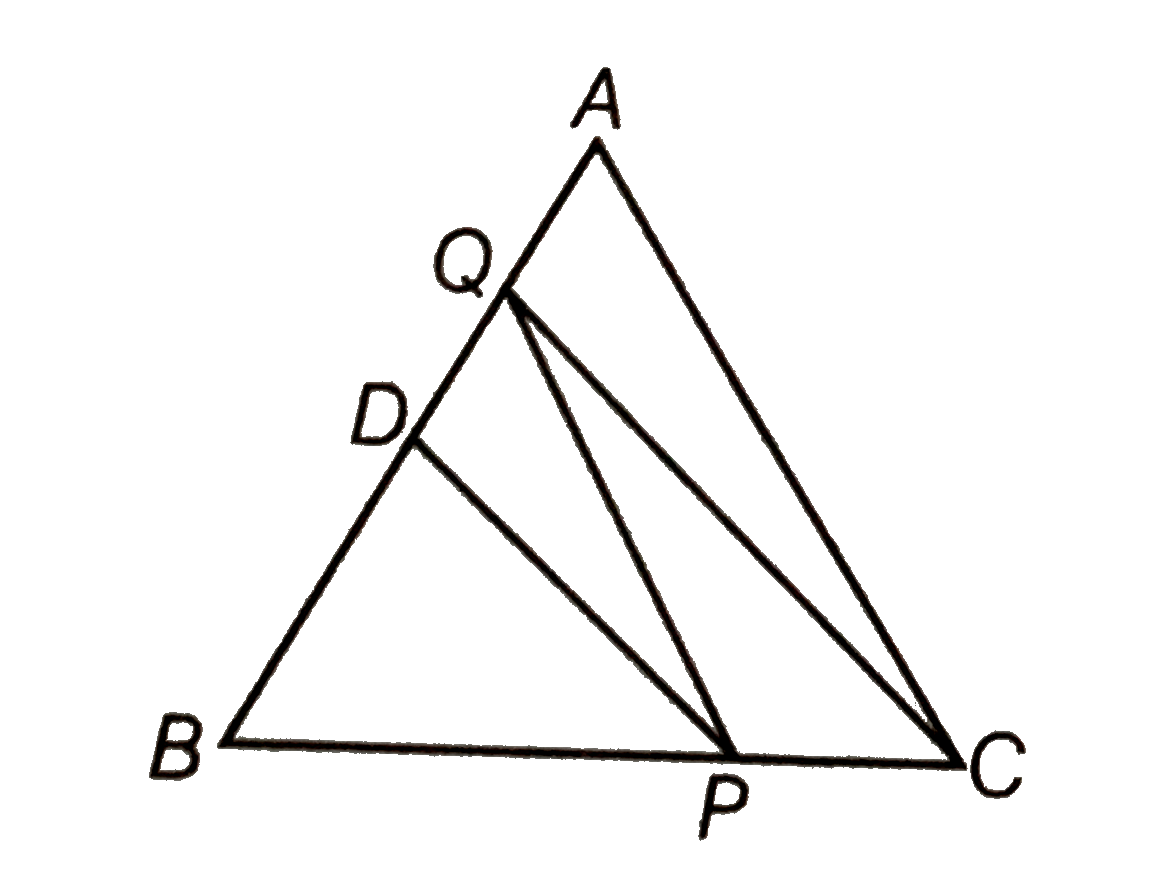
In `Delta` ABC, D is the mid-point of AB and P is any point on BC. If `CQ || PD` meets AB and Q (shown in figure), then prove that
`ar (DeltaBPQ) = (1)/(2) ar (DeltaABC)`.
View SolutionABCD is a trapezium in which AB I I DC. DC is produced to E such tha t CE= AB, prove that ar(`Delta`ABD) = ar(`Delta`BCE).
View SolutionProve that the area of an equilateral triangle is equal to `sqrt3/4 a^(2)`, where a is the side of the triangle.
View SolutionIn the given figure, ABCD is a parallelogram. E and F are any two points on AB and BC, respectively. Prove that `ar (triangleADF)=ar(triangleDCE)`.
View Solution
EXERCISE ( Subjective Problems ) (Short Answer Type ) questions and complete solutions for chapter AREAS OF PARALLELOGRAMS AND TRIANGLES of MTG IIT JEE FOUNDATION of Class 9
All Exercises from MTG IIT JEE FOUNDATION Class 9 MATHS AREAS OF PARALLELOGRAMS AND TRIANGLES
- EXERCISE ( Subjective Problems ) (Long Answer Type )
- EXERCISE ( Subjective Problems ) (Short Answer Type )
- EXERCISE ( Subjective Problems ) (Very Short Answer Type )
- EXERCISE ( Comprehension Type )
- EXERCISE ( Integer/Numerical Value Type )
- EXERCISE ( Multiple Choice Questions )
- EXERCISE (Assertion & Reason Type )
- EXERCISE (Match the Following )
- ILLUSTRATIONS
- NCERT SECTION ( EXERCISE 9.1)
- NCERT SECTION ( EXERCISE 9.2)
- NCERT SECTION ( EXERCISE 9.3)
- NCERT SECTION ( EXERCISE 9.4)
- Olympiad/HOTS Corner
- SOLVED EXAMPLES