Complete Solutions to Exercise 10.2 of chapter CIRCLES of Class 9 book with complete answers and questions
| Class 9 | MATHS |
| Chapter | CIRCLES |
| NCERT EXEMPLAR | Exercise 10.2 |
| Number Of Questions | 10 |
| Academic Year | 2023-24 |
Two chords AB and CD of a circle are each at distances 4 cm from the centre. Then,
View SolutionTwo chords AB and AC of a circle with centre O are on the opposite sides of OA. Then, `angleOAB=angleOAC`.
View SolutionThe congruent circles with centres Oand O' intersect at two points A and B. Then, `angleAOB=angleAO'B`.
View SolutionThrough three collinear points a circle can be draw.
View SolutionA circle of radius 3 cm can be drawn through two points A, B such that AB=6 cm. State true or false
View SolutionIf AOB is a diameter of a circle and C is a point on the circle, then `AC^(2)+BC^(2)=AB^(2)`.
View SolutionABCD is a cyclic quadrilateral such that `angleA=90^(@),angleB=70^(@),angleC=95^(@) and angleD=105^(@)`.
View SolutionIf A, B, C and D are four points such that `angleBAC=30^(@)and angleBDC=60^(@)`,then D is the centre of the circle through A, B and C.
View SolutionIf A, B, C and D are four points such that `angleBAC=45^(@) and angleBDC=45^(@)`, then A, B, C and D are concyclic.
View SolutionIn figure, if AOB is a diameter and `angleADC=120^(@),"then"angleCAB=30^(@)`.
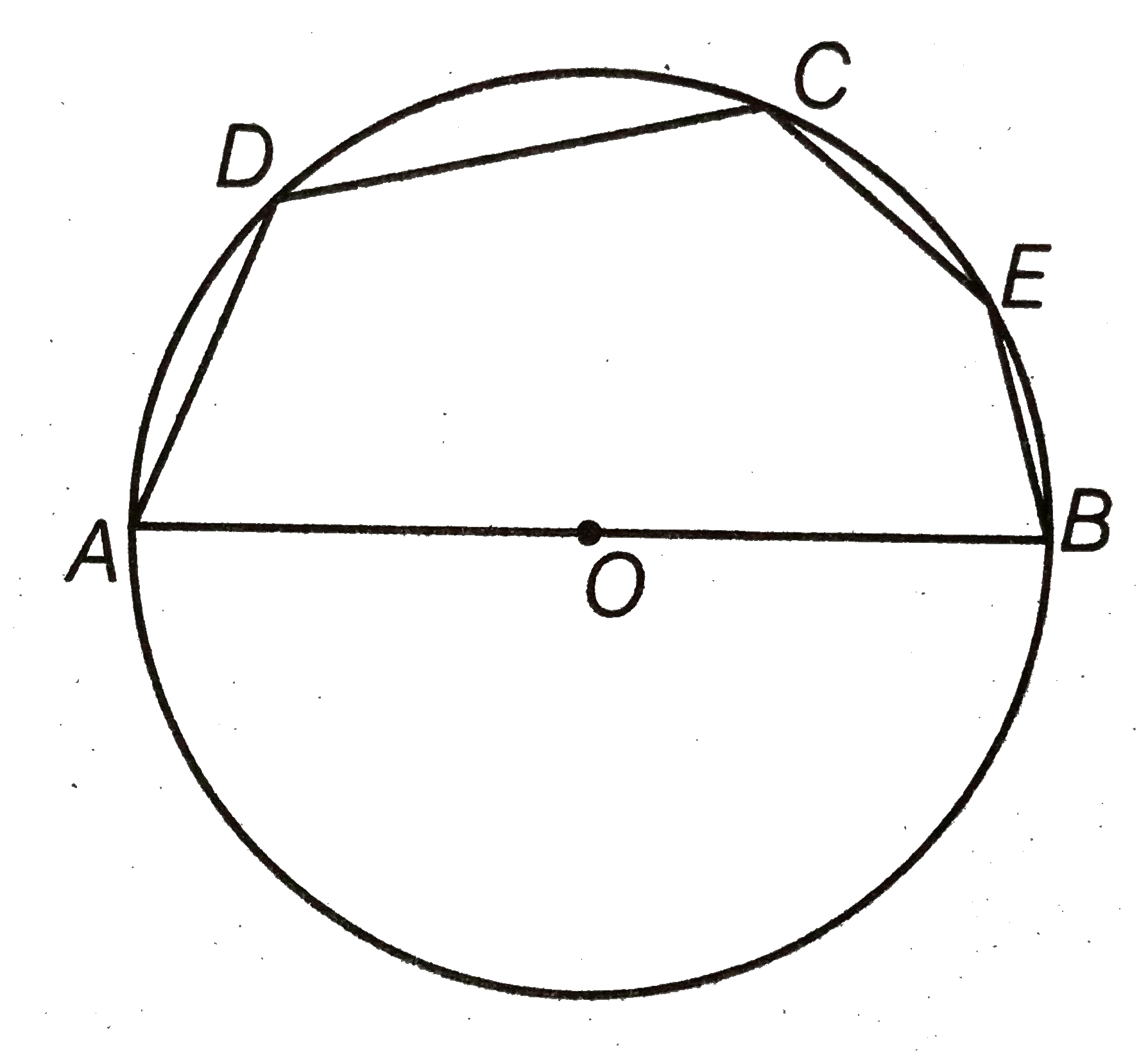
View Solution
Exercise 10.2 questions and complete solutions for chapter CIRCLES of NCERT MATHS (ENGLISH) of Class 9