In an experiment brass and steel wires of length 1 m each with areas of cross section 1mm2 are used. The wires are connected in series and one end of the combined wire is connected to a rigid support and other end is subjected to elongation. The stress required to produce a net elongation of 0.2 mm is [Given the Youngs Modulus for steel and brass are respectively 120 × 109 N/m2 and 60 × 109 N/m2 ]1 4.0 × 106 N/m2 2 1.2 × 106 N/m2 3 1.8 × 106 N/m24 8 ×106
Correct option 4 8 ×106Explanation :
Related Solutions
In an experiment, brass and steel wires of length 1 m each with areas of cross section 1mm^(2) are used. The wires are connected in series and one end of the combined wire is connected to a rigid support and other end is subjected to elongation. The stress required to produce a net elongation of 0.2 mm is, [Given, the Young’s Modulus for steel and brass are, respectively, 120 xx 10^(9) N//m^(2) and 60 xx 10^(9) N//m^(2)
View SolutionIn an experiment, brass and steel wires of length 1 m each with areas of cross section are used. The wires are connected in series and one end of the combined wire is connected to a rigid support and other end is subjected to elongation. The stress required to produce a net elongation of 0.2 mm is, [Given, the Young’s Modulus for steel and brass are, respectively,
View SolutionIn an experiment, brass and steel wires of length 1 m each with areas of cross section 1mm^(2) are used. The wires are connected in series and one end of the combined wire is connected to a rigid support and other end is subjected to elongation. The stress required to produce a net elongation of 0.2 mm is, [Given, the Young’s Modulus for steel and brass are, respectively, 120 xx 10^(9) N//m^(2) and 60 xx 10^(9) N//m^(2)
View SolutionIn an environment, brass and steel wires of lengh 1 m each with areas of cross section are used. The wires are connected in series ankd one end of the combined wie is connected toa rigid support and other endis subjected to elongation. The stress (in ) requird to produce a net elongation of 0.2 mm is
[Given the Young's modulus for steel and brass are respectively and )View SolutionTwo wires of diameter 0.25 cm, one made of steel and the other made of brass are loaded as shown in fig. The unloaded length of steel wire is and that of brass wire is 1.0m. Compute the elongations of the steel and the brass wires.
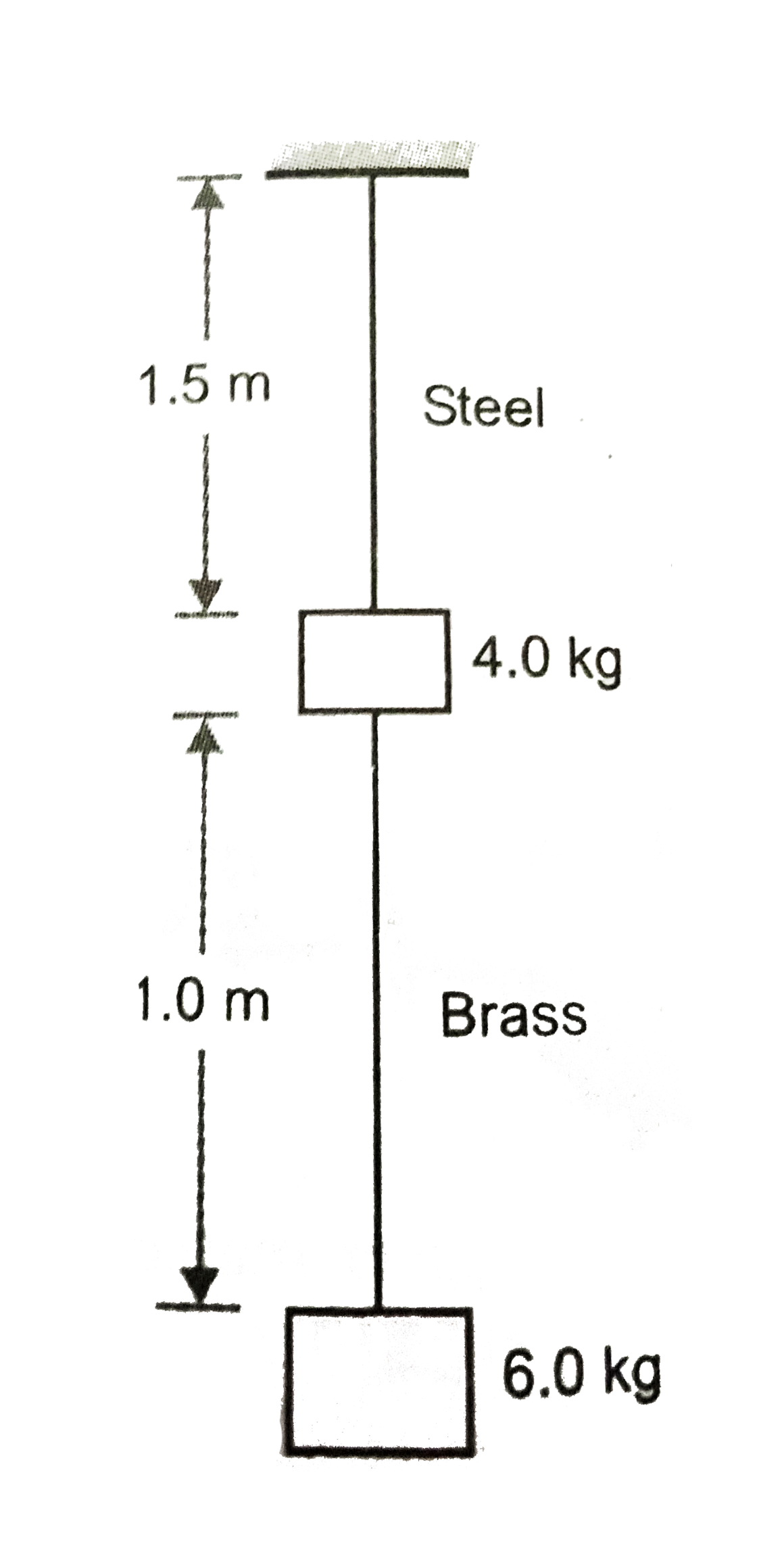 View Solution
View Solutionएक प्रयोग में पीतल तथा स्टील के दो तारो का प्रयोग किया गया है, जिनमे प्रत्येक की लम्बाई 1 m तथा अनुप्रस्थ-काट का क्षेत्रफल है। इन तारो को श्रेणीक्रम में जोड़ते है तथा संयुक्त तार के एक सिरे को दृढ़ स्तम्भ से जोड़ते है एवं दूसरे सिरे को खींचा जाता है। 0.2 mm की कुल वृद्धि केलिय प्रतिबल का मान होगा (दिया है, स्टील तथा पीतल के यंग प्रत्यास्थता गुणांक, तथा )
View SolutionThe Young's Modulus of steel is twice that of brass. Two wires of same length and of same area of cross-section, one of steel and another of brass are suspended from the same roof. If then the weights added to the steel and brass wires must be in the ratio of
View SolutionAn aluminium wire and a steel wire of the same length and cross section are joined end to end. The composite wire is hung from a rigid support and the load is suspended at the free end. If Y_(AL) = 7xx10^(10) N//m^(2) and Y_("steel") = 7xx10^(11) N//m^(2) , the ratio of elongation of aluminium and steel wires is
View SolutionA copper wire of length 2.2 m and a steel wire of length 1.6 m,both of diameter 3.0 mm, are connected end to end. When stretched by a load, the net elongation is found to be 0.70 mm. obtain the load applied.
View SolutionA copper wire of length 2.2 m and a steel wire of length 1.6 m, both of diameter 3.0 mm, are connected end to end. When stretched by a load , the net elongation is found to be 0.70 mm. Obtain the load applied.
View SolutionA copper wire of length 2.2 m and a steel wire of length 1.6 m, both of diameter 3.0 mm, are connected end to end. When stretched by a load , the net elongation is found to be mm. Obtain the load applied.
View SolutionA copper wire of length 2.2 m and a steel wire of length 1.6 m, both of diameter 3.0 mm, are connected end to end. When stretched by a load , the net elongation is found to be mm. Obtain the load applied.
View SolutionA copper wire of length 2.2 m and a steel wire of length 1.6 m, both of diameter 3.0 mm, are connected end to end. When stretched by a load , the net elongation is found to be mm. Obtain the load applied.
View SolutionThe ratios of length areas of cross-section and Young's modulii of steel of that of brass wires shown in the figure are a,b and c respectively . The ratio of increase in the lengths of brass of that of steel wires is [Assume that the masses of steel and brass wires are negligible]
View SolutionThe Young's modulus of steel is twice that of brass. Two wires of the same length and of the same area of cross section, one of steel and another of brass are suspended from the same roof. If we want the lower ends of the wires to be at the same level, then the weight added to the steel and brass wires must be in the ratio of
View SolutionThe young's modulus of steel is twice that of brass . Two wires of same length and of same area of cross section, one of steel and another of brass are suspended from the same roof .If we want the lower ends of the wires to be at the same level, then the weigths added to the steel and brass wires must be in the ratio of :
View SolutionThe Young's modulus of steel is twice that of brass. Two wires of the same length and of the same area of cross section, one of steel and another of brass are suspended from the same roof. If we want the lower ends of the wires to be at the same level, then the weight added to the steel and brass wires must be in the ratio of
View SolutionThe Young's modulus of steel is twice that of brass. Two wires of same length and of same area of cross section, one of steel and another of brass are suspended from the same roof. If we want the lower ends of the wires to be at the same level, then the weights added to the steel and brass wires must be in the ratio of:
View SolutionTwo Young's modulus of steel! is twice that of brass. Two wires of same length and of same area of cross section, one of steel and another of brass are suspended from the same roof. If we want the lower ends of the wires to be at the same level, then the weights added to the steel and brass wires must be in the ratio of :
View SolutionThe Young's modulus of steel is twice that of brass. Two wires of the same length and of the same area of cross section, one of steel and another of brass are suspended from the same roof. If we want the lower ends of the wires to be at the same level, then the weight added to the steel and brass wires must be in the ratio of
View Solution
