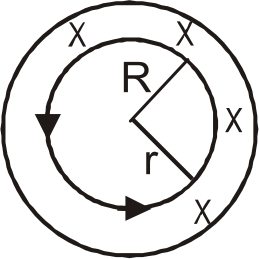
Time varying magnetic filed is present in a circular region of radius R. Match the proper entries from column-2 to column-1 using the codes given below the columns,
Column IColumn II(P) An unsteady magnetic field(1) Electric field is perpendicular to the length of rod (Q)Induced electric field at a point within magnetic field (r gtR)(2)r2dBdt(R)Induced electric field at a point out side the magnetic field (r lt R)(3)R22rdBdt(S)If a rod is placed along the diameter of the magnetic field(4) induced electric field
PQRS(A)3214
PQRS(B)2134
PQRS(C)4213
PQRS(D)4231
Explanation :
time varying magnetic field produced electric field unknown as induced electric field.
So (P)→(4)
for flt R
∮E.DI=−AdBdt
E2πr=−πr2dBdt
E=−r2dBdt
So, (Q)→(2)
For rgt R
E2pr=−πR2dBdtss,E=−R22rdBdt
So (R)→(3)
If rod ris placed along the diameter of magnetic field, then electric field is perpendicular to length of rod.
Topper's Solved these Questions
Similar Questions
A radioactive element decays by β−emission. A detector records n beta particles in 2s and in next 2s it records 0.75n beta particles. Find mean life correct to nearest whole number. Given ln |2|=0.6931, ln |3|=1.0986.
04:24View SolutionWhen p−n junction diode is forward biased then
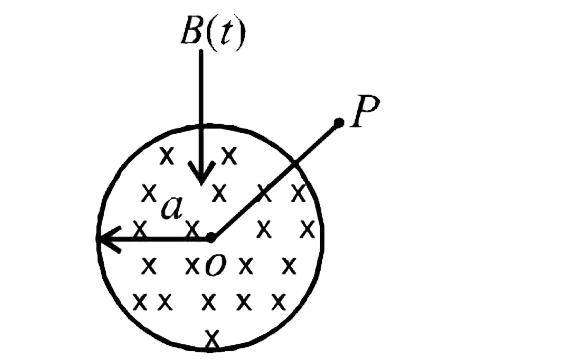
02:41View SolutionA uniform but time-varying magnetic field B(t) exists in a circular region of radius a and is directed into the plane of the paper, as shown. The magnitude of the induced electric field at point P at a distance r from the centre of the circular region
01:38View SolutionA uniform but time varying magnetic field B(t) exist in a circular region of radius a and is directed into the plane of the paper as shown. The magnitude of the induced electric field at point P at a distance r form the centre of the circular region.
02:35View SolutionA time varying uniform magnetic field passes through a circular region of radius R. The magnetic field is directed outwards and it is a function of radial distance 'r' and time 't' according to relation B-B_(0)rt. The induced electric field strength at a radial distance R//2 from the centre will be.
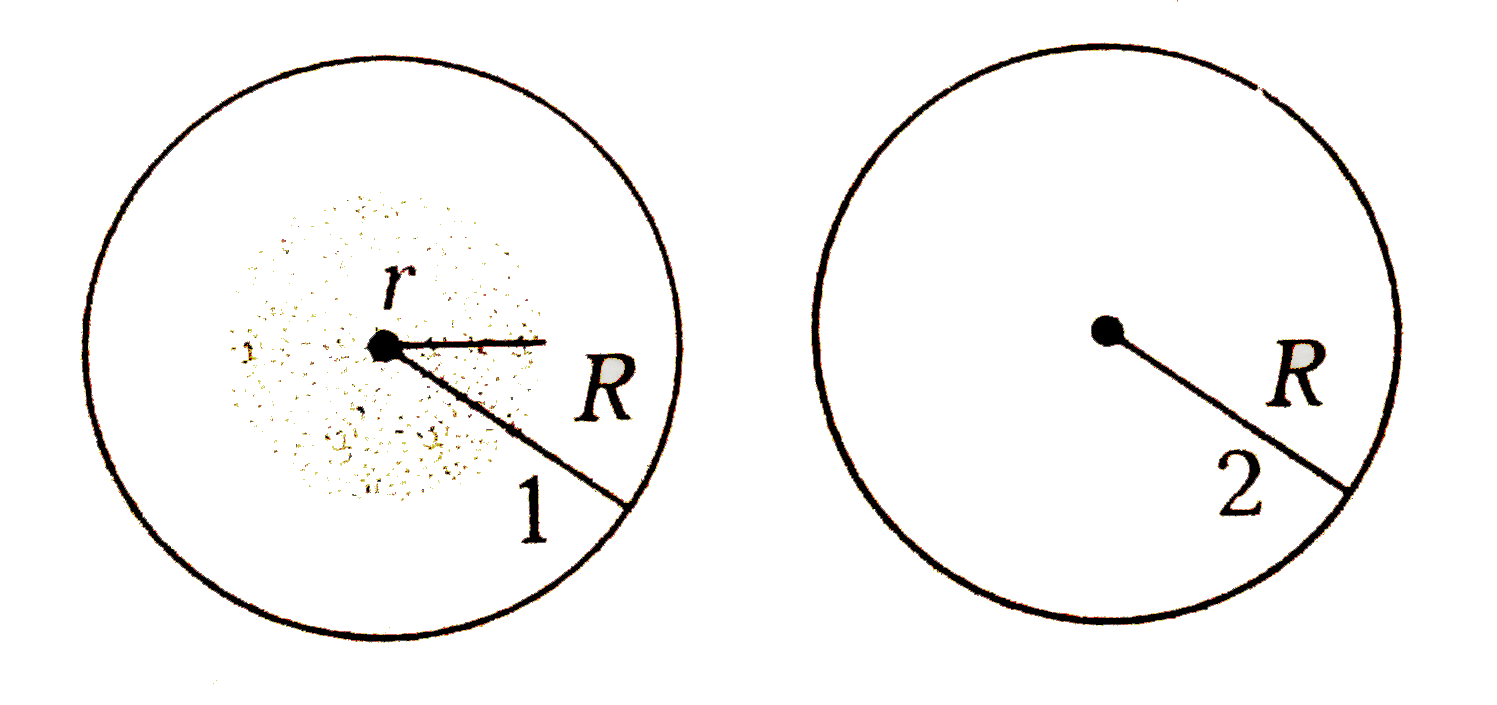
03:17View SolutionA uniform magnetic field is restricted within a region of radius r. The magnetic field changes with time at a rate dBdt. Loop 1 of radius R > r encloses the region r and loop 2 of radius R is outside the region of magnetic field as shown in figure. Then, the emf generated is
 05:09View Solution
05:09View SolutionA uniform but time-varying magnetic field B (t) exists in a circular region of radius a and is directed into the plane of the paper as shown . The magnitude of the induced electric field at point P at a distance r from the centre of the circular region is
03:23View SolutionA uniform magnetic field is restricted within a region of radius r. The magnetic field changes with time at a rate d→Bdt. Loop 1 of radius R > r encloses the region r and loop 2 of radius R is outside the region of magnetic field as shown in the figure below. Then the emf generated is
 View Solution
View Solution(A ): Induced electric fields are produced by time varying magnetic field. (R): According to faraday’s law ointvecE.vecdl=(dϕ)/(dt)
View SolutionA uniform but time varying magnetic field B(t) exists in a circular region of radius a and is directed into the plane of paper as shown. The magnitude of the induced electric field at point P at a distance r from the centre of the circular region.
02:51View SolutionA uniform but time-varying magnetic field B(t) exists in a circular region of radius a and is directed into the plane of the paper, as shown in the figure. The magnitude of the induced electric field at point P at a distance r from the centre of the circular region
02:44View SolutionA uniform but time varying magnetic field B(t) exist in a circular region of radius a and is directed into the plane of the paper as shown. The magnitude of the induced electric field at point P at a distance r form the centre of the circular region.
 05:25View Solution
05:25View SolutionA uniform but time-varying magnetic field B(t) exists in a circular region of radius a and is directed into the plane of the paper, as shown. The magnitude of the induced electric field at point P at a distance r from the centre of the circular region
 05:51View Solution
05:51View Solutionप्रेरण B का एकसमान चुंबकीय क्षेत्र त्रिज्या R के एक बेलनाकार क्षेत्र मे सीमित है। यदि चुंबकीय क्षेत्र, dBdt=αTs−1 की नियत दर से बढ़ रहा है, तो अक्ष से r दूरी पर स्थित एक बिंदु P पर प्रेरित विद्युत क्षेत्र की तीव्रता, जैसा कि चित्र में दर्शाया गया है, बराबर है:
 03:18View Solution
03:18View SolutionFigure shows a circular region of radius R in which uniform magnetic field B exists. The magnetic field is increasing at a rate (dB)/(dt) . The induced electric field at a distance r from the centre for r lt R is
02:35View Solution

