Calculate the moment of inertia
(a) of a copper uniform disc relative to the symmetry axis perpendicular to the plane of the disc, if its thickness is equal to b=2.0mm and its radius to R=100mm,
(b) of a uniform solid cone relative to its symmetry axis, if the mass of the cone is equal to m and the radius of its base to R.
(a) Consider an elementary disc of thickness dx. Moment of inertia of this element about the z-axis, passing through its C.M.
dIz=(dm)R22=ρSdxR22
where ρ=density of the material of the plate and S=area of cross section of the plate.
Thus the sought moment of inertia
Iz=ρSR2b∫0dx=R22ρSb
=π2ρbR4 (as S=πR2)

putting all the values we get, Iz=2⋅gm⋅m2
(b) Consider an element disc of radius r and thickness dx at a distance x from the point O. Then r=xtanα and volume of the disc.
=πr2tan2αdx
Hence, its mass dm=πx2tanαdx⋅ρ (where ρ=density of the cone =m/13πR2h)
Moment of inertia of this element, about the axis OA,
dI=dmr22
=(πx2tan2αdx)x2tanα2
=πρ2x4tan4αdx

Thus the sought moment of inertia I=πρ2tan4αh∫0x4dx
=πρR4⋅h410h4 (as tanα=Rh)
Hence I=3mR210 (putting ρ=3mπR2h)

Topper's Solved these Questions
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Elastic Deformation Of Asolid Body25videos
PHYSICAL FUNDAMENTALS OF MECHANICS
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Hydrodynamics25videos
PHYSICAL FUNDAMENTALS OF MECHANICS
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Universal Gravitation34videos
PHYSICAL FUNDAMENTALS OF MECHANICS
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Electromagnetic Waves, Radiation36videos
OSCILLATIONS AND WAVES
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - THERMODYNAMICS AND MOLECULAR PHYSICSEXERCISE - Transport Phenomena38videos
THERMODYNAMICS AND MOLECULAR PHYSICS
Similar Questions
Calculate the moment of inertia of a disc of radius R and mass M, about an axis passing through its centre and perpendicular to the plane.
View SolutionFind the moment of inertia of a uniform half-disc about an axis perpendicular to the plane and passing through its centre of mass. Mass of this disc is M and radius is R.
View Solution
Knowledge Check
- Question 1 - Select One
The moment of inertia of a uniform semicircular disc of mass M and radius r about a line perpendicular to the plane of the disc through the center is
A12Mr2BMt2C25Mr2D14Mr2 - Question 2 - Select One
The moment of inertia of a copper disc, rotating about an axis passing through its centre and perpendicular to its plane
Aincreases if its temperature is increasedBchanges if its axis of rotation is changedCincreases if its angular velocity is increasedDboth (a) and (b) are correct - Question 3 - Select One
A uniform semicircular disc of mass 'm' and radius 'R' is shown in the figure. Find out its moment of inertia about.
(a) axis 'AB'( shown in the figure 0 which passes through geometrical centre and lies in the plane of the disc
(b) axis 'CD' which passes through its centre of mass and it is perpendicular to the plane of the disc.
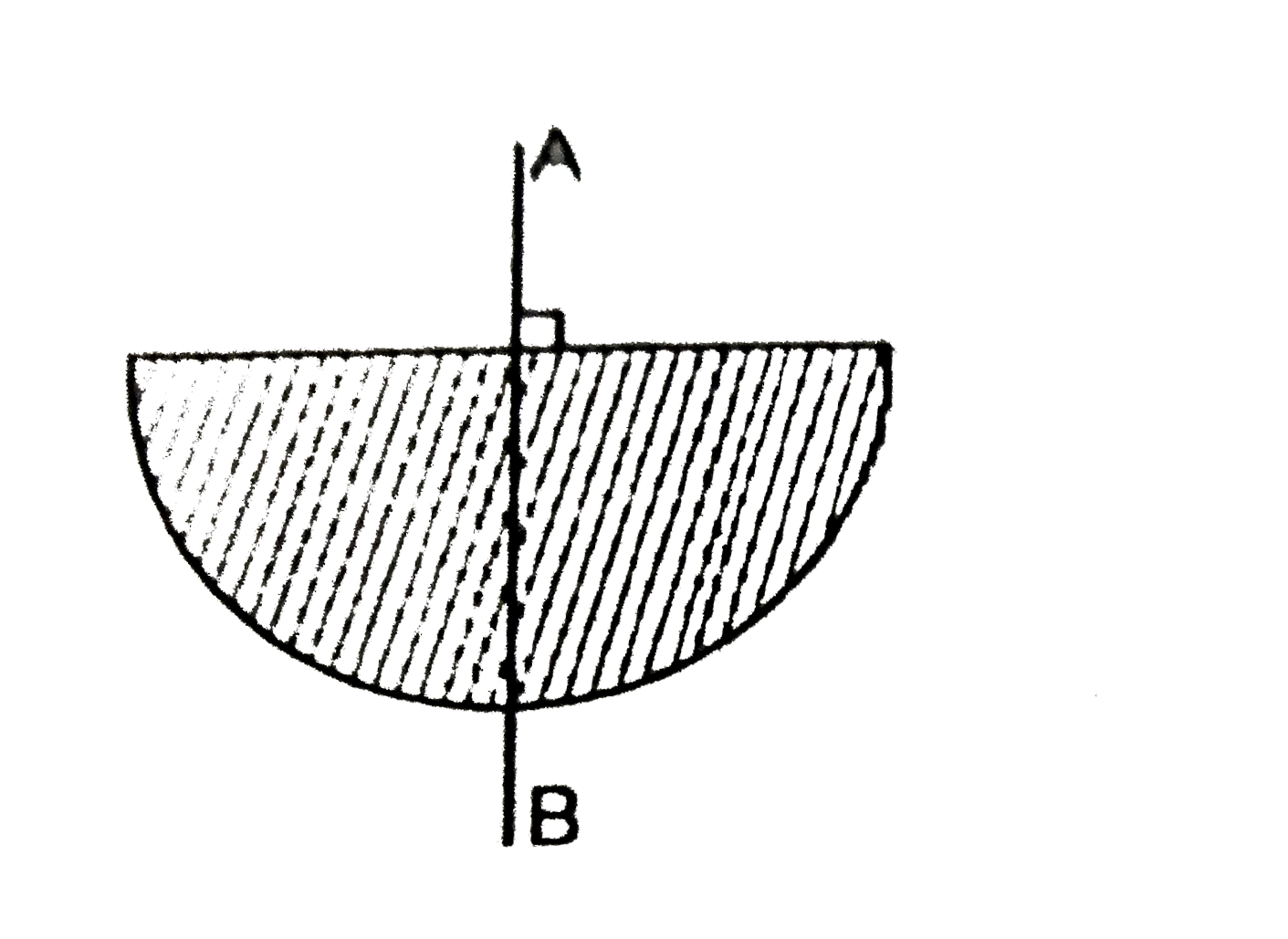 AMR22−M(2Rπ)2BMR22−M(4Rπ)2CMR22+M(4R3π)2DMR22+M(2Rπ)2
AMR22−M(2Rπ)2BMR22−M(4Rπ)2CMR22+M(4R3π)2DMR22+M(2Rπ)2
Find the moment of inertia of a uniform cylinder about an axis through its centre of mass and perpendicular to its base. Mass of the cylinder is M and radius is R.
View SolutionCalculate the moment of inertia of uniform circular disc of mass 500 g, radius 10 cm about : the axis tangent to the disc and parallel to its diameter
View SolutionThe moment of inertia of a uniform circular disc of radius R and mass M about an axis passing from the edge of the disc and normal to the disc is
View SolutionThe moment of inertia of a uniform circular disc of radius R and mass M about an axis passing from the edge of the disc and normal to the disc is.
View SolutionA disc of radius R is pivoted at its rim. The period for small oscillations about an axis perpendicular to the plane of disc is
View Solution

