What work has to be performed to make a hoop out of a steel band of length l=2.0m, width h=6.0cm, and thickness δ=2.0mm? The process is assumed to proceed within the elasticity range of the material.
The work done to make a loop out of a steel band appears as the elastic energy of the loop and may be calculated from the same.
If the length of the band is l, the radius of the loop R=l2π. Now consider an element ABCD of the loop. The elastic energy of this element can be calculated by the same sort of arguments as used to derive the formula for internal bending moment. Consider a fibre at a distance z from the neutral surface PQ. This fibre experiences a force p and undergoes an extension ds where ds=Zdφ, while PQ=s=Rdφ. Thus strain dss=ZR. If α is the cross sectional area of the fibre, the elastic energy associated with it is
12E(ZR)2Rdφα
Summing over all the fibres we get
EIφ2R∑αZ2=Eldφ2R
For the whole loop this gives,
using ∫dφ=2π,
ElπR=2EIπ2l
Now I=δ/2∫−∂ts/2Z2hdZ=hδ312
So the energy is 16π2Ehδ3l=0⋅08kJ

Topper's Solved these Questions
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Hydrodynamics25videos
PHYSICAL FUNDAMENTALS OF MECHANICS
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Relativistic Mechanics49videos
PHYSICAL FUNDAMENTALS OF MECHANICS
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Dynamics Of A Solid Body56videos
PHYSICAL FUNDAMENTALS OF MECHANICS
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Electromagnetic Waves, Radiation36videos
OSCILLATIONS AND WAVES
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - THERMODYNAMICS AND MOLECULAR PHYSICSEXERCISE - Transport Phenomena38videos
THERMODYNAMICS AND MOLECULAR PHYSICS
Similar Questions
What work has to be done to make a hoop out of a copper band of length l=3m,width h=6 cm and thickness δ=1mm? (Y of copper =1.3×1011Nm−2 )
[Hint: Consider strain energy in a a thin layer and intergraate from r−δ/2tor+δ/2]View SolutionA rectangular air mattress has a length 2.0 m, a width 0.50 m and thickness can float on water if the mass of the mattress is 2.0 kg. what is the density of the mattress ?
View Solution
Knowledge Check
- Question 1 - Select One
What works has to be performed to make a hoop out of a steel band of length l, width h and thickness δ? The process is assumed to proceed within the elasticity range of the material.
Aπ2Ebδ3/lBπ3Ebδ3/lCπ4Ebδ3/lDπ5Ebδ3/l - Question 2 - Select One
A wire 100cm long and 2.0mm diameter has a resistance of 0.7 ohm , the electrical resistivity of the material is
A4.4×10−6ohm×mB2.2×10−6ohm×mC1.1×10−6ohm×mD0.22×10−6ohm×m - Question 3 - Select One
If the force required to pull out a glass plate of length 9.8 cm and thickness 2 mm from a liquid is 0.6 gmwt. The surface tension of water is Nm−1
A2.94×10−3B29.4×103C29.4×10−2D29.4×10−3
Find the resistance of a hollow cylindrical conductor of length 1.0m and inner and outer radii 1.0mm and 2.0mm respectively. The resistivity of the material is 2.0×10−8(Ω)m.
View SolutionFind the resistance of a hollow cylindrical conductor of length 1.0m and inner and outer radii 1.0mm and 2.0mm respectively. The resistivity of the material is 2.0×10−8(Ω)m.
View SolutionFind the resistance of a hollow cylindrical conductor of length 1.0m and inner and outer radii 1.0mm and 2.0mm respectively. The resistivity of the material is 2.0×10−8(Ω)m.
View SolutionFigure shows an aluminium wire of length 60 cm joined to a steel wire of length 80 cm and stretched between two fixed supports. The tension produced is 40 N. The cross-sectional area of the steel wire is .1.0mm2 and that of the aluminium wire is 3.0 mm 2. What could be the minimum frequency of a tuning fork which can produce standing waves in the system with the joint as a node ? The density of aluminium is 2.6gcm−3and that of steel is 7.8gcm−3
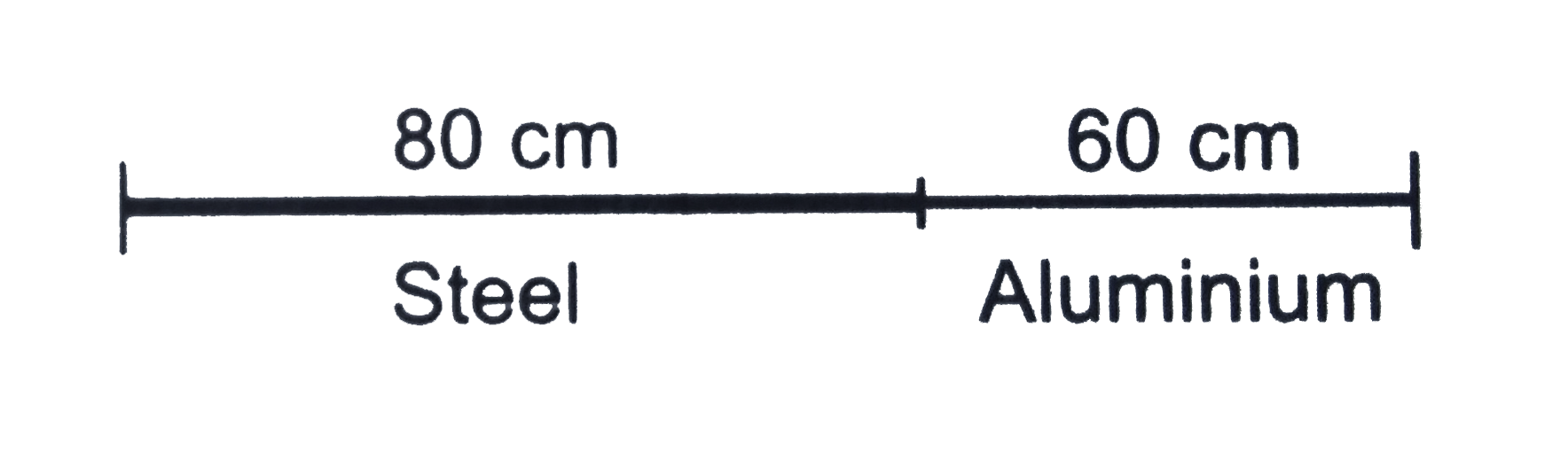 View Solution
View SolutionTwo discs of the same material and thickness have radii 0.2 m and 0.6 m. Their moments of inertia about their axes will be in the ratio of
View Solution

