PQRS
is a cyclic
quadrilateral such that PR
is a diameter of the circle. If ∠QPR=67∘and∠SPR=72∘,
then ∠QRS=
(a)41∘
(b) 23∘
(c) 67∘
(d) 18∘
Video Solution
Text Solution
Angle in a semicircle is 90∘
Thus ∠PQR=∠PSR=90∘
∠QPS=∠QPR+∠RPS=67∘+72∘=139∘
Sum of all angles of quadrilateral must be 360∘
Thus, 90∘+90∘+139∘+∠QRS=360∘
∠QRS=41∘
|
Topper's Solved these Questions
Similar Questions
Knowledge Check
If P:Q=2:3,Q:R=5:7andR:S=3:10 then P : S = ?
A1:5B1:7C3:2D6:15If P is the husband of Q and R is the mother of S and Q, what is R to P?
A(a) MotherB(b) SisterC(c) AuntD(d) Mother-in-lawP is Q's brother. R is Q's mother. S is R's father. T is S's mother. How is P related to T?
P, Q का भाई है। R, Q की माता है। S, R का पिता है। T, S की माता है। P, T से किस प्रकार संबंधित है?AGranddaughter (पोती)BGreat grandson (परपोता)CGrandson (पोता)DGrandmother (दादी)
In Fig. 6.31, if PQ∣∣ST,∠PQR=110∘ and∠RST=130∘, find ∠QRS.
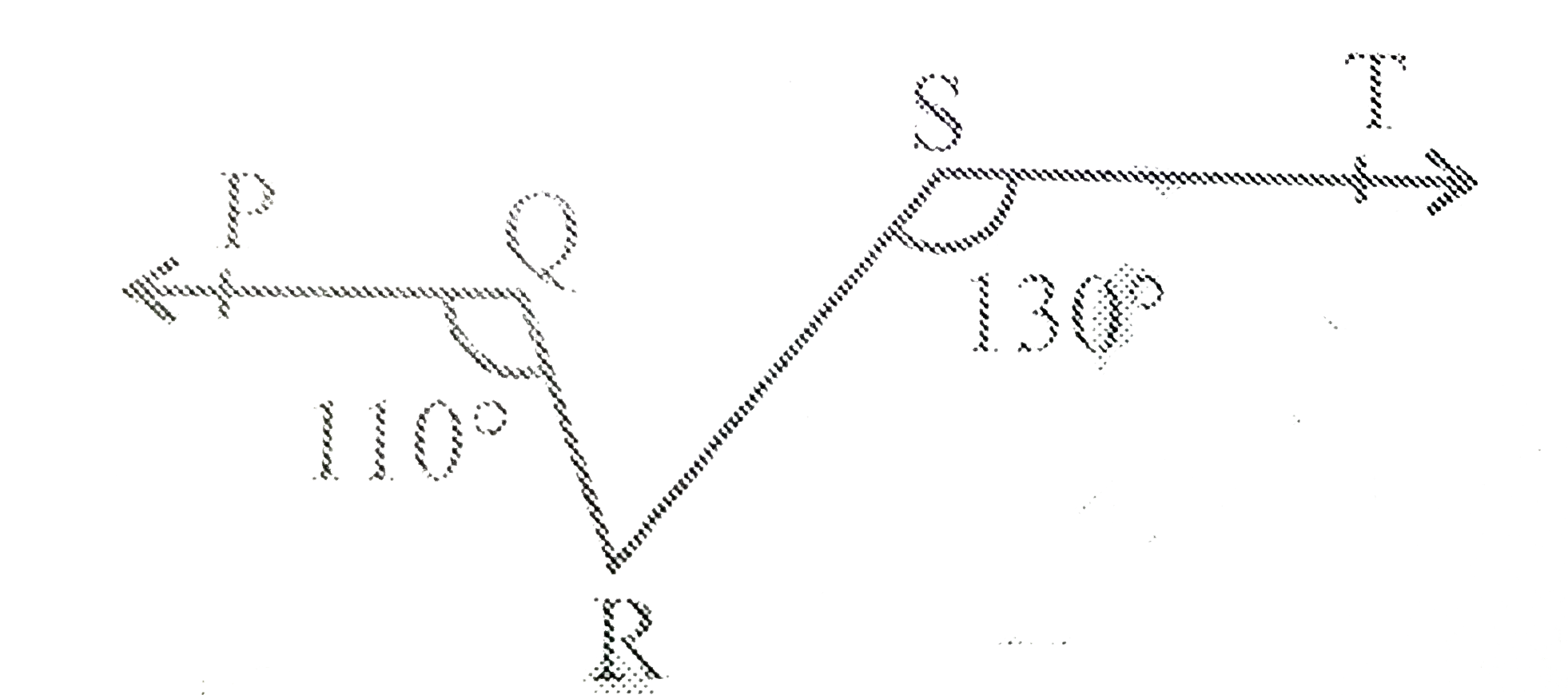
View SolutionABCD is a cyclic quadrilateral. The bisectors of the angles A, B, C and D. cut the circle at P, Q, R and S respectively. What is ∠PQR+∠RSP equal to ?
View SolutionIn Fig. 6.15, ∠PQR=∠PRQ, then prove that ∠PQS=∠PRT.

View SolutionIn Figure, PQRS is a quadrilateral. PQ is its longest side and RS is its shortest side. Prove that ∠R> ∠P and ∠S> ∠Q Given : PQRS is a quadrilateral. PQ is its longest side and RS is its shortest side. To Prove : (i) ∠R> ∠P (ii) ∠S> ∠Q Construction : Joint PR and QS
View SolutionIn figure PS is the bisector of ∠QPRof ΔPQR. Prove that QSSR=PQPR.

View Solution
