The following is the distribution of weights (in kg) of 50 persons: Marks:, 50-55, 55-60, 60-65, 65-70, 70-75, 75-80, 80-85, 85-90 No. of Person, 12, 8, 5, 4, 5, 7, 6, 3 Draw a histogram for the above data.
To draw a histogram for the given distribution of weights of 50 persons, follow these steps:
Step 1: Understand the Data
The data provided consists of weight intervals (in kg) and the corresponding number of persons in each interval. The intervals and their frequencies are:
- 50-55 kg: 12 persons
- 55-60 kg: 8 persons
- 60-65 kg: 5 persons
- 65-70 kg: 4 persons
- 70-75 kg: 5 persons
- 75-80 kg: 7 persons
- 80-85 kg: 6 persons
- 85-90 kg: 3 persons
Step 2: Set Up the Axes
- Draw two perpendicular lines to create the axes of the histogram.
- The horizontal axis (x-axis) will represent the weight intervals (50-55, 55-60, etc.).
- The vertical axis (y-axis) will represent the number of persons. Choose a suitable scale for the y-axis, such as increments of 2 or 4, up to the maximum frequency (which is 12).
Step 3: Mark the Intervals on the X-axis
- Divide the x-axis into equal parts for each weight interval. Each interval should be represented as a bar that spans the width of the interval.
- Mark the intervals: 50-55, 55-60, 60-65, 65-70, 70-75, 75-80, 80-85, and 85-90.
Step 4: Plot the Frequencies
- For each interval, draw a vertical bar that corresponds to the number of persons in that interval:
- For 50-55 kg, draw a bar up to 12 on the y-axis.
- For 55-60 kg, draw a bar up to 8.
- For 60-65 kg, draw a bar up to 5.
- For 65-70 kg, draw a bar up to 4.
- For 70-75 kg, draw a bar up to 5.
- For 75-80 kg, draw a bar up to 7.
- For 80-85 kg, draw a bar up to 6.
- For 85-90 kg, draw a bar up to 3.
Step 5: Draw the Bars
- Make sure each bar is of equal width and is adjacent to the next bar (no gaps between bars).
- Use a ruler to ensure the bars are straight and evenly spaced.
Step 6: Label the Histogram
- Label the x-axis as "Weight (kg)" and the y-axis as "Number of Persons".
- Optionally, you can also label each bar with the corresponding frequency for clarity.
Step 7: Review the Histogram
- Check that all bars are correctly drawn and that the heights correspond to the frequencies given in the data.
The data provided consists of weight intervals (in kg) and the corresponding number of persons in each interval. The intervals and their frequencies are:
- 55-60 kg: 8 persons
- 60-65 kg: 5 persons
- 65-70 kg: 4 persons
- 70-75 kg: 5 persons
- 75-80 kg: 7 persons
- 80-85 kg: 6 persons
- 85-90 kg: 3 persons
- Draw two perpendicular lines to create the axes of the histogram.
- The horizontal axis (x-axis) will represent the weight intervals (50-55, 55-60, etc.).
- The vertical axis (y-axis) will represent the number of persons. Choose a suitable scale for the y-axis, such as increments of 2 or 4, up to the maximum frequency (which is 12).
- Divide the x-axis into equal parts for each weight interval. Each interval should be represented as a bar that spans the width of the interval.
- Mark the intervals: 50-55, 55-60, 60-65, 65-70, 70-75, 75-80, 80-85, and 85-90.
- For each interval, draw a vertical bar that corresponds to the number of persons in that interval:
- For 50-55 kg, draw a bar up to 12 on the y-axis.
- For 55-60 kg, draw a bar up to 8.
- For 60-65 kg, draw a bar up to 5.
- For 65-70 kg, draw a bar up to 4.
- For 70-75 kg, draw a bar up to 5.
- For 75-80 kg, draw a bar up to 7.
- For 80-85 kg, draw a bar up to 6.
- For 85-90 kg, draw a bar up to 3.
- Make sure each bar is of equal width and is adjacent to the next bar (no gaps between bars).
- Use a ruler to ensure the bars are straight and evenly spaced.
- Label the x-axis as "Weight (kg)" and the y-axis as "Number of Persons".
- Optionally, you can also label each bar with the corresponding frequency for clarity.
- Check that all bars are correctly drawn and that the heights correspond to the frequencies given in the data.
Topper's Solved these Questions
- DATA HANDLING-I (CLASSIFICATION AND TABULATION DATA)Book:RD SHARMAChapter:DATA HANDLING-I (CLASSIFICATION AND TABULATION DATA)Exercise:All Questions
Explore 27 Videos
- DATA HANDLING-III (PIE CHARTS OR CIRCLE GRAPHS)Book:RD SHARMAChapter:DATA HANDLING-III (PIE CHARTS OR CIRCLE GRAPHS)Exercise:All Questions
Explore 32 Videos
Similar Questions
The following is the distribution of weights (in kg) of 52 persons:
⎧⎪ ⎪ ⎪ ⎪ ⎪ ⎪ ⎪ ⎪ ⎪⎨⎪ ⎪ ⎪ ⎪ ⎪ ⎪ ⎪ ⎪ ⎪⎩Weight in kgPerson30−401040−501550−601760−70670−804
What is the lower limit of class 50-60?
Find the class marks of the classes 40-50, 50-60
What is the class size?
View SolutionThe following table gives production yield per hectare of wheat of 100 farms of a village: Production yield 50-55 55-60 60-65 65-70 70-75 75-80 in kg per hectare: No. of farms: 2 8 12 24 38 16 Draw ‘less than’ ogive and ‘more than’ ogive.
View Solution
Knowledge Check
The modal class for the following frequency distribution, is
Marks:0−1010−2020−4040−5050−6060−7070−9090−100No. of students:461416148165A20-40B40-50C50-60D70-90
Calculate the mean and standard deviation for the following distribution: Marks:, 20-30, 30-40, 40-50, 50-60, 60-70, 70-80, 80-90 No. of students:, 3, 6, 13, 15, 14, 5, 4
View SolutionThe distribution below gives the weight of 30 students in a class. Find the median weight of students: Weight (in kg): 40-45 45-50 50-55 55-60 60-65 65-70 70-75 No. of students: 2 3 8 6 6 3 2
View SolutionThe following table shows the distribution of the percentage of marks of a group of students:
Percentage of Marks30−4040−5050−6060−7070−8080−90Number of Students4113752
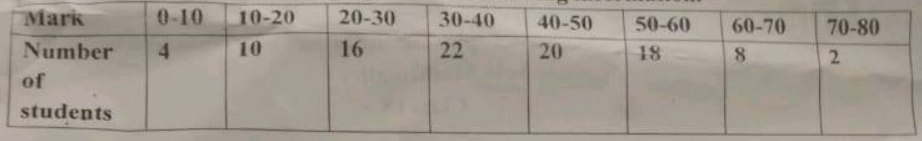
View SolutionThe following table gives the marks scored by 100 students in an entrance examination.
View SolutionA car is going for a long journey of 16 hours, starting at 5:00 hrs. The speeds of the car at different hours are given below:
Time (in hours):, 5:00, 7:00, 9:00, 11:00, 13:00, 15:00, 17:00, 19:00, 21:00
Speed (in km/hour):, 40, 50, 60, 80, 70, 65, 75, 60, 50
Draw a velocity-time graph for the above data:
View SolutionCalculate the median from the following data: Rent (in Rs.): 15-25 25-35 35-45 45-55 55-65 65-75 75-85 85-95 No. of Houses: 8 10 15 25 40 20 15 7
View SolutionGiven the following frequency distribution with some missing frequencies
{(10−20,20−30,30−40,40−50,50−60,60−70,70−80),(Frequency,180,?,34,180,136,?,50)
If the total frequency is 685 and median is 42.6 then the missing frequencies are
View Solution