One of the important approach of the study of real gases involves the analysis of a parameter Z called the compressibility factor Z=PVmRT where P is pressure, Vm is molar volume ,T is absolute temperature and R is the universal gas constant .Such a relation can also be expressed as Z=(V m realVm ideal) (where Vm ideal and V_("m real") are the moler volume for ideal and real gas respectively tendencies among constituent particles.As pressure is force among is lowered or temperature is increased the value of Z approaches ideal behaviour
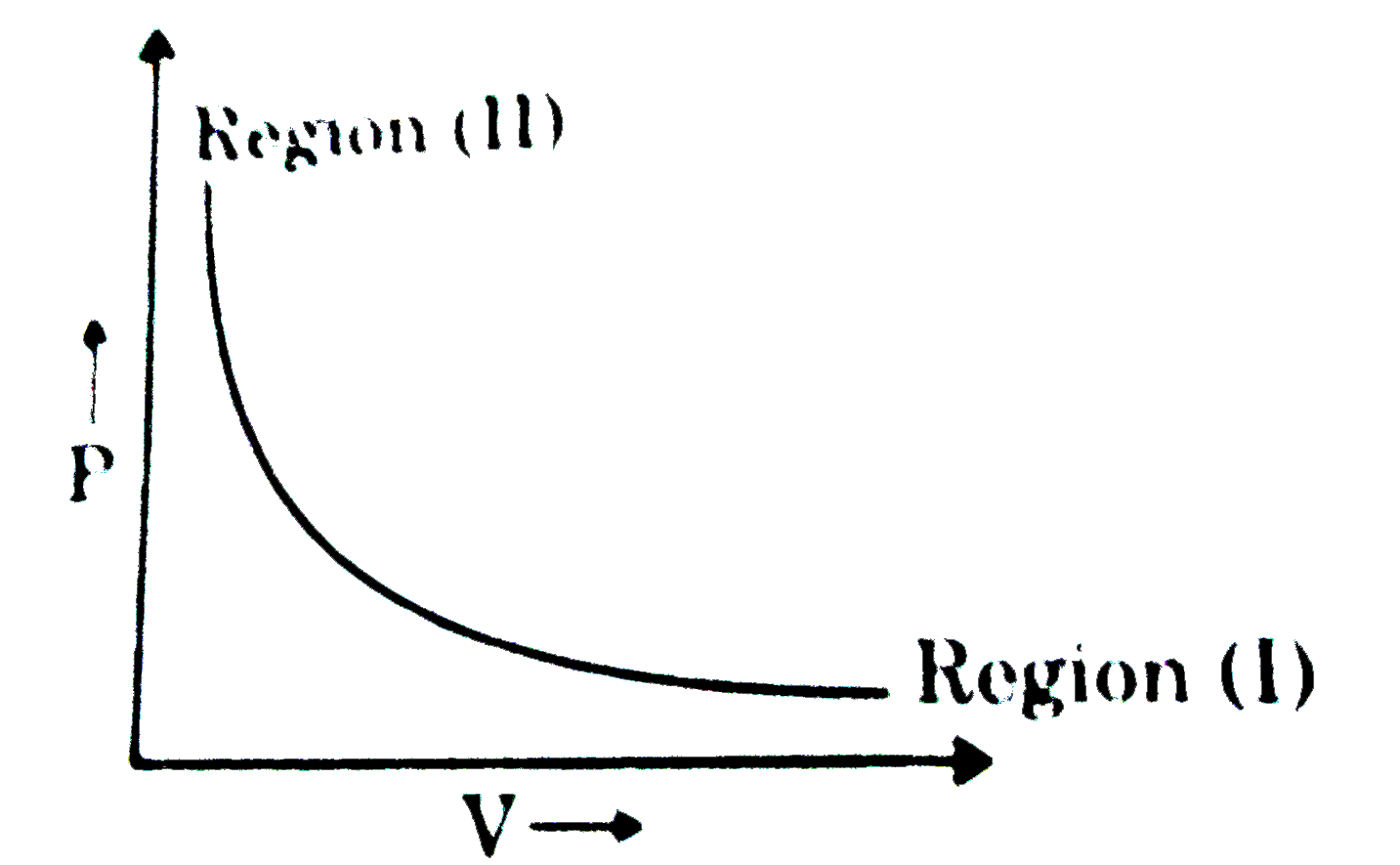
Following graph represents a pressure (P) volume (V) relationship at a fixed temperature (T) for moles of a real gas.The graph has two regions masked (I) and (II). Which of the folloing option true ?
Z<1 in the region (II)
Z = 1 in the region (II)
Z = 1 for the curve
Z approaches 1 as we move from region (II) region(I)
The correct Answer is:D
Topper's Solved these Questions
- GRAPHICAL INTERPRETATIONBook:GRB PUBLICATIONChapter:GRAPHICAL INTERPRETATIONExercise:Comprehension 2
Explore 1 Video
- GRAPHICAL INTERPRETATIONBook:GRB PUBLICATIONChapter:GRAPHICAL INTERPRETATIONExercise:Comprehension 3
Explore 1 Video
- GRAPHICAL INTERPRETATIONBook:GRB PUBLICATIONChapter:GRAPHICAL INTERPRETATIONExercise:Comprehension 1
Explore 1 Video
- F-BLOCK ELEMENTSBook:GRB PUBLICATIONChapter:F-BLOCK ELEMENTSExercise:Subjective type
Explore 7 Videos
- HYDROCARBON (ALIPHATIC)Book:GRB PUBLICATIONChapter:HYDROCARBON (ALIPHATIC)Exercise:Previous years jee questions
Explore 28 Videos
Similar Questions
One mole of an ideal gas undergoes a process (10L, 4atm) to (4L, 10 atm ). The process may be represented by straight line in P-V diagram
The correct statement for the process :
View SolutionIn order to perdict variation of Gibbs free energy with progress of reaction at constant temperature and 1 bar pressure, it is important to know realtive stability of the components involved in chemical reaction at standard useful work then the change in Gibbs free energy should be calculated
Which of the following options correctly represects the graph between Gibbs free energy (G0 and state of reaction (SOR) at 1 bar and 300 K for the reaction But -1 ene (A) ⇔ but -2 ene (B)
View SolutionEvery reaction whether started by taking only reactants or by taking only products or any other combination always has a tendency to move towards equilibrium. Hence, equilibrium state can be considered as the one with most stable composition. In case of isomers, based on stability data position of equilibrium can als be decided.
Which of the following options correctly represent ΔG∘f of cis but-2-ene, trans but-2-ene and but-1-ene in kJ/mole?
View SolutionThe first f-block organometallic compound [ThH(OR)(C5Me5)2] was prepared in late 1970s. Find the oxidation state of central metal atom/cation.
View SolutionWhich of the following will decolourise alkaline KMnO4?
View SolutionAt Boyle's temperature the value of compressibility factor Z=(PV_(m)//RT=V_("real")//V_("ideal")) has a value of 1, over wide range of pressure. This is due to the fact that in the van der Waal's equation:
View SolutionOne of the important approach of the study of real gases involves the analysis of a parameter Z called the compressibility factor Z=PVmRT where P is pressure, Vm is molar volume ,T is absolute temperature and R is the universal gas constant .Such a relation can also be expressed as Z=(V m realVm ideal) (where Vm ideal and V_("m real") are the moler volume for ideal and real gas respectively tendencies among constituent particles.As pressure is force among is lowered or temperature is increased the value of Z approaches ideal behaviour
Choose the conslusions which are appropriate for the observation stated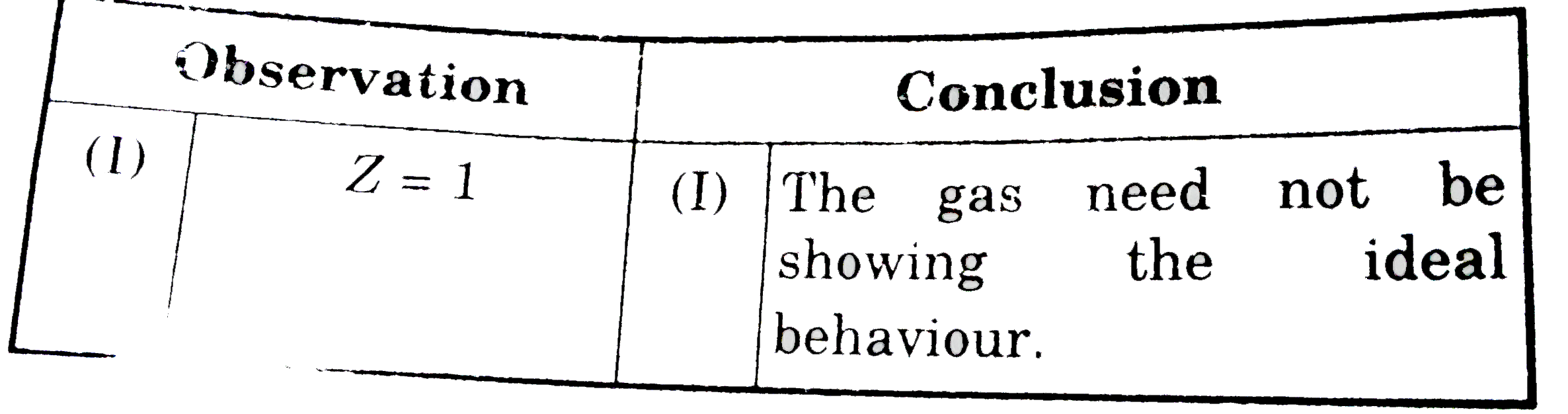
View SolutionOne of the important approach of the study of real gases involves the analysis of a parameter Z called the compressibility factor Z=PVmRT where P is pressure, Vm is molar volume ,T is absolute temperature and R is the universal gas constant .Such a relation can also be expressed as Z=(V m realVm ideal) (where Vm ideal and V_("m real")arethemo≤rvolumeforalandrealgasrespectivelytendenciesamongconstituentpartic≤s.Aspressureisforceamongisloweredortemperatureis∈creasedthevalueofZapproachesalbehaviour<br>Forarealgas'G',Zgt1 at STP, then for 'G' which of the following is true ?
View SolutionAt Boyle's temperature the value of compressibility factro Z=(PVm(/RT=Vreal/Val) has a value of 1 over a wide range of pressure. This is due to the fact that in the van der Waal's equation
View SolutionOne of the important approach to the study of real gases involves the analysis of parameter Z called the compressibility factor Z=PVmRT where P is pressure, Vm is molar volume, T is absolute temperature and R is the universal gas constant. such a relation can also be expressed as Z=(VmrealVmal) (where Vmal and Vmreal are the molar volume for ideal and real gas respectively). Gas corresponding Z<1 have attractive forces amoung constituent particles. As the pressure is lowered or temperature is increased the value of Z approaches 1. (reaching the ideal behaviour)
ObservationConclusionI.Z=1I.The gas need not be showing the ideal behaviourII.Z>1II.On applying pressure the gas will respond byincreasing its volumeIII.Z<1III.The gas may be liquefiedIV.Z→1for lowPIV.The gas is approaching the ideal behaviour
View SolutionOne of the important approach to the study of real gases involves the analysis of parameter Z called the compressibility factor Z=PVmRT where P is pressure, Vm is molar volume, T is absolute temperature and R is the universal gas constant. such a relation can also be expressed as Z=(VmrealVmal) (where Vmal and Vmreal are the molar volume for ideal and real gas respectively). Gas corresponding Z<1 have attractive forces amoung constituent particles. As the pressure is lowered or temperature is increased the value of Z approaches 1. (reaching the ideal behaviour)
For a real gas GZ>1 at STP Then for 'G': Which of the following is true:
View SolutionOne of the important approach to the study of real gases involves the analysis of parameter Z called the compressibility factor Z = (PV_(m))/(RT) where P is pressure, V_(m) is molar volume, T is absolute temperature and R is the universal gas constant. such a relation can also be expressed as Z = ((V_(m)real)/(V_(m)ideal)) (where V_(m ideal) and V_(m real) are the molar volume for ideal and real gas respectively). Gas corresponding Z lt 1 have attractive forces amoung constituent particles. As the pressure is lowered or temperature is increased the value of Z approaches 1. (reaching the ideal behaviour) Following graph represents a pressure (P) volume (V) relationship at a fixed temperature (T) for n moles of a real gas. The graph has two regions marked (I) and (II) . Which of the following options is true.
View SolutionOne of the approach to the study of real gases involves the analysis of a parameter Z called the compressibility factor Z=(PV_(m))/(RT) where P is pressure, V_(m) is molar volume, T is absolute temperature and R is the universal gas constant . Such a relation can also be expressed as Z =((V_("m real"))/(V_("m ideal"))) (where V_("m ideal ") and V_("m real") are the molar volume for ideal and real gas respectively) . Gas corresponding Z gt 1 have repluive tendenciesamong constituent particles due to their size factor, among constitutent particles . As the pressure is lowered or temperature is increased the value of Z approaches 1. ( reaching the ideal behaviour) For a real gas 'G'Z gt 1 at STP then for 'G' which of the following is true
View SolutionFor a real gas with very large value of molar volume, which of the following equation can most suitably be applied: (A) Z = 1 - (a)/(V_(m)RT) (B) PV =RT (C) Z = 1+ (Pb)/(RT) (D) PV_(m) -RT = (a)/(V_(m)
View Solutionवास्तविक गैसों के अध्ययन की एक महत्वपूर्ण पहुँच जिसमें पैरामीटर Z का विश्लेषण शामिल है सम्पीड़यता गुणांक Z=(PVm)RT) कहलाता है जहाँ P दाब है,Vm मोलर आयतन है, T परम ताप है तथा R सार्वत्रिक गैस स्थिरांक है। अतः इस संबंध को इस प्रकार भी प्रदर्शित कर सकते है Z=((V“mवास्तविक”V“m”आदर्श (जही V“mआदर्श” तथा V“mवास्तविक” क्रमशः आदर्श तथा वास्तविक गैस के लिए मोलर आयतन है)। Z>1 अनुरूप गैस के घटक कणों के मध्य उनके आकार गुणांक के कारण प्रतिकर्षण की प्रवृति होती है जबकि जो गैस Z<1 के अनुरूप होती है उनके घटक कणों के मध्य आकर्षण बल होता है जैसे-जैसे दाब कम होता है या ताप अधिक होता है तब 2 का मान 1 तक पहुँच जाता है (आदर्श व्यवहार तक पहुंच जाता है)।
निष्कर्ष को चुनिए जो कि नियमित निरीक्षण के लिए योग्य हो

View Solution
