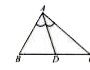
In following figure if AD if the bisector of ∠ABC, then prove that AB>BD
Video Solution
Text Solution
Given ABC is a triangle such that AD is the bisector of ∠ABC
To Prove AB>BD
Proof Since ,AD if the bisector of ∠BAC.
∴ ∠BAD=CAD ….(i)
But ∠ADB>∠CAD
[exterior angle of a triangle is greater than each of the opposite interior angle ]
∴ ∠ABD>∠CAD [from Eq.(i)]
⇒ AB>BD [side opposite to greater angle is longer ]
|
Topper's Solved these Questions
Similar Questions
Knowledge Check
In the given figure , if AD is the bisector of ∠BAC . If AB=10 cm , AC = 14 cm and BC = 6 cm , then find BD and DC .
 A2.5 cm , 3.5 cmB3 cm , 3 cmC3.5 cm , 4.5 cmD4 cm , 2 cm
A2.5 cm , 3.5 cmB3 cm , 3 cmC3.5 cm , 4.5 cmD4 cm , 2 cm
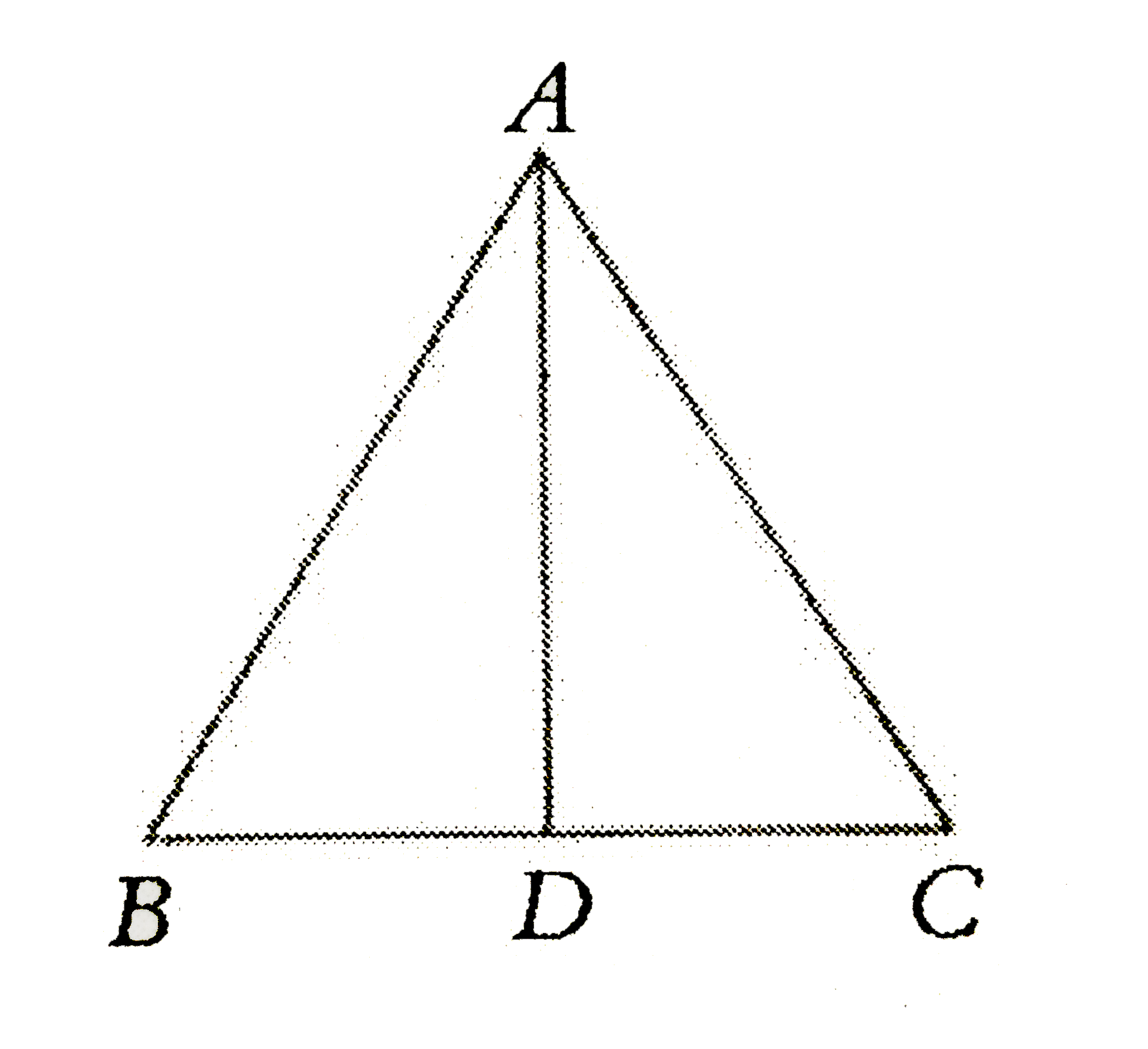
In the given figure, AD is the bisector of ∠BAC. Prove that triangles ABD and ADC are congruent, if AB=AC.
View SolutionIn Figure, if AD is the bisector of LA , show that: AB>BD (ii) AC>CD
View SolutionIn the given figure of AD = CB then prove that AC = BD

View SolutionIn the given figure if AC=BD then prove that AB=CD
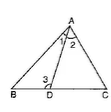
View SolutionIn the given figure, AC>AB and AD is the bisector of ∠A. show that ∠ADC>∠ADB.
View SolutionIn the adjoining figure, ΔABC is an isosceles triangle in which AB = AC and AD is the bisector of ∠A. Prove that:

BD = CD
View SolutionIn the given figure, if AC = BD, then prove that AB = CD.

View Solution