Consider the force F on a charge 'q' due to a uniformlycharged spherical shell of radius R carrying charge Q distributed uniformly over it. Which one of the following statements is true for F, if 'q' is placed at distacen r from the centre of the shell?
F=14π∈0QqR2 for r<R
14π∈0qQR2>F>0 for r<R
F−14π∈0Qqr2 for r>R
F=14π∈0Qqr2 for all r
To solve the problem, we need to analyze the force F on a charge q placed at a distance r from the center of a uniformly charged spherical shell of radius R carrying a total charge Q.
1. Understanding the Setup:
- We have a uniformly charged spherical shell with radius R and total charge Q.
- A charge q is placed at a distance r from the center of the shell.
2. Applying Gauss's Law:
- According to Gauss's law, the electric field E due to a spherical charge distribution can be analyzed based on the position of the point charge q relative to the shell.
- Gauss's law states:
∮E⋅dA=Qencϵ0
- Where Qenc is the enclosed charge within the Gaussian surface.
3. Case 1: When r<R:
- If the charge q is located inside the shell (i.e., r<R), the enclosed charge Qenc=0 because there is no charge inside the shell.
- Therefore, from Gauss's law:
E=0
- The force F on charge q is given by:
F=q⋅E=q⋅0=0
4. Case 2: When r≥R:
- If the charge q is located outside the shell (i.e., r≥R), the entire charge Q of the shell can be considered to be concentrated at the center.
- The electric field E at a distance r from the center is given by:
E=14πϵ0⋅Qr2
- The force F on charge q is then:
F=q⋅E=q⋅(14πϵ0⋅Qr2)=qQ4πϵ0r2
5. Conclusion:
- For r<R, F=0.
- For r≥R, F=qQ4πϵ0r2.
- Thus, the correct statement regarding the force F is that it is zero when r<R and follows the inverse square law when r≥R.
- We have a uniformly charged spherical shell with radius R and total charge Q.
- A charge q is placed at a distance r from the center of the shell.
- According to Gauss's law, the electric field E due to a spherical charge distribution can be analyzed based on the position of the point charge q relative to the shell.
- Gauss's law states:
∮E⋅dA=Qencϵ0
- Where Qenc is the enclosed charge within the Gaussian surface.
- If the charge q is located inside the shell (i.e., r<R), the enclosed charge Qenc=0 because there is no charge inside the shell.
- Therefore, from Gauss's law:
E=0
- The force F on charge q is given by:
F=q⋅E=q⋅0=0
- If the charge q is located outside the shell (i.e., r≥R), the entire charge Q of the shell can be considered to be concentrated at the center.
- The electric field E at a distance r from the center is given by:
E=14πϵ0⋅Qr2
- The force F on charge q is then:
F=q⋅E=q⋅(14πϵ0⋅Qr2)=qQ4πϵ0r2
- For r<R, F=0.
- For r≥R, F=qQ4πϵ0r2.
- Thus, the correct statement regarding the force F is that it is zero when r<R and follows the inverse square law when r≥R.
Topper's Solved these Questions
Similar Questions
Find out energy stored in the electric field of uniformly charged thin spherical shell of total charge Q and radius R.
View SolutionOver a thin ring of radius R a charge q is distributed none-uniformly. Calculate the work done of the force field in displacing a point charge q' from centre of the ring to infinity.
View Solution
Knowledge Check
A thin spherical conducting shell of radius R has a charge q. Another charge Q is placed at the centre of the shell. The electrostatic potential at a point P a distance R2 from the centre of the shell is
A(a) 2Q4πε0RB(b) 2Q4πε0R−2q4πε0RC(c) 2Q4πε0R+q4πε0RD(d) (q+Q)24πε0RA spherical shell of radius R has a charge +q units. The electric field due to the shell at a point
Ainside is zero and varies as r−1 outside itBinside is constant and varies as r−2Cinside is zero and varies as r−2 outside itDinside is constant and varies as r−1 outside itThe electrostatic potential of a uniformly charged thin spherical shell of charge Q and radius R at a distance r from the centre
AQ4πε0r for points outside and Q4πε0R for points inside the shellBQ4πε0e for both points inside nad outside the shellCzero for points outside and Q4πε0r for points inside the shellDzero for both points inside and outside the shell
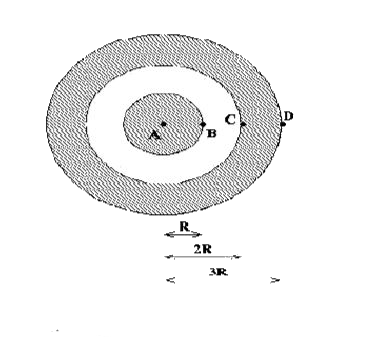
A solid spherical conductor has charge +Q and radius R. It is surrounded by a solid spherical shell with charge -Q, innerradius 2R, and outer radius 3R. Which of the following statements is true?
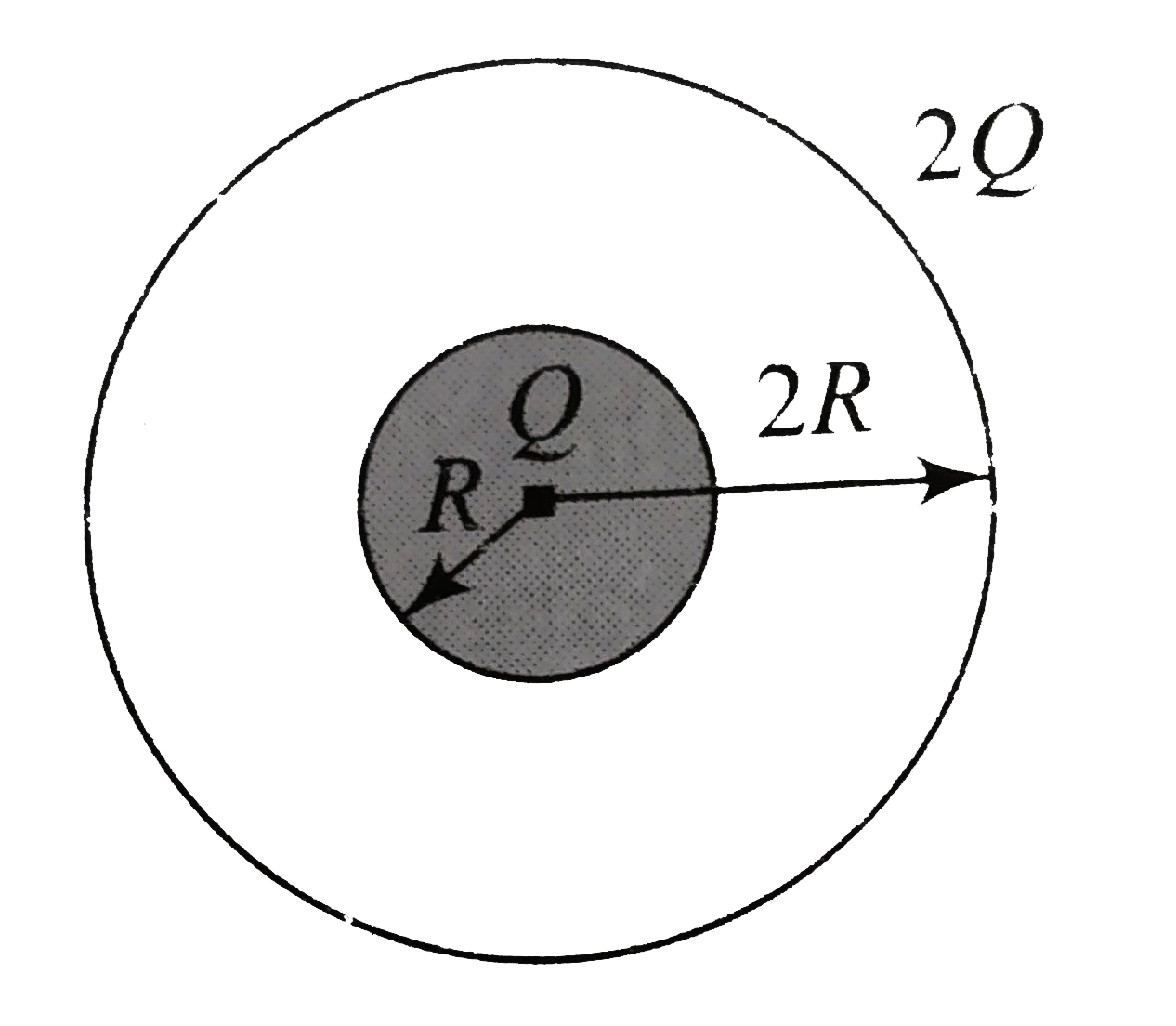
View SolutionA charge Q is uniformly distributed in a dielectric sphere of radius R (having dielectric constant unity). This dielectric sphere is enclosed by a concentric spherical shell of radius 2R and having uniformly distributed charge 2Q. Which of the following graph correctly represents variation of electric field with distance r from the common centre?
View SolutionA spherical shell of radius R=1.5cm has a charge q=20μC uniformly distributed over it. The force exerted by one half over the other half is
View SolutionA thin spherical shell of radius R has charge Q spread uniformly over its surface. Which of the following graphs most closely represents the electric field E(r) produced by the shell in the range 0≤r<∞, where r is the distance from the centre of the shell?
View SolutionA thin spherical conducting shell of radius R has a charge q. Another charge Q is placed at the centre of the shell. The electrostatic potential at a point P a distance R2 from the centre of the shell is
View Solution
