Doubtnut Promotions Sticky

(a) Can the graph of the function intersect the horizontal asymptote?
(b) Can the graph of the function intersect the vertical asymptote?

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

(a) Yes. See the following graph for illustration.
ocr_image
(b) No. If the fraph of the curve intersects the vertical asymptote, then it is not a function as it fails the vertical line test.
ocr_image

|
Updated on:21/07/2023
Class 12MATHSFUNDAMENTAL OF MATHEMATICS

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • Question 1 - Select One

    The graphs of x=aandy=b intersect at

    A(a,b)
    B(b,a)
    C(-a,b)
    D(a,-b)
  • Question 2 - Select One

    The function represented by the following graph is
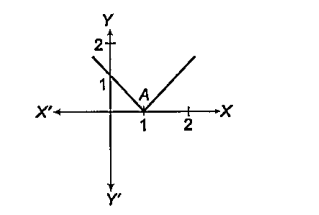    ocr_image

    Acontinuous but not differentiable at x=1
    Bdifferentiable but not continuous at x=1
    Ccontinuous and differentiable at x=1
    Dneither continuous nor differentiable at x=1
  • Question 3 - Select One

    Which of the following is false?
    (i) If only the positive values of x are taken, the quadratic graph does not intersect the K-axis.
    (ii) if only negative values are taken, the quadratic graph does not intersect the X-axis.
    (iii)The intercept of X-axis can be known when we substitute x =0, in the quadratic function.
    (iv)There can be minimum zero and maximum two x-intercepts .

    A(A) (i) and (iv)
    B(B) (i),(ii),(iii)and (iv)
    C(C) (i),(ii) and (iii)
    D(D) none of these