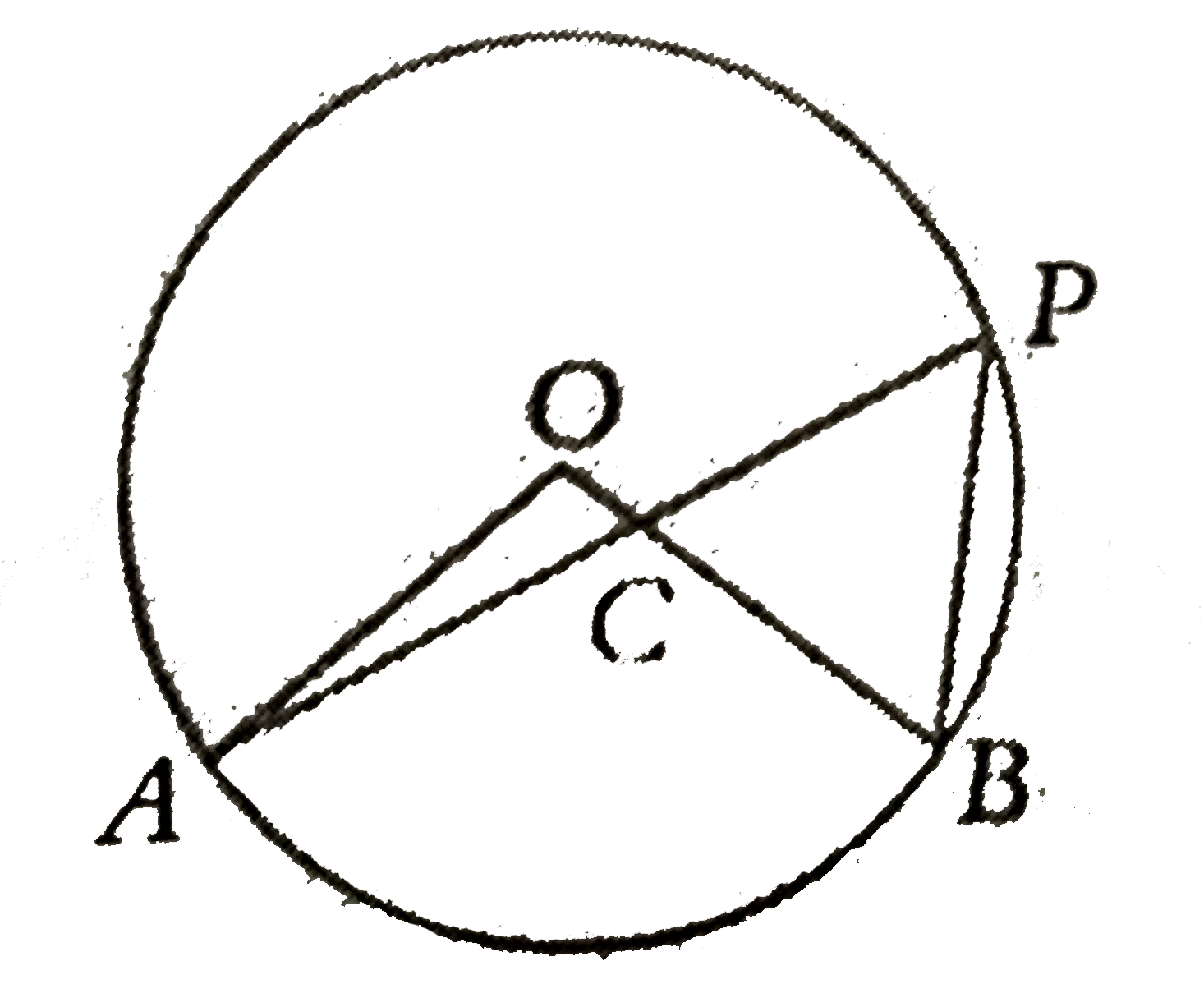
In the above figure (not to scale), O is the centre of the circle. ¯¯¯¯¯¯AP and ¯¯¯¯¯¯BP are two chords. C is the point of intersection of ¯¯¯¯¯¯AP and ¯¯¯¯¯¯OB. If ∠OAC=30∘ and ∠PBC=80∘, then ∠AOB=
110∘
100∘
130∘
120∘
The correct Answer is:B
(i) Sum of the angles of a triangle is 180∘. Vertically opposite angles are equal.
(ii) Use the relation ∠APB=12∠AOB=x.
(iii) ∠OCA=∠PCB. ( as they are vertically opposite angles )
(iv) Sum of the angles of a triagnel is 180∘.
Topper's Solved these Questions
- BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - GEOMETRY EXERCISE - Level 220videos
GEOMETRY
- BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - GEOMETRY EXERCISE - Level 37videos
GEOMETRY
- BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - GEOMETRY EXERCISE - Essay type Question3videos
GEOMETRY
- BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - FormulaeEXERCISE - Level 37videos
Formulae
- BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - INDICESEXERCISE - CONCEPT APPLICATION (Level 3)7videos
INDICES
Similar Questions
Knowledge Check
- Question 1 - Select One

In the above figure (not to scale), O is the centre of the circle. ¯¯¯¯¯¯AP and ¯¯¯¯¯¯BP are two chords. C is the point of intersection of ¯¯¯¯¯¯AP and ¯¯¯¯¯¯OB. If ∠OAC=30∘ and ∠PBC=80∘, then ∠AOB=A110∘B100∘C30∘D120∘ - Question 2 - Select One
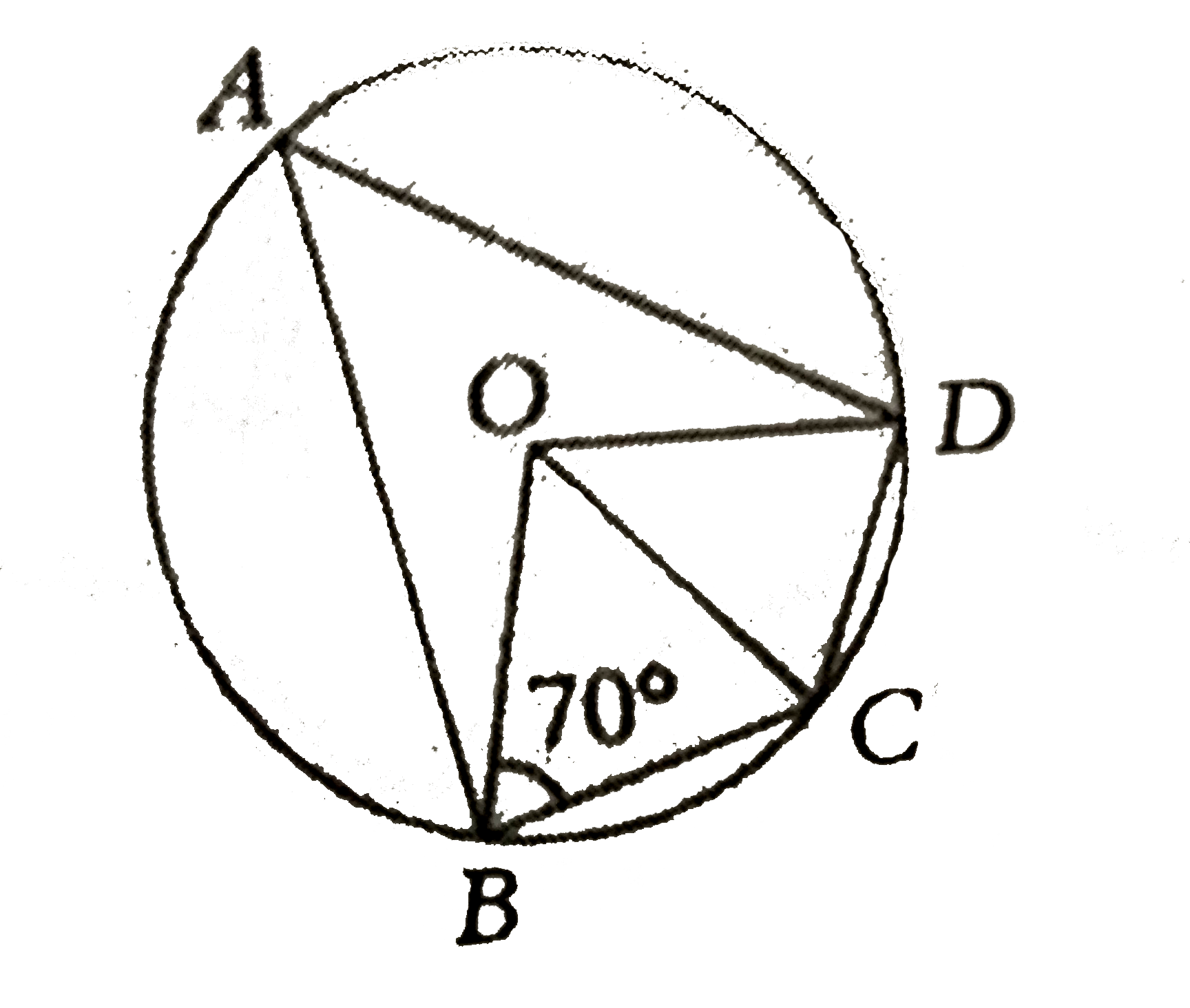
In the above figure (not to scale), O is the centre of the circle. ¯¯¯¯¯¯BC and ¯¯¯¯¯¯CD are equal chords. If ∠OBC=70∘ , then find ∠BAD.A40∘B60∘C55∘D45∘
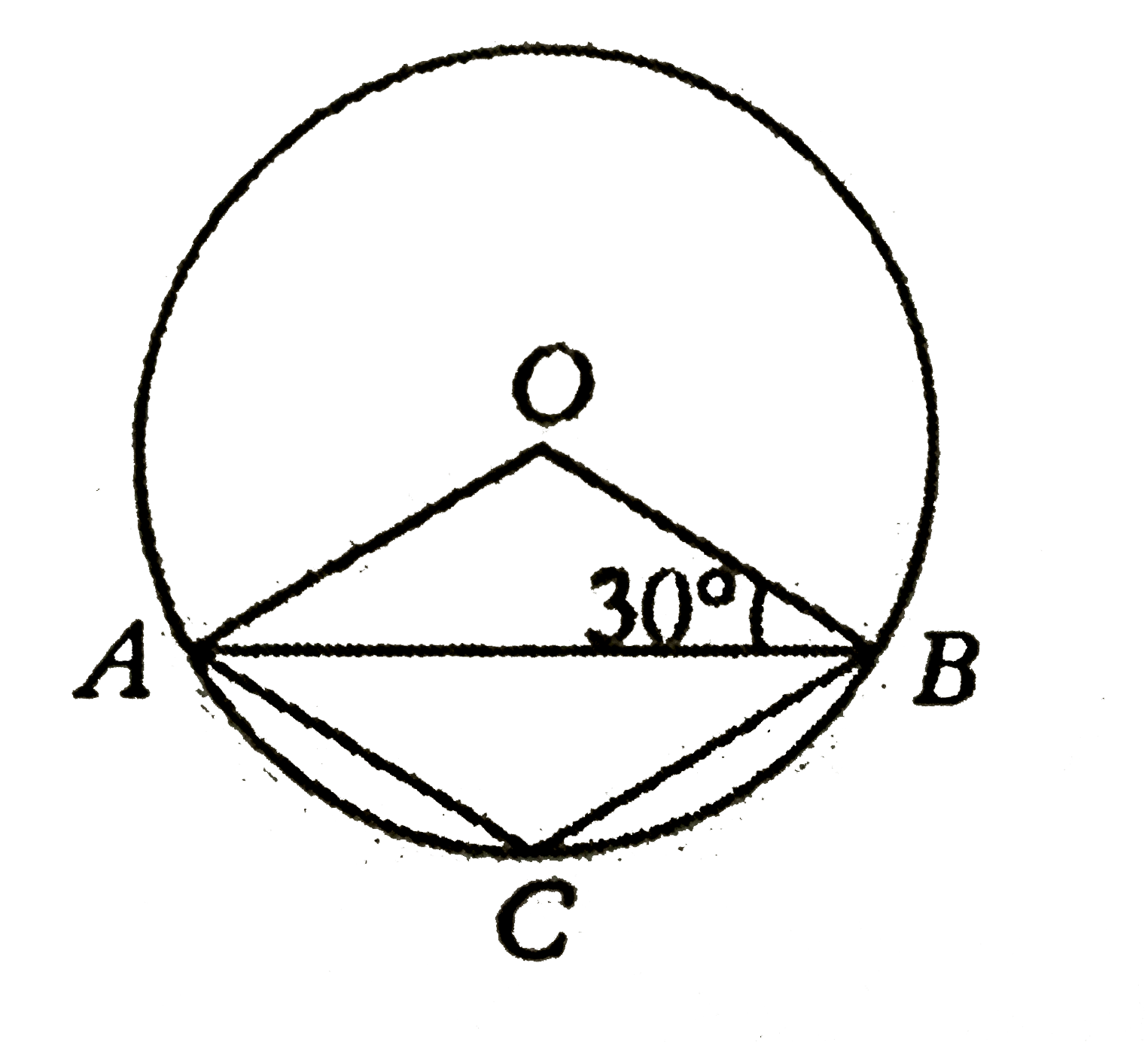
In the figure (not to scale ), O is the centre of the circle and ∠OBA=30∘. Find ∠ACBView SolutionThe position vectors of the points A,B with respect to O are ¯a and ¯b and c is mid point of line segment ¯¯¯¯¯¯AB then ¯¯¯¯¯¯OC=
View SolutionIn the given figure, AB and CD are two equal chords. If O is the centre of the circle , ∠AOB=120∘, then find ∠OCD.
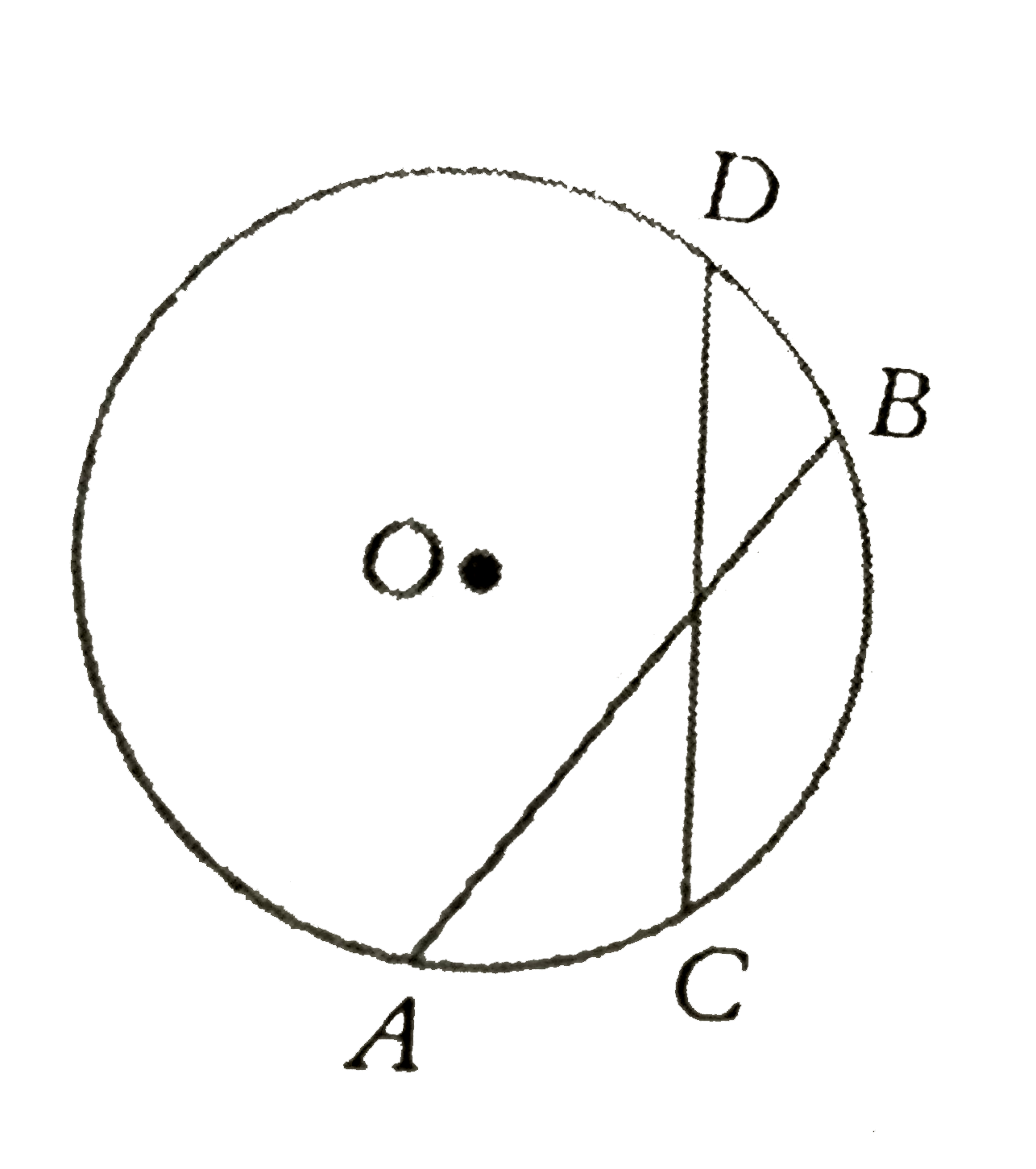 View Solution
View Solution
In the figuare above ( not to scale), ¯¯¯¯¯¯AD is the angle bisector of ∠EAF,∠AFC=110∘ and ∠DCF=20∘. If ∠DAF=30∘ and ∠EBD=10∘, then ∠AEB=View Solution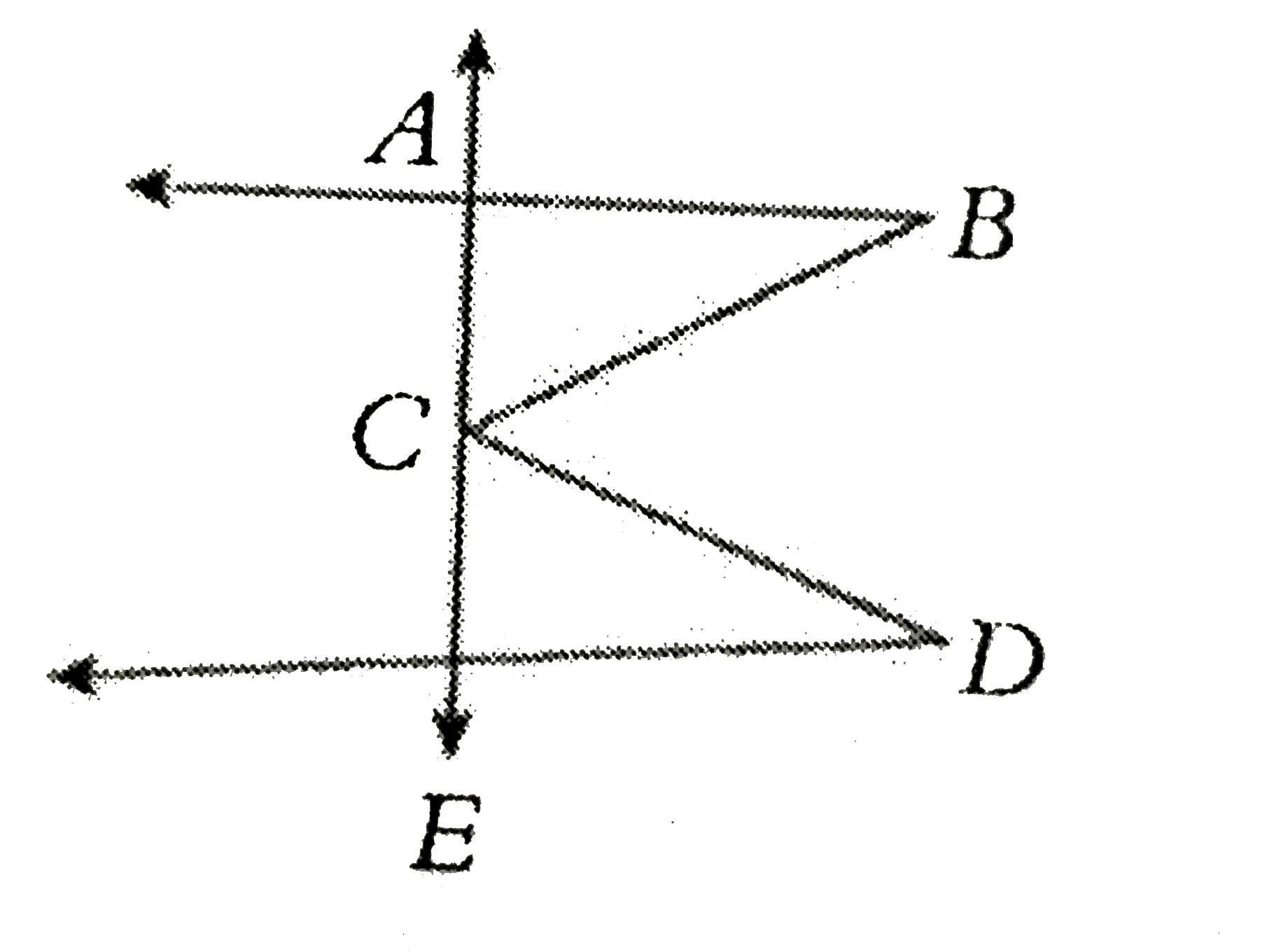
In the above figure, ¯¯¯¯¯¯AB∣∣¯¯¯¯¯¯DE and ACE is a straight line . I f ∠ABC=30∘ and ∠CDE=20∘, then find ∠BCD.View SolutionIn the following figure, O is the centre of the circle and ∠AMB=120∘ , Find the angle between the two tangents AP and BP.
 View Solution
View Solution


