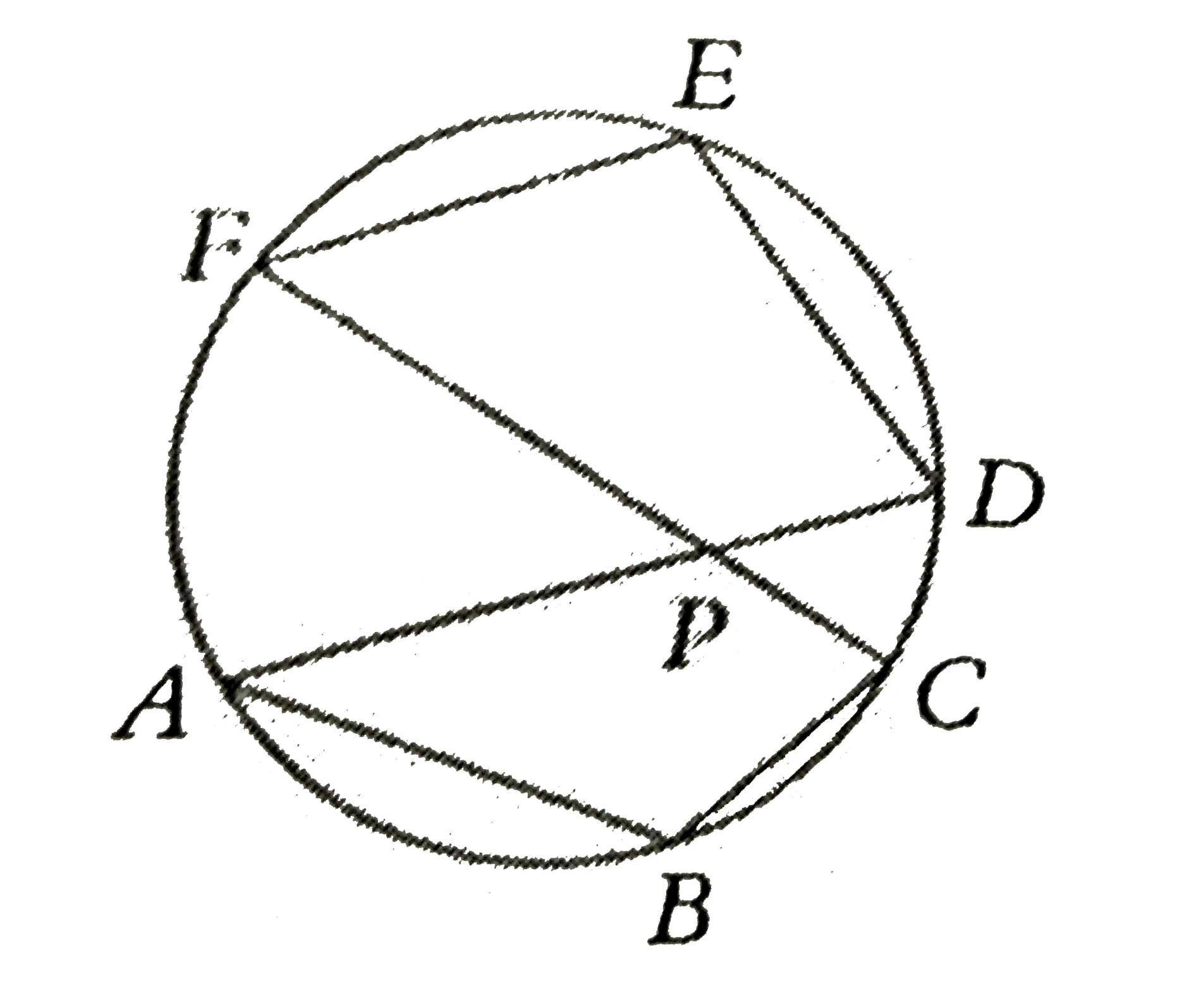
In the above figure (not to scale) ¯¯¯¯¯¯AB,¯¯¯¯¯¯BC,¯¯¯¯¯¯CF,¯¯¯¯¯¯DE and ¯¯¯¯¯¯FE are chords of the circle. If ∠ABC=100∘ and ∠FED=110∘, then ∠FPA=
20∘
30∘
40∘
70∘
The correct Answer is:b
(i) Opposite angles of a cyclic quadrilateral are supplementary.
(ii) Join AF, then ACF and ADEF are cyclic quadrilaterals.
(iii) In a cyclic quadrilateral opposite angles ae supplementary.
(iv) By using the above two statements get the angles PAF and PFA and proceed to get the required angle.
Topper's Solved these Questions
- GEOMETRY BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - GEOMETRY EXERCISE - Level 2
20 Videos
- GEOMETRY BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - GEOMETRY EXERCISE - Level 3
7 Videos
- GEOMETRY BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - GEOMETRY EXERCISE - Essay type Question
3 Videos
- FormulaeBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - FormulaeEXERCISE - Level 3
7 Videos
- INDICESBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - INDICESEXERCISE - CONCEPT APPLICATION (Level 3)
7 Videos
Similar Questions
Knowledge Check
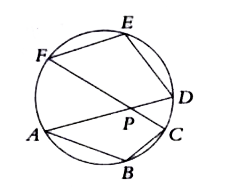
In the above figure (not to scale) ¯¯¯¯¯¯AB,¯¯¯¯¯¯BC,¯¯¯¯¯¯CF,¯¯¯¯¯¯DE,and¯¯¯¯¯¯FE are chords of the circle . If ∠ABC=100∘and∠FED=110∘ , then ∠FPA= _______.A20∘B30∘C40∘D70∘
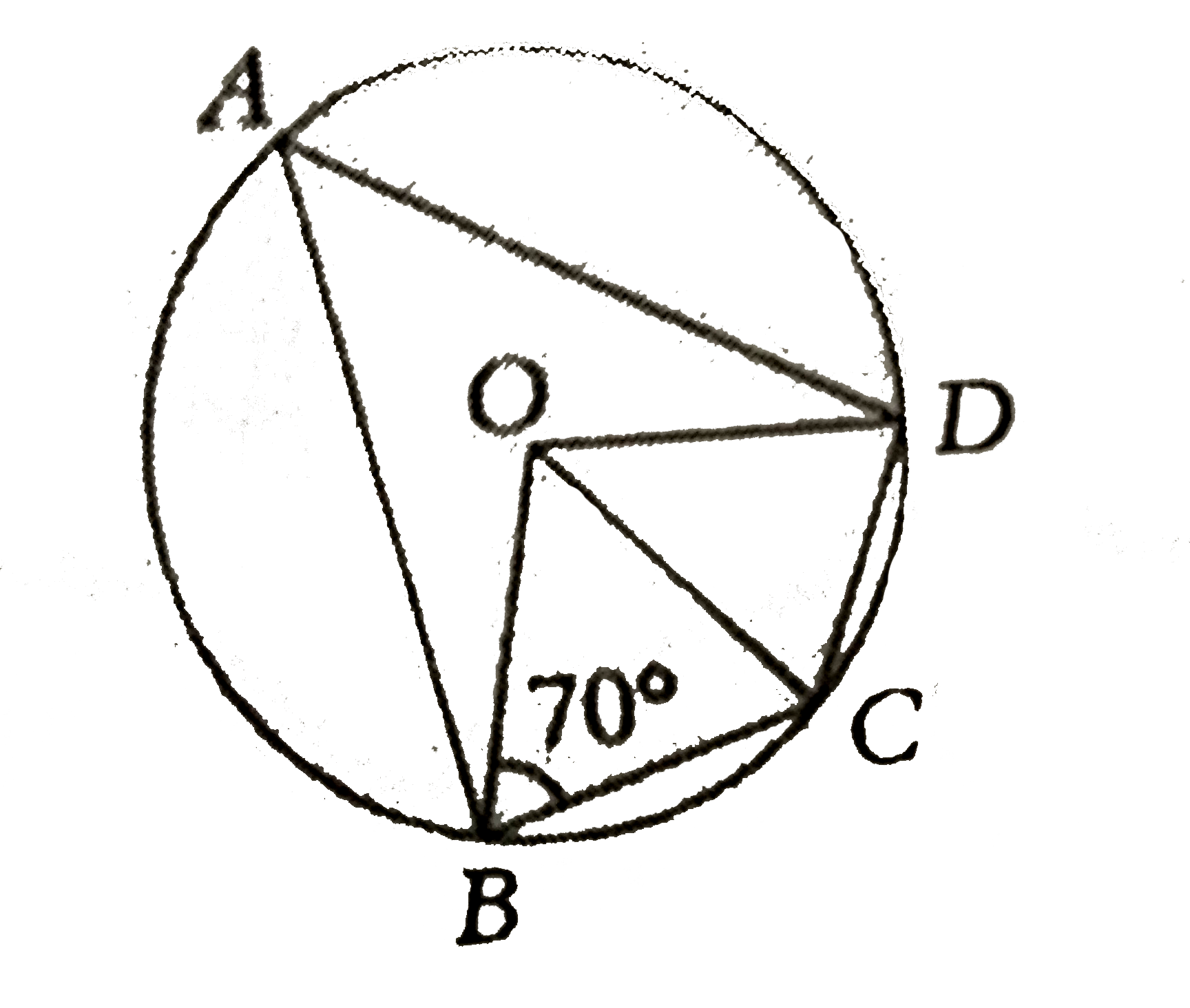
In the above figure (not to scale), O is the centre of the circle. ¯¯¯¯¯¯BC and ¯¯¯¯¯¯CD are equal chords. If ∠OBC=70∘ , then find ∠BAD.
View Solution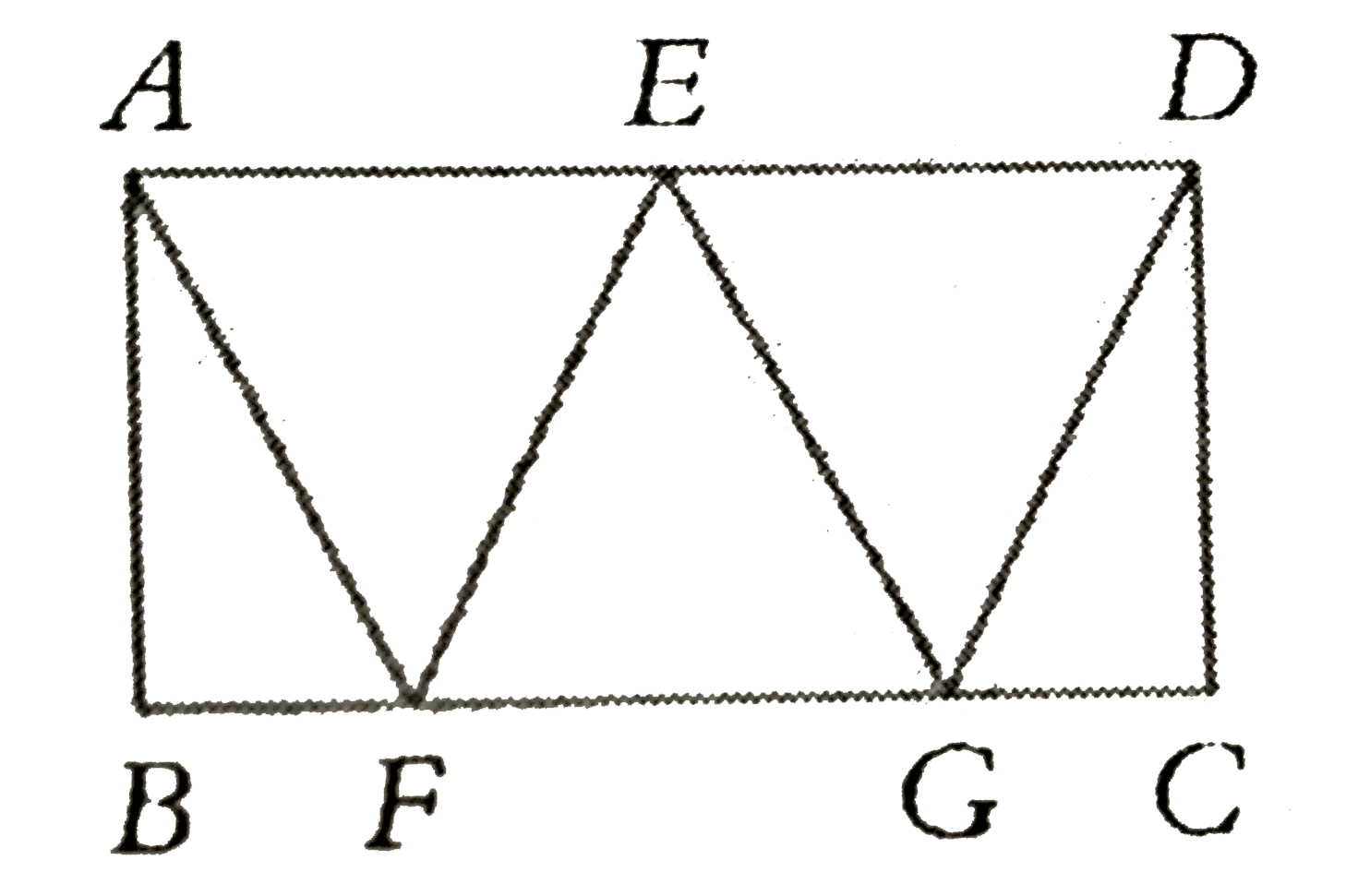
In the figure above (not to scale), ¯¯¯¯¯¯EF∣∣∣∣¯¯¯¯¯¯GD,¯¯¯¯¯¯AF∣∣∣∣¯¯¯¯¯¯EG,¯¯¯¯¯¯AD∣∣¯¯¯¯¯¯BC and ∠DCG=100∘. If ∠CDG=40∘, then find ∠AEF.
View Solution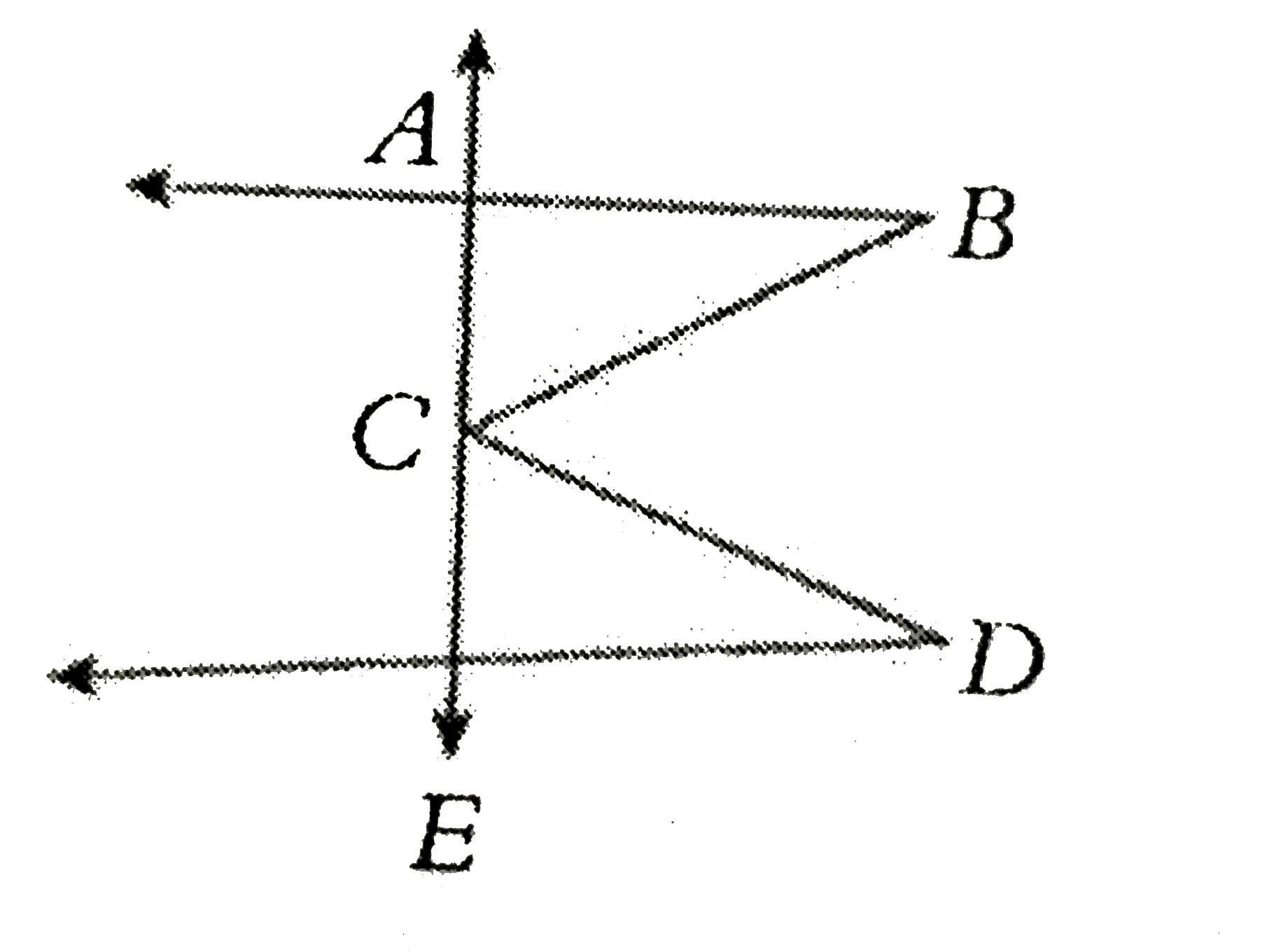
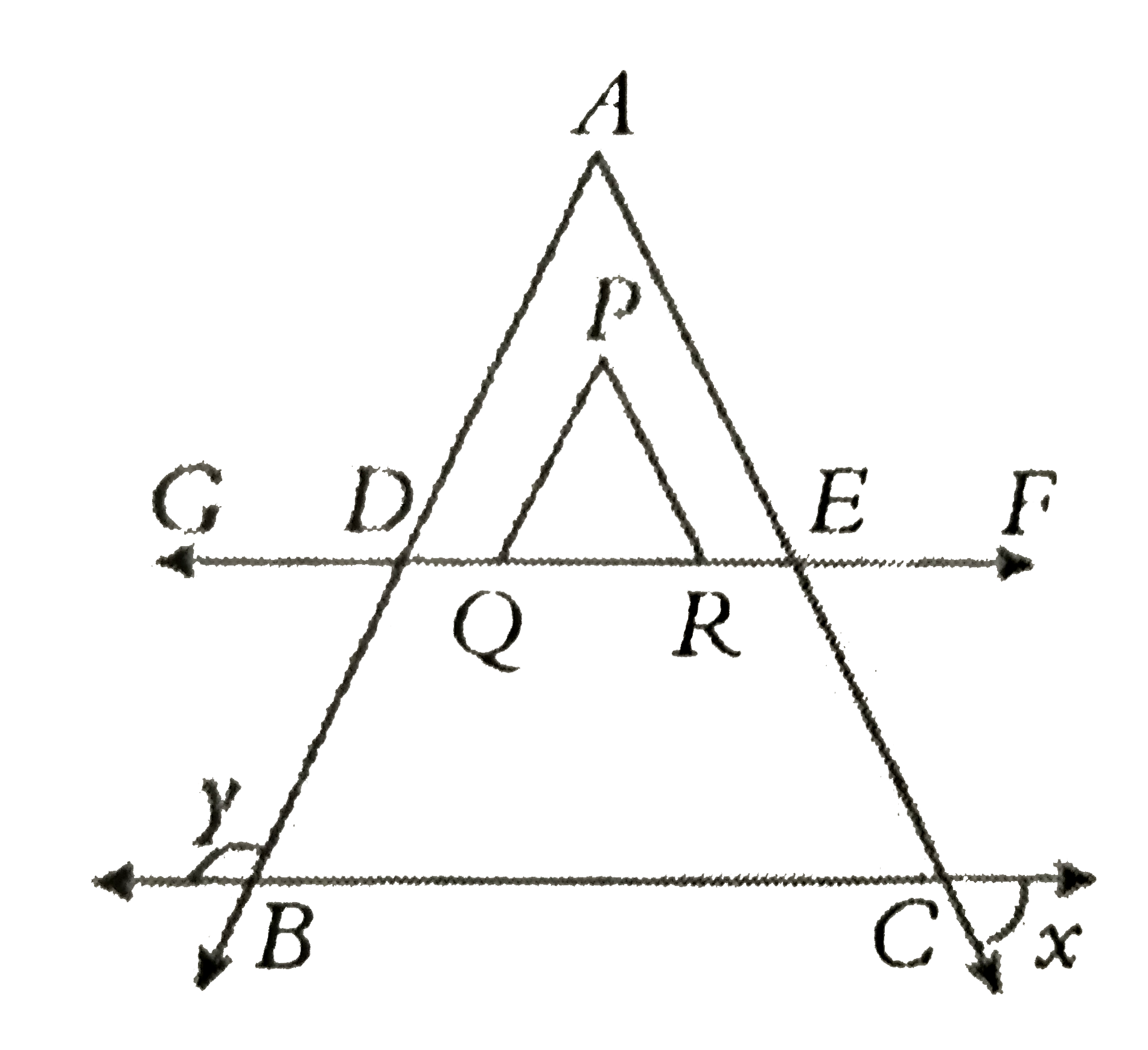
In the above figure, ¯¯¯¯¯¯AB∣∣¯¯¯¯¯¯DE and ACE is a straight line . I f ∠ABC=30∘ and ∠CDE=20∘, then find ∠BCD.
View SolutionFind m∠C in the given figure if ¯¯¯¯¯¯AB∣∣¯¯¯¯¯¯DC.
View SolutionIn the given figure ¯¯¯¯¯¯AB=¯¯¯¯¯¯BC and ¯¯¯¯¯¯AC=¯¯¯¯¯¯CD if ∠BAD=y and ∠ADB=x then ∠y=
View Solution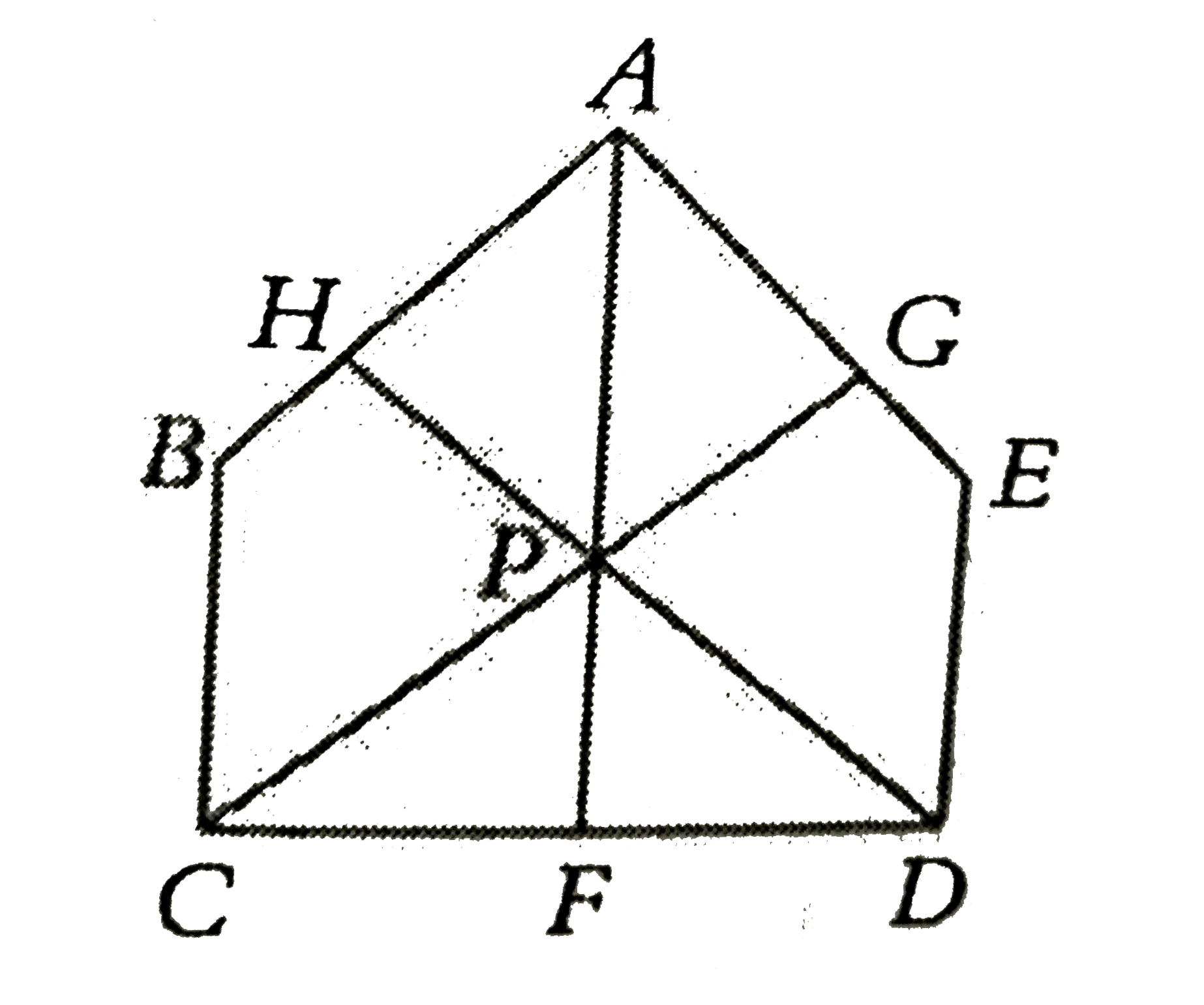
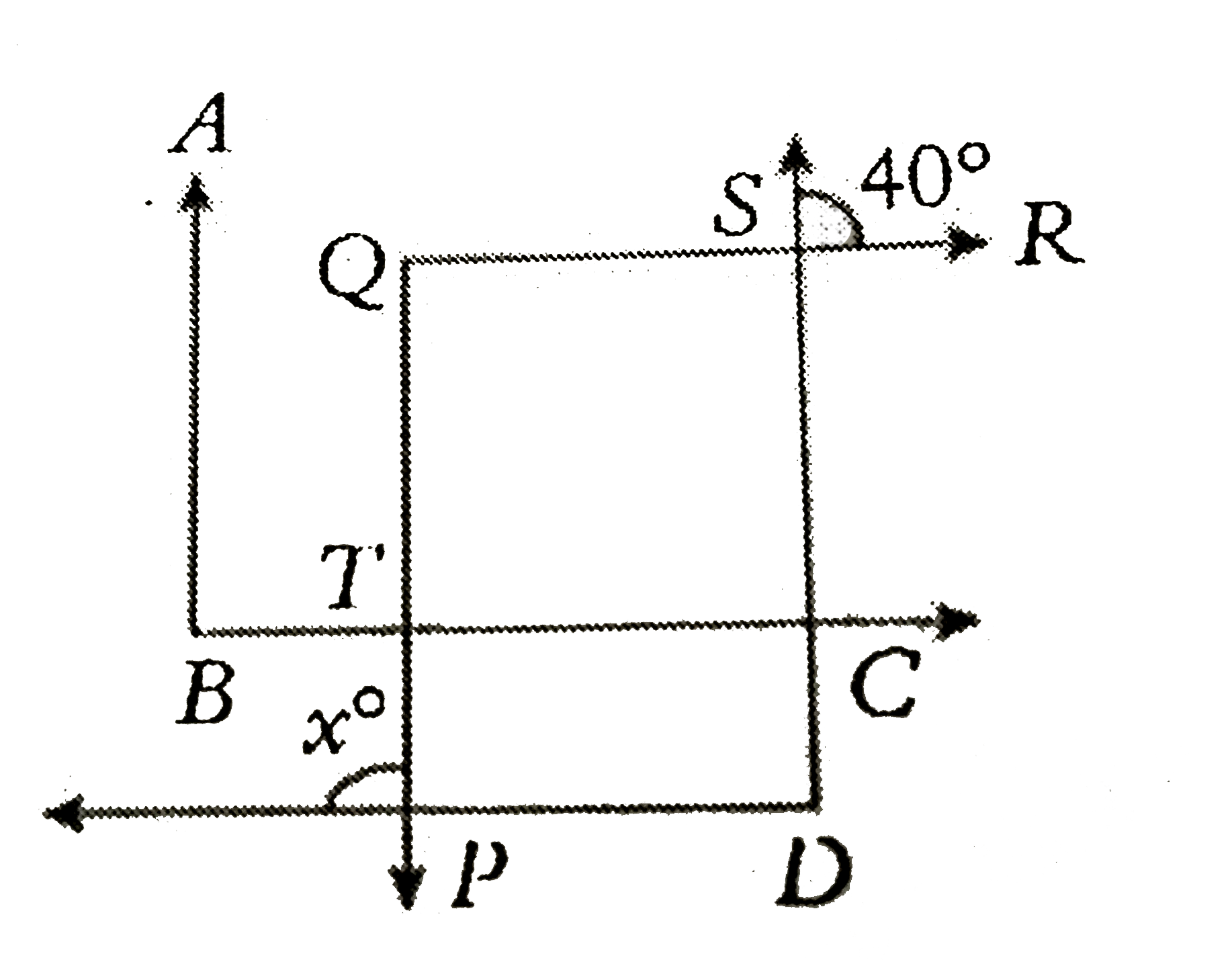
In the above figure, ¯¯¯¯¯¯AF∣∣∣∣¯¯¯¯¯¯ED,¯¯¯¯¯¯CG∣∣∣∣¯¯¯¯¯¯AB and ¯¯¯¯¯¯AE∣∣¯¯¯¯¯¯¯HD If ∠FPD=40∘, then ∠AED=
View SolutionIn the figure below (not to scale), ¯¯¯¯¯¯¯MR⊥¯¯¯¯¯¯¯MP,¯¯¯¯¯¯¯MQ⊥¯¯¯¯¯¯¯¯MN, and ¯¯¯¯¯¯¯MS is bisector of ∠RMQ . If ∠PMN=50∘ , then find the measure of ∠RMS.
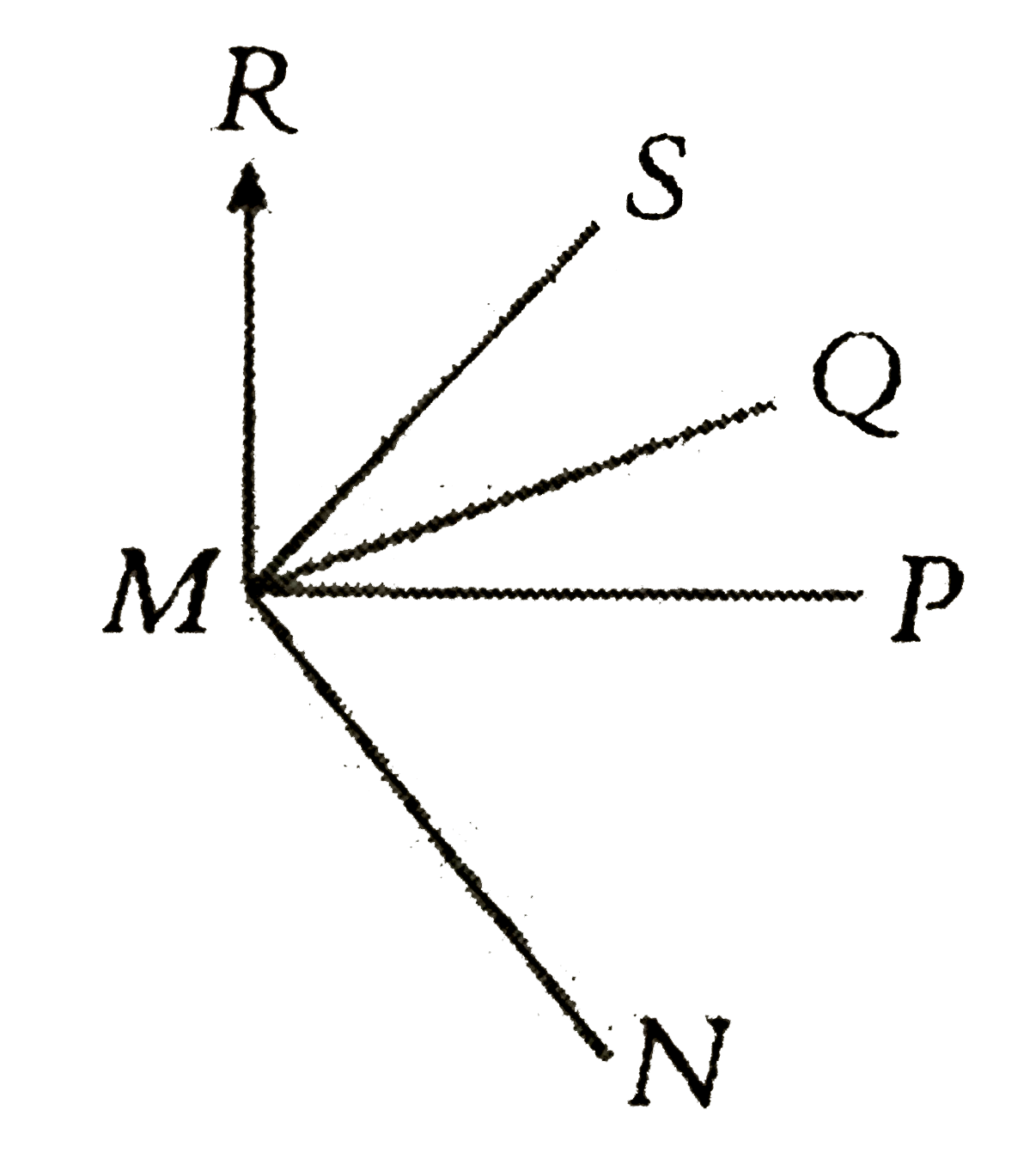
View Solution