Find the area of the region bounded by the limits x=0,x=π2,andf(x)=sinx,g(x)=cosx.
Video Solution
Text Solution
The correct Answer is:2(√2−1) sq. units
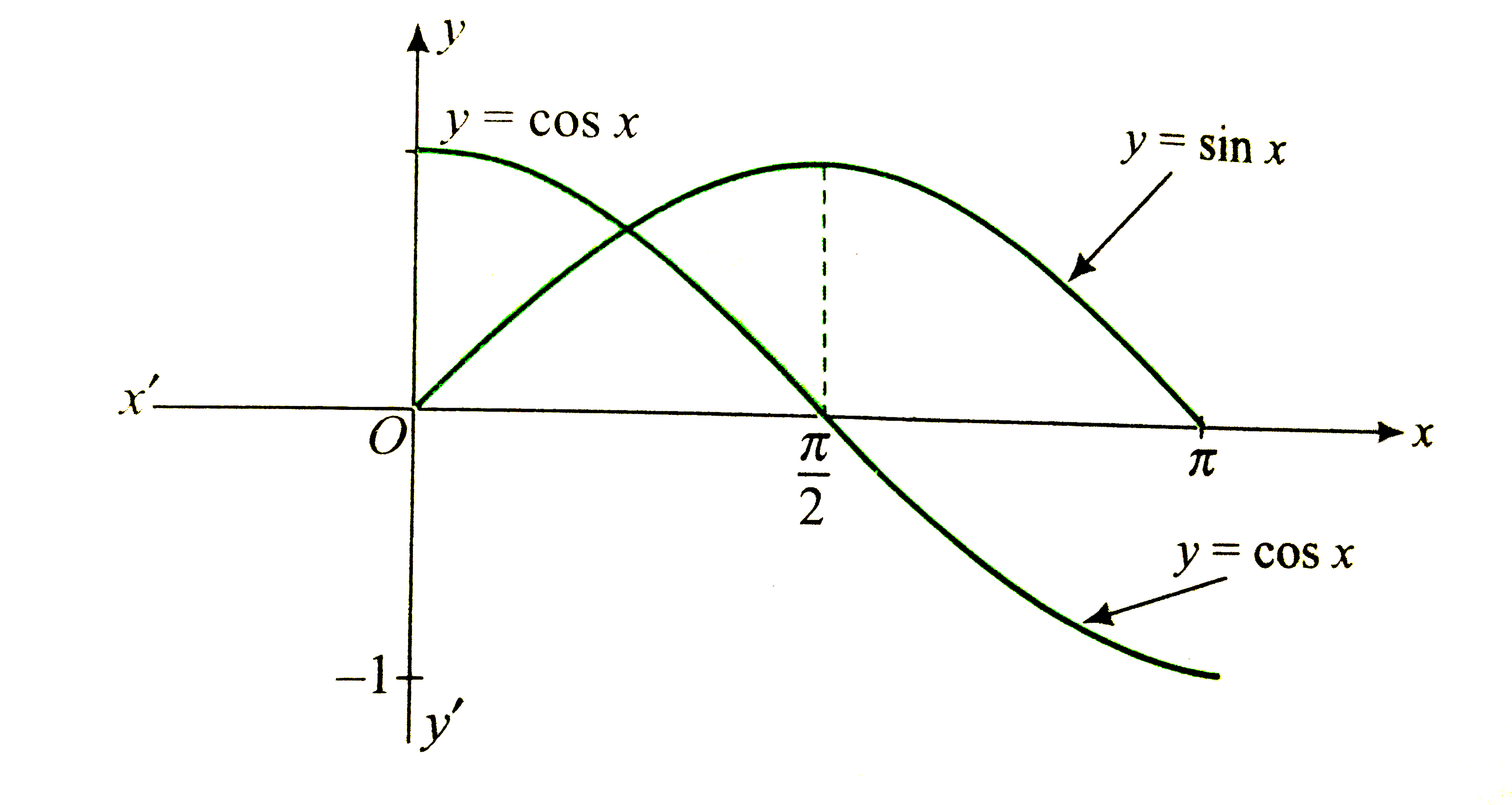
Required area =∫π/20|sinx−cosx|dx
=2∫π/40(cosx−sinx)dx
=2|sinx+cosx|π/40
=2(1√2+1√2−1)=2(√2−1) sq. units.
|
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - CENGAGECHAPTER - AREAEXERCISE - Exercise 9.37videos
AREA
- BOOK - CENGAGECHAPTER - AREAEXERCISE - Exercise (Single)40videos
AREA
- BOOK - CENGAGECHAPTER - AREAEXERCISE - Exercise 9.19videos
AREA
- BOOK - CENGAGECHAPTER - APPLICATIONS OF DERIVATIVESEXERCISE - Question Bank29videos
APPLICATIONS OF DERIVATIVES
- BOOK - CENGAGECHAPTER - AREA UNDER CURVESEXERCISE - Question Bank20videos
AREA UNDER CURVES
Similar Questions
Find the area of the region bounded by the limits x=0,x=π2,andf(x)=sinx,g(x)=cosx.
View SolutionFind the area of the region bounded by y=√xandy=x.
View SolutionFind the area of the region bounded by the curves y=x2+2, y=x,x=0andx=3.
View SolutionFind the area of the region bounded by the curves y=x2+2y=x,x=0,andx=3.
View SolutionFind the area of the region bounded by the parabola y=x2 and y=|x|.
View SolutionFind the area of the region bounded by the parabola y=x2 and y=|x| .
View SolutionFind the area of the region bounded by the curves y=x2+2;y=x;x=0 and x=3
View SolutionFind the area of the region bounded by the curve y=x3,y=x+6 and x=0
View SolutionFind the area of the region bounded by the curves y=x2+2,y=x,x=0 and x=3.
View SolutionFind the area of the region bounded by y=x2+1,y=x,x=0 and y=2.
View Solution

