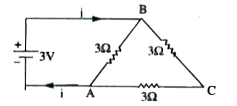
(a) Calculate I1andI2 in terms of I as shown in Fig(i)
(b) Calculate V1andV2 in terms of V as shown in Fig (ii).
Text Solution
(a) Current distribution in parallel Using junction law we can write
I=I1+I2 …(i)
R1andR2 are connected in parallel, hence the potential difference must be the same for both.
I1R1=I2R2⇒I2=I1R1R2 ...(ii)
On substituting I2 from equation (ii) in equation (i)
I1+I1R1R2=I
⇒I1=R2R1+R2I
Substituting I1 in equation (ii)
⇒I2=R1R1+R2I
The current is distributed inversely proportional to resistances in parallel
(b) Voltage distribution in series Let I be the common current flowing through both resistors. Voltage is distributed between two resistors connected in series
V=V1+V2 ...(iii)
I=VR1+R2 ..(iv)
V1=IR1=VR1R1+R2
V2=IR2=VR2R1+R2
The potential difference is distributed directly proportional to resistances in series.
|
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - MODERN PUBLICATIONCHAPTER - CURRENT ELECTRICITYEXERCISE - NCERT SOLVED (NCERT Textbook Exercises)
13 Videos
- CURRENT ELECTRICITYBOOK - MODERN PUBLICATIONCHAPTER - CURRENT ELECTRICITYEXERCISE - NCERT SOLVED (NCERT Additional Exercises)
11 Videos
- CURRENT ELECTRICITYBOOK - MODERN PUBLICATIONCHAPTER - CURRENT ELECTRICITYEXERCISE - CONCEPTUAL QUESTIONS
35 Videos
- ATOMS BOOK - MODERN PUBLICATIONCHAPTER - ATOMS EXERCISE - Chapter Practice Test
16 Videos
- DUAL NATURE OF RADIATION AND MATTER BOOK - MODERN PUBLICATIONCHAPTER - DUAL NATURE OF RADIATION AND MATTER EXERCISE - CHAPTER PRACTICE TEST
15 Videos
Similar Questions
Knowledge Check
Current I1andI2 flow in the wires shown in Fig. The field is zero at distance x to the right of O. Then
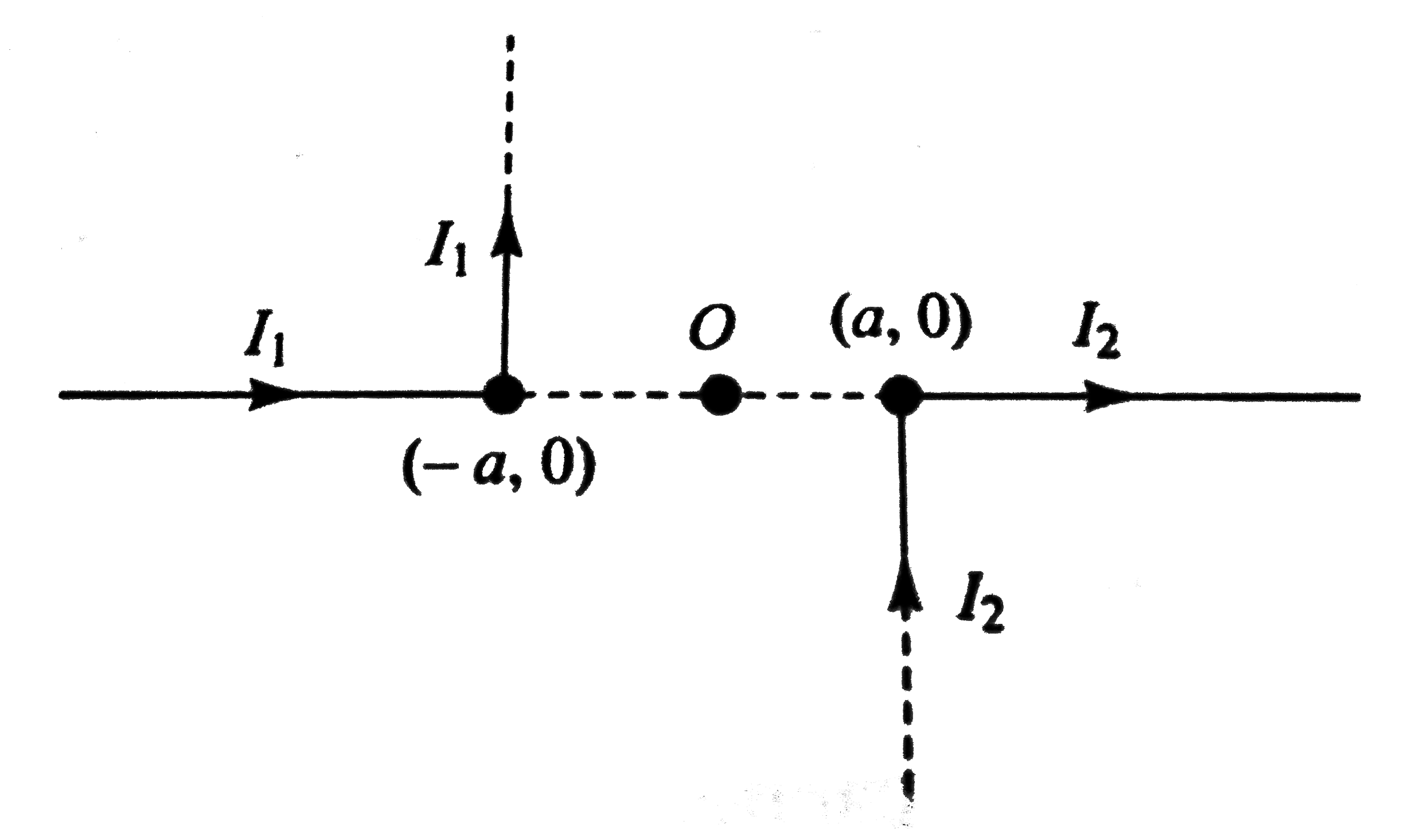 Ax=(I1I2)aBx=(I2I1)aCx=(I1−I2I1+I2)aDx=(I1+I2I1−I2)a
Ax=(I1I2)aBx=(I2I1)aCx=(I1−I2I1+I2)aDx=(I1+I2I1−I2)a
Calculate the magnitude of the magnetic field at point P as shown in Fig, in terms of R,I1andI2.

View SolutionCalculate the magnitude of the magnetic field at point P as shown in Fig, in terms of R,I1andI2.

View SolutionIn the circuit shown in fig., the value of I1+I2 is
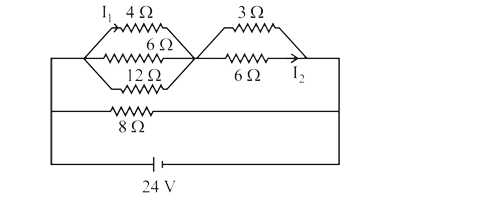
View SolutionIn the circuit shown in fig., the value of I1+I2 is

View SolutionIn the circuit shown in fig., the value of I1+I2 is

View SolutionCalculate the strength of 20 V of H2O2 in terms of
(i) normality
View SolutionCalculate the values of currents ·I1I2I3andI4 Is and loin the section of networks shown in the figure
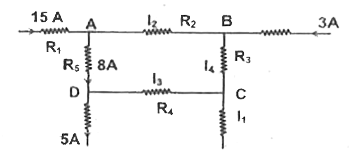
View Solution