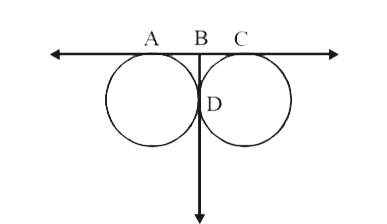
In the given figure, BD=DC and ∠CBD=30∘, find ∠BAC.
Video Solution
Text Solution
The correct Answer is:∠BAC=60∘
BD=DC⇒∠BCD=∠CBD=30∘
∠BDC=180∘−(30∘+30∘)=120∘
∠BAC+∠BDC=180∘⇒∠BAC=60∘
|
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - RS AGGARWALCHAPTER - CIRCLES EXERCISE - Multiple Choice Questions (Mcq)5videos
CIRCLES
- BOOK - RS AGGARWALCHAPTER - CIRCLES EXERCISE - MULTIPLE CHOICE QUESTION(MCQ)37videos
CIRCLES
- BOOK - RS AGGARWALCHAPTER - CIRCLES EXERCISE - EXERCISE 12B18videos
CIRCLES
- BOOK - RS AGGARWALCHAPTER - BAR GRAPH, HISTOGRAM AND FREQUENCY POLYGONEXERCISE - EXERCISE 17B16videos
BAR GRAPH, HISTOGRAM AND FREQUENCY POLYGON
- BOOK - RS AGGARWALCHAPTER - CONGRUENCE OF TRIANGLES AND INEQUALITIES IN A TRIANGLEEXERCISE - Multiple Choice Questions (Mcq)20videos
CONGRUENCE OF TRIANGLES AND INEQUALITIES IN A TRIANGLE
Similar Questions
Knowledge Check
- Question 1 - Select One
In the given figure, find the value of BD.
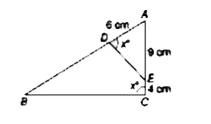 A13.5 cmB11 cmC3.5 cmD9.5 cm
A13.5 cmB11 cmC3.5 cmD9.5 cm
In the given figure, prove that AC=BD.
 View Solution
View SolutionIn Figure, AB=ACand∠ACD=10∘, find ∠BAC
 View Solution
View SolutionIn Figure, if ∠BAC=600 and ∠BCA=200, find ∠ADC
View Solutionin the given triangle, BD=AD=CD then ∠BAC=
View SolutionIn the given figure if ∠DBC=70∘ and ∠BAC=40∘ then find the value of ∠BCD
View SolutionIn Figure, BD=DC and ∠DBC=250 find the measure of ∠BAC
View SolutionIn Figure, if AB DE, ∠BAC=350and ∠CDE=530, find ∠DCE.
View Solution