ABCD is trapezium in which AB∣∣DC, DC = 30 cm and AB = 50 cm. If X and Y are, respectively the mid-points of AD and BC, prove that
ar(DCYX)=79ar(XYBA).
Given In a trapezium ABCD, AB∣∣DC, DC = 30 cm and AB = 50 cm.
Also, X and Y are respectively the mid-points of AD and BC.
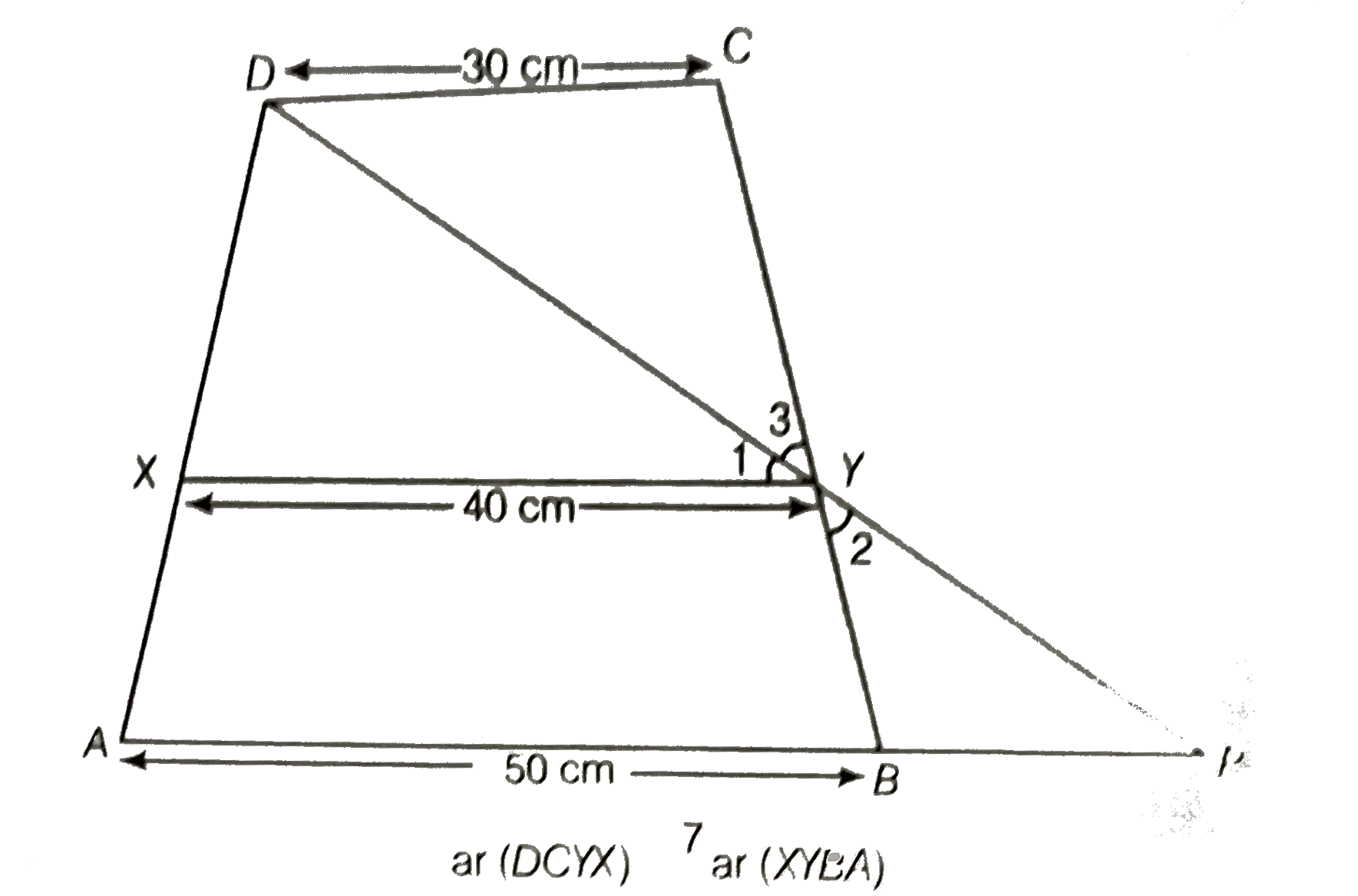
To prove ar(DCYX)=79ar(XYBA)
Construction Join DY and extend it to meet produced AB at P.
Proof In ΔDCY and ΔPBY,
CY=BY [since, Y is the mid-point of BC]
∠DCY=∠PBY [alternate interior angles]
and ∠2=∠3 [vertically opposite angles]
∴ ΔDCY≅PBY [by ASA congruence rule]
Then, DC=BP [by CPCT]
But DC=30cm [given]
∴ DC=BP=30cm
Now, AP=AB+BP
=50+30=80cm
In ΔADP, by mid-point theorem,
XY=12AP=12×80=40cm
Let distance between AB, XY and XY, DC is h cm.
Now, area of trapezium DCYX=12h(30+40)
[∵area of trapezium =12sum of parallel sides×distance between them]
=12h(70)=35hcm2
Similarly, area of trapezium=12h(40+50)=12h×90=45hcm2
∴ ar(DCYX)ar(XYBA)=35h45h=79
⇒ ar(DCYX)=79ar(XYBA) Hence proved.
Topper's Solved these Questions
Similar Questions
ABCD is a square. E and F are respectively the mid-points of BC and CD. If R is the mid-point of EF, prove that ar(ΔAER)=ar(ΔAFR).
 View Solution
View SolutionIn a trapezium ABCD, AB || DC, AB = a cm, and DC = b cm. If M and N are the midpoints of the nonparallel sides, AD and BC respectively then find the ratio of ar(DCNM) and ar(MNBA).
View SolutionABCD is a trapezium in which AB∣∣DCand∠A=∠B=45∘. Find angles C and D of the trapezium.
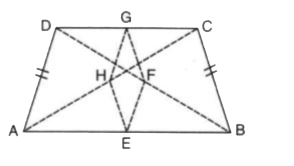
View SolutionABCD is a quadrilateral in which AD = BC. E, F, G and H are the mid-points of AB, BD, CD and AC respectively. Prove that EFGH is a rhombus.
 View Solution
View SolutionABCD is a square, X is the mid-point of AB and Y the mid-point of BC. Prove that
∠DXA=∠AYBView SolutionIn trapezium ABCD, sides AB and DC are parallel to each other. E is mid-point of AD and F is mid-point of BC.
Prove that : AB + DC = 2EF.View SolutionConstruct a triangle ABC in which AC=5cm, BC= 7 cm and AB= 6cm.
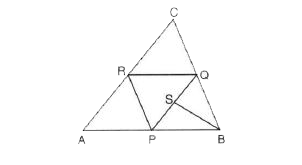
Mark D, the mid-point of ABView SolutionThe following figure shows a triangle ABC in which P, Q and R are mid-points of sides AB, BC and CA respectively. S is mid-point of PO.
Prove that : ar.(ΔABC)=8×ar.(ΔQSB)
 View Solution
View SolutionIn ΔABC , if L and M are the points on AB and AC, respectively such that LM∣∣BC. Prove that ar(ΔLOB)=ar(ΔMOC).
View SolutionABCD is a trapezium with AB || DC. E and F are points on non-parallel sides AD and BC respectively such that EF is parallel to AB. Show thatAEED=BFFC.
View Solution

