A bucket is being lowered down into a well through a rope passing over a fixed pulley of radius 10 cm. Assume that the rope does not slip on the pulley. Find the angular velocity and angular acceleration of the pulley at an instant when the bucket is going down at at speed of 20 cm/s and has an acceleration of 4.0ms2.
To solve the problem, we need to find the angular velocity and angular acceleration of the pulley when the bucket is being lowered.
1. Identify Given Values:
- Radius of the pulley, R=10cm=0.1m
- Linear speed of the bucket, v=20cm/s=0.2m/s
- Linear acceleration of the bucket, a=4m/s2
2. Calculate Angular Velocity (ω):
The relationship between linear velocity (v) and angular velocity (ω) is given by:
ω=vR
Substituting the values:
ω=0.2m/s0.1m=2rad/s
3. Calculate Angular Acceleration (α):
The relationship between linear acceleration (a) and angular acceleration (α) is given by:
α=aR
Substituting the values:
α=4m/s20.1m=40rad/s2
4. Final Answers:
- Angular velocity of the pulley, ω=2rad/s
- Angular acceleration of the pulley, α=40rad/s2
Summary:
The angular velocity of the pulley is 2rad/s and the angular acceleration is 40rad/s2.
- Radius of the pulley, R=10cm=0.1m
- Linear speed of the bucket, v=20cm/s=0.2m/s
- Linear acceleration of the bucket, a=4m/s2
The relationship between linear velocity (v) and angular velocity (ω) is given by:
ω=vR
Substituting the values:
ω=0.2m/s0.1m=2rad/s
The relationship between linear acceleration (a) and angular acceleration (α) is given by:
α=aR
Substituting the values:
α=4m/s20.1m=40rad/s2
- Angular velocity of the pulley, ω=2rad/s
- Angular acceleration of the pulley, α=40rad/s2
The angular velocity of the pulley is 2rad/s and the angular acceleration is 40rad/s2.
Topper's Solved these Questions
- BOOK - HC VERMA ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - worked out Examples29videos
ROTATIONAL MECHANICS
- BOOK - HC VERMA ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - Objective -126videos
ROTATIONAL MECHANICS
- BOOK - HC VERMA ENGLISHCHAPTER - REST AND MOTION : KINEMATICSEXERCISE - Question for short Answer13videos
REST AND MOTION : KINEMATICS
- BOOK - HC VERMA ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Question for short Answer15videos
SIMPLE HARMONIC MOTION
Similar Questions
A article moves in a circle of radius 20 cm with linear speed of 10 m/s. Find the angular velocity
View SolutionA block of mass m is attached at the end of an inextensible string which is wound over a rough pulley of mass M and radius R figure a. Assume the string does not slide over the pulley. Find the acceleration of the block when released.
View SolutionCalculate the magnitude of linear acceleration of a particle moving in a circle of radius 0.5 m at the instant when its angular velocity is 2.5 rad s–1 and its angular acceleration is 6rads−2.
View SolutionCalculate the magnitude of linear acceleration of a particle moving in a circle of radius 0.5 m at the instant when its angular velocity is 2.5 rad s–1 and its angular acceleration is 6rads−2.
View SolutionA light rope is passed over a pulley such that at its one end a block is attached , and on the other end a boy is climbing up with acceleration g2 relative to rope. Mass of the block is 30 kg and that of the boy is 40 kg. Find the tension and acceleration of the rope .
View SolutionTwo masses m1 and m2 are joined with the help of massless string passing over a pulley of Radius R and mass M. Assume that string doesn't slip over the pulley. Take m1=10kg, m2=5kg, M=10kg, R=1m.
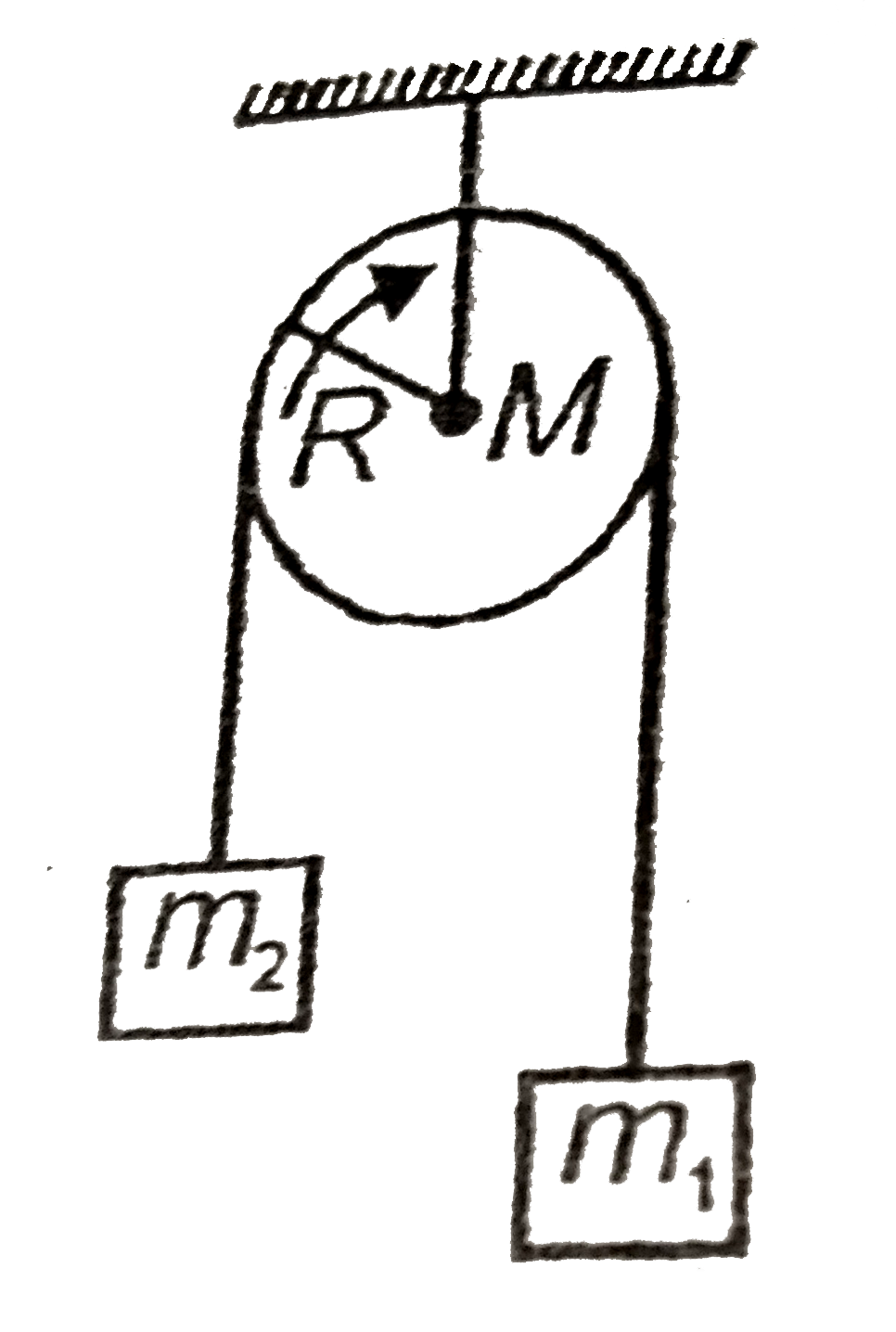
(a) Calculate the acceleration of each book.
(b) Calculate the tensions in the thread.View SolutionA 2 kg block A is attached to one end of a light string that passes over an an ideal pulley and a 1 kg sleeve B slides down the other part of the string with an acceleration of 5m/s2 with respect to the string. Find the acceleration of the block, acceleration of sleeve and tension in the steing. [g=10m/s2]
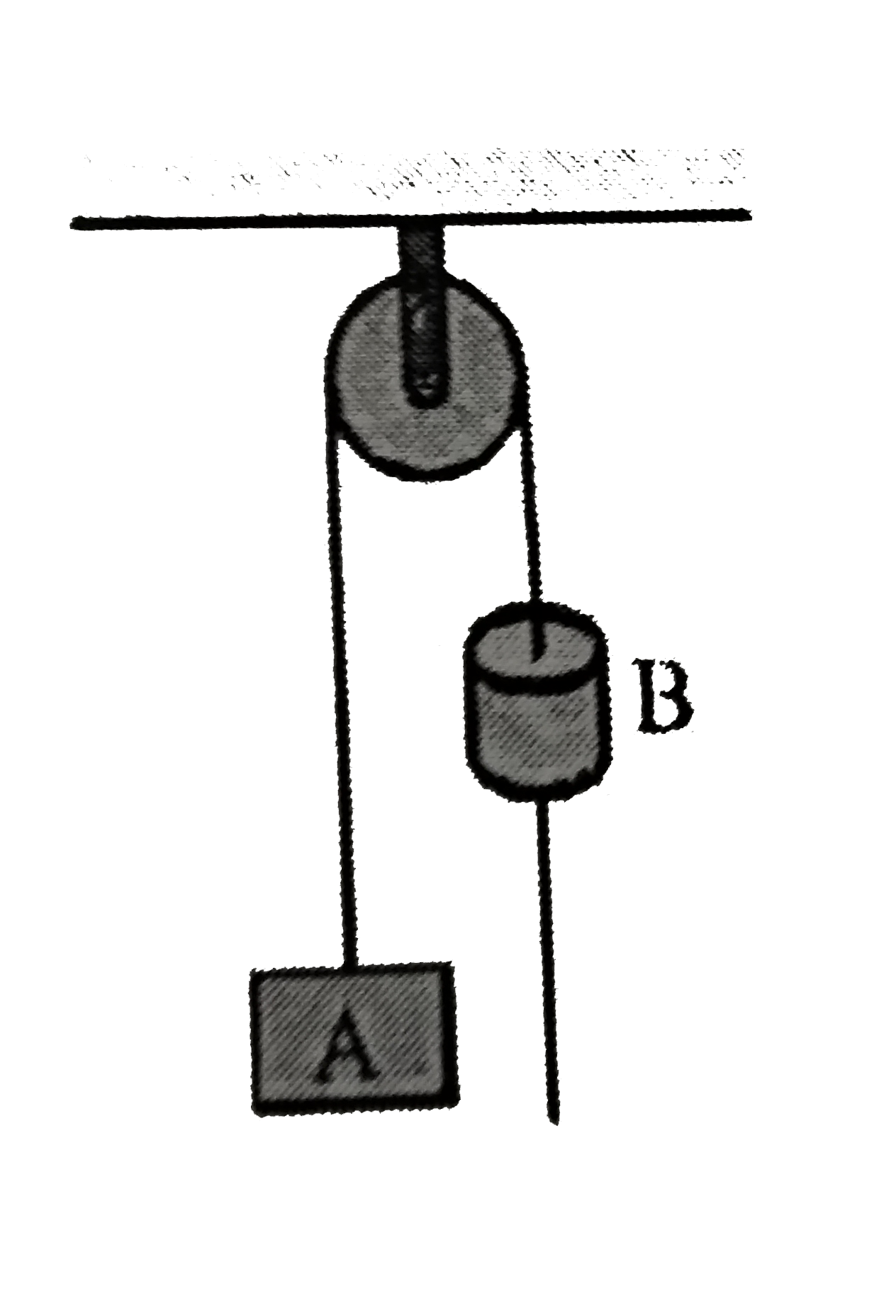 View Solution
View SolutionTwo blocks A and B having masses 5 kg and 10 kg respectively connected through a massless string which is passing over a pulley (disc) of mass 2 kg and radius 25 cm as shown in figure If system is. released from rest. then angular acceleration of the pulley is (String does not slip over the pulley horizontal surface is smooth and g=10ms−2 (∀KTST02_N∃TPHYE02011_Q01)
View SolutionA block hangs from a string wrapped on a disc of radius 20 cm free to rotate about its axis which is fixed in a horizontal position. If the angular speed of the disc is 10 rad/s at some instant, with what speed is the block going down at that instant?
View SolutionA particle travels in a circle of radius 20 cm at a speed thast uniformly increases. If the speed changes from 5.0 m/s to 6.0 m/s in 2.0s, find the angular aceleration.
View Solution

