Question

Let V and E represent the gravitational potential and field at a distance r from the centre of a uniform solid sphere. Consider the two statements.
A. the plot of V agasinst r is discotinuous
B. The plot of E against r is discontinuous.

Options

A. both A and B are true
B. A is true but B is false
C. B is true but A is false
D. both A and B are false

Answer

D

Explanation

To solve the problem, we need to analyze the gravitational potential (V) and gravitational field (E) at a distance r from the center of a uniform solid sphere. We will consider two cases: when the point is inside the sphere and when it is outside the sphere.

Step 1: Determine the gravitational potential (V)
1. Outside the sphere (r > a): The gravitational potential is given by the formula:
\( V = -\frac{GM}{r} \)
where G is the gravitational constant, M is the mass of the sphere, and r is the distance from the center of the sphere.

2. Inside the sphere (r < a): The gravitational potential is given by:
\( V = -\frac{GM}{2a} + \frac{GM}{a^3} r^2 \)
where a is the radius of the sphere.

Step 2: Analyze the continuity of the potential (V)
- At the surface of the sphere (r = a), we can evaluate both expressions for V:
- For r = a (outside):
\( V = -\frac{GM}{a} \)
- For r = a (inside):
\( V = -\frac{GM}{2a} + \frac{GM}{a^3} a^2 = -\frac{GM}{2a} + \frac{GM}{2a} = -\frac{GM}{2a} \)
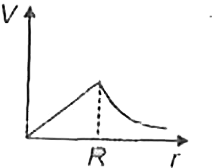
- Since the values of V at r = a are different for inside and outside, the plot of V against r is discontinuous at r = a.

Step 3: Determine the gravitational field (E)
1. Outside the sphere (r > a): The gravitational field is given by:
\( E = \frac{GM}{r^2} \)

2. Inside the sphere (r < a): The gravitational field is given by:
\( E = \frac{GM}{a^3} r \)

Step 4: Analyze the continuity of the field (E)
- At the surface of the sphere (r = a), we can evaluate both expressions for E:
- For r = a (outside):
\( E = \frac{GM}{a^2} \)
- For r = a (inside):
\( E = \frac{GM}{a^3} a = \frac{GM}{a^2} \)
- Since the values of E at r = a are the same for inside and outside, the plot of E against r is continuous at r = a.

Conclusion
- Statement A: The plot of V against r is discontinuous. True
- Statement B: The plot of E against r is discontinuous. False

Thus, the correct answer is that statement A is true, and statement B is false.