A water tank is constructed on the top of a building. With what speed will the water come out of a tap 6.0 m below the water level in the tank? Assume steady flow and that the pressure above the water level is equal to the atmospheric pressure
To solve the problem of determining the speed at which water will come out of a tap located 6.0 m below the water level in a tank, we can use Bernoulli's theorem and the principles of fluid mechanics. Here’s a step-by-step solution:
Step 1: Understand the Setup
We have a water tank at a height (H) of 6.0 m above the tap. The pressure above the water level in the tank and at the outlet of the tap is equal to atmospheric pressure.
Step 2: Apply Bernoulli's Equation
According to Bernoulli's theorem, for two points in a fluid flow, we have:
P1ρg+v212g+z1=P2ρg+v222g+z2
where:
- P1 and P2 are the pressures at points 1 (water level in the tank) and 2 (tap outlet).
- v1 and v2 are the velocities of water at points 1 and 2.
- z1 and z2 are the heights at points 1 and 2.
Step 3: Set the Parameters
- Since both points are open to the atmosphere, P1=P2=Patmospheric.
- Let z1=H=6.0m (height of the water level above the tap).
- Let z2=0 (height of the tap).
- We can assume v1 (velocity of water at the tank) is negligible compared to v2 (velocity at the tap).
Step 4: Simplify Bernoulli's Equation
Substituting the known values into Bernoulli's equation:
Patmosphericρg+v212g+H=Patmosphericρg+v222g+0
Cancelling Patmosphericρg from both sides, we get:
v212g+H=v222g
Step 5: Neglect v1
Since v1 is negligible, we can ignore it:
H=v222g
Step 6: Solve for v2
Rearranging the equation gives:
v22=2gH
Taking the square root:
v2=√2gH
Step 7: Substitute Values
Using g=9.81m/s2 and H=6.0m:
v2=√2×9.81×6.0
Calculating this gives:
v2=√117.72≈10.85m/s
Final Answer
The speed at which the water comes out of the tap is approximately 11 m/s.
---
We have a water tank at a height (H) of 6.0 m above the tap. The pressure above the water level in the tank and at the outlet of the tap is equal to atmospheric pressure.
According to Bernoulli's theorem, for two points in a fluid flow, we have:
P1ρg+v212g+z1=P2ρg+v222g+z2
where:
- P1 and P2 are the pressures at points 1 (water level in the tank) and 2 (tap outlet).
- v1 and v2 are the velocities of water at points 1 and 2.
- z1 and z2 are the heights at points 1 and 2.
- Since both points are open to the atmosphere, P1=P2=Patmospheric.
- Let z1=H=6.0m (height of the water level above the tap).
- Let z2=0 (height of the tap).
- We can assume v1 (velocity of water at the tank) is negligible compared to v2 (velocity at the tap).
Substituting the known values into Bernoulli's equation:
Patmosphericρg+v212g+H=Patmosphericρg+v222g+0
Cancelling Patmosphericρg from both sides, we get:
v212g+H=v222g
Since v1 is negligible, we can ignore it:
H=v222g
Rearranging the equation gives:
v22=2gH
Taking the square root:
v2=√2gH
Using g=9.81m/s2 and H=6.0m:
v2=√2×9.81×6.0
Calculating this gives:
v2=√117.72≈10.85m/s
The speed at which the water comes out of the tap is approximately 11 m/s.
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
About floaring in a water tank is carrying a number of large stones. If the stories were unloaded into water what will happen to water level?
AriseBfallCremain unchangedDrise till half the number of stones are unloaded and then begin to fall.
A tank is filled to a height H. The range of water coming out of a hole which is a depth H/4 from the surface of water level is
View SolutionA tank containing water has an orifice in one vertical side. If the centre of orifice is 4.9 m below the surface level in the tank, the velocity of discharge is:
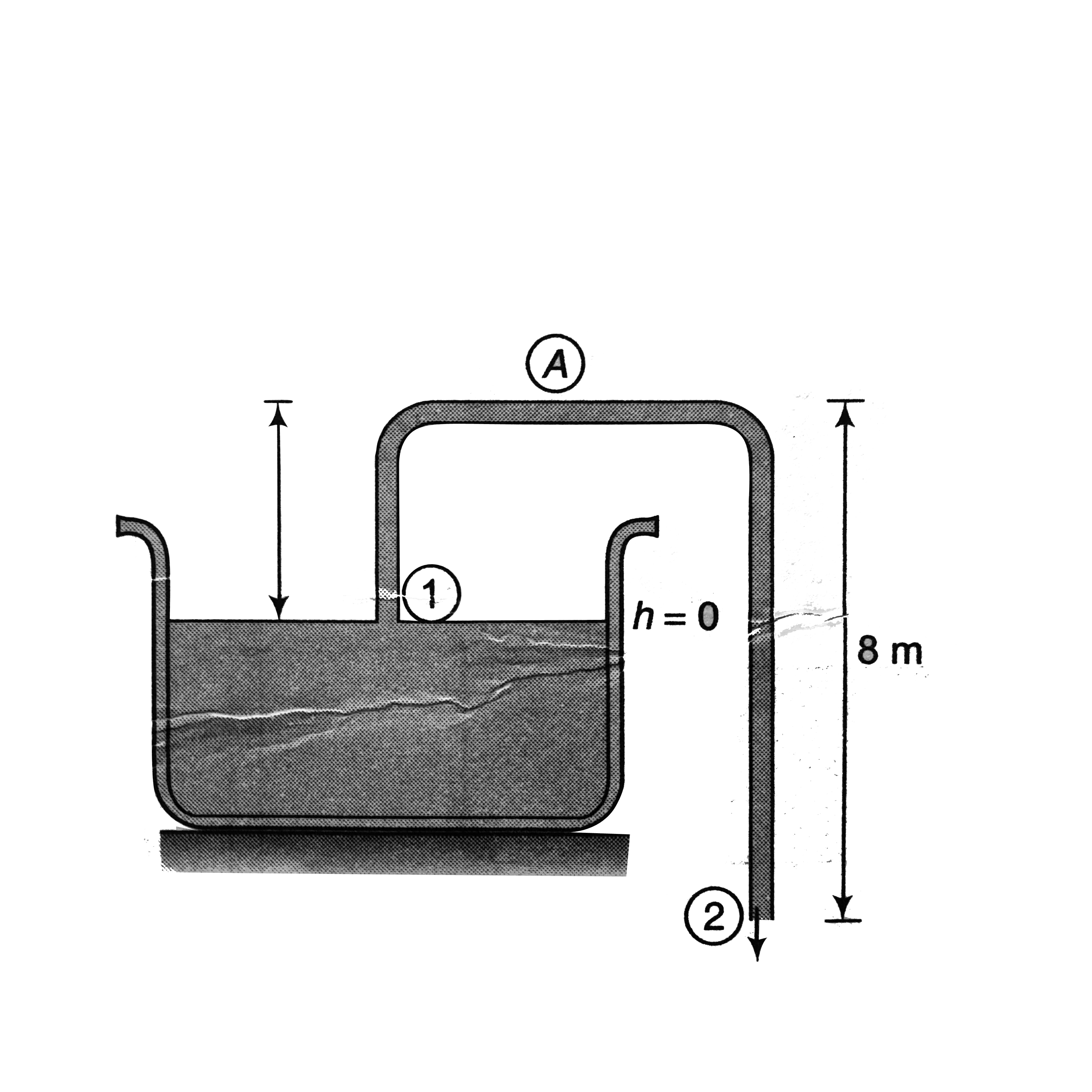
View SolutionThe U-tube acts as a water siphon. The bend in the tube is 1m above the water surface. The tube outlet is 7m below the water surface. The water issues from the bottom of the siphon as a free jet at atmospheric pressure. Determine the speed of the free jet and the minimum absolute pressure of the water in the bend. Given atmospheric pressure =1.01×105N/m2,g=9.8m/s2 and density of water =103kg/m3
 .View Solution
.View SolutionFigure shows a large closed cylindrical tank containing water, Initially the air trapped above the water surface has a height h0and pressure 2p0 where p0is the atmospheric pressure. There is a hole in the wall of tank at a depth h1below the top from which water comes out. A long vertical tube is connected as shown. (a) Find the height h2 of the water in the long tube above the top initially. (b) Find the speed with which water comes out of hole.(c)Find the height of the water in the long tube above the top when the water stops coming out of the hole.
 View Solution
View SolutionA rectangular water tank of base 11m×6m contains water upto a height of 5 m. If the water in the tank is transferred to a cylindrical tank of radius 3.5 m, find the height of the water level in the tank.
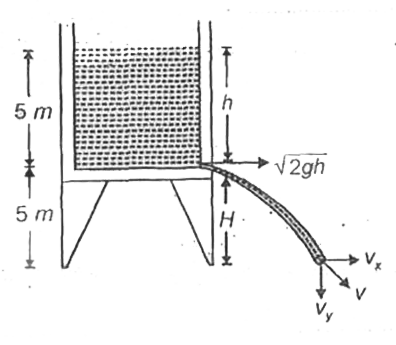
View SolutionA water tank is placed on a platform of height 5 m high and there is an orifice near the bottom in the wall of tank at 5 m below the level of water. Find the speed with which water will hit the ground [Take g = 10 m/s2 ]
 View Solution
View SolutionA rectangular tank is 225 m by 162 m at the base. With what speed must water flow into it through and aperture 60cm by 45cm that the level may be raised 20cm in 5 hours.?
View Solution

