If two capacitors C1 & C2 are connected in parallel then equivalent capacitance is 10F. If both capacitance are connected across 1V battery then energy stored by C2 is 4 times of C1. Then the equivalent capacitance if they are connected in series is-
1.6μF
16μF
4μF
14μF
The correct Answer is:A
To solve the problem step by step, let's break down the information given and apply the relevant formulas.
Step 1: Understand the given information
We have two capacitors, C1 and C2, connected in parallel with an equivalent capacitance of 10F. When connected to a 1V battery, the energy stored in C2 is four times that stored in C1.
Step 2: Write the equation for equivalent capacitance in parallel
For capacitors in parallel, the equivalent capacitance C is given by:
C=C1+C2
From the problem, we know:
C1+C2=10(Equation 1)
Step 3: Write the equation for energy stored in capacitors
The energy U stored in a capacitor is given by:
U=12CV2
For C1:
U1=12C1(12)=12C1
For C2:
U2=12C2(12)=12C2
According to the problem, U2=4U1:
12C2=4(12C1)
This simplifies to:
C2=4C1(Equation 2)
Step 4: Substitute Equation 2 into Equation 1
Now, substitute C2 from Equation 2 into Equation 1:
C1+4C1=10
This simplifies to:
5C1=10
Thus, we find:
C1=2F
Step 5: Calculate C2
Using Equation 2:
C2=4C1=4×2=8F
Step 6: Find the equivalent capacitance in series
For capacitors in series, the equivalent capacitance Ceq is given by:
1Ceq=1C1+1C2
Substituting the values of C1 and C2:
1Ceq=12+18
Finding a common denominator (which is 8):
1Ceq=48+18=58
Thus:
Ceq=85=1.6F
Final Answer
The equivalent capacitance when the capacitors are connected in series is:
1.6F
We have two capacitors, C1 and C2, connected in parallel with an equivalent capacitance of 10F. When connected to a 1V battery, the energy stored in C2 is four times that stored in C1.
For capacitors in parallel, the equivalent capacitance C is given by:
C=C1+C2
From the problem, we know:
C1+C2=10(Equation 1)
The energy U stored in a capacitor is given by:
U=12CV2
For C1:
U1=12C1(12)=12C1
For C2:
U2=12C2(12)=12C2
According to the problem, U2=4U1:
12C2=4(12C1)
This simplifies to:
C2=4C1(Equation 2)
Now, substitute C2 from Equation 2 into Equation 1:
C1+4C1=10
This simplifies to:
5C1=10
Thus, we find:
C1=2F
Using Equation 2:
C2=4C1=4×2=8F
For capacitors in series, the equivalent capacitance Ceq is given by:
1Ceq=1C1+1C2
Substituting the values of C1 and C2:
1Ceq=12+18
Finding a common denominator (which is 8):
1Ceq=48+18=58
Thus:
Ceq=85=1.6F
The equivalent capacitance when the capacitors are connected in series is:
1.6F
Topper's Solved these Questions
Similar Questions
Two capacitors with capacitances C1andC2 are connected in parallel. Find the resultant capacitance.
View SolutionFour capacitors are connected as shown in the figure. The equivalent capacitance between P and Q is
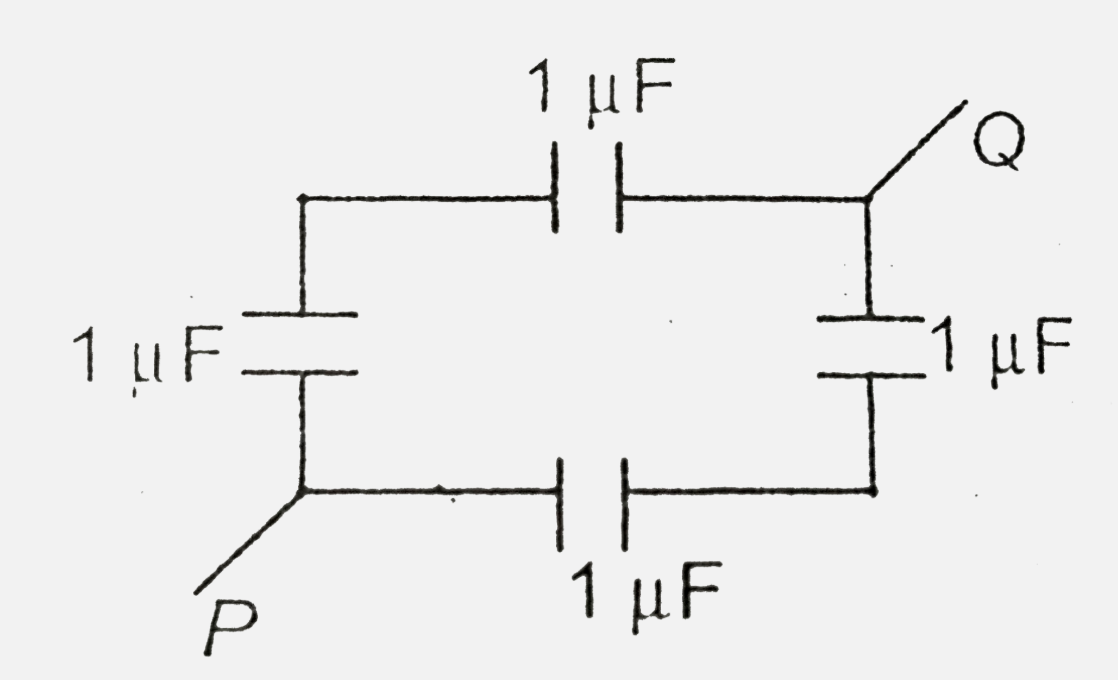
View SolutionFour capacitors are connected as shown . The equivalent capacitance between the points X and Y is

View SolutionTwo capacitors with capacitances C1andC2 are connected in series. Find the resultant capacitance.
View SolutionFind the equivalent capacitance between A and B. All the capacitors have capacitance C.

View SolutionFind the equivalent capacitance between A and B. All the capacitors have capacitance C.

View SolutionThere are 7 identical capacitors. The equivalent capacitance when they are connected in series is C. The equivalent capacitance when they are connected in parallel is
View SolutionEffective capacitance of parallel combination of two capacitors C1andC2 is 10μF. When the capacitors are individually connected to a voltage source of 1V, the energy stored in the capacitor C2 is 4 times of C1. If these capacitors are connected in series, their effective capacitor will be: lt
View SolutionTwo identical capacitors are first connected in series and then in parallel. The ratio of equivalent capacitance is
View SolutionObtain an expression for equivalent capacitance when three capacitors C1,C2andC3 are connected in series.
View Solution
