The charge flowing through a resistance R varies with time t as Q=at−bt2. The total heat produced in R is
a3Rb
a3R2b
a3R3b
a3R6b
The correct Answer is:A
To solve the problem of finding the total heat produced in a resistance R when the charge Q flowing through it varies with time t as Q=at−bt2, we can follow these steps:
Step 1: Differentiate Charge to Find Current
The current I is defined as the rate of change of charge with respect to time. Therefore, we can express the current as:
I=dQdt=ddt(at−bt2)
Calculating the derivative:
I=a−2bt
Step 2: Identify the Time When Current is Zero
To find the time when the current is zero, we set I=0:
a−2bt=0
Solving for t:
t=a2b
Step 3: Write the Expression for Heat Produced
The heat produced H in a resistor is given by the formula:
H=∫T0I2Rdt
where T is the time interval from 0 to a2b.
Step 4: Substitute Current into the Heat Formula
Substituting I=a−2bt into the heat formula:
H=∫a2b0(a−2bt)2Rdt
Step 5: Expand the Expression
Expanding (a−2bt)2:
(a−2bt)2=a2−4abt+4b2t2
Thus, the heat produced becomes:
H=R∫a2b0(a2−4abt+4b2t2)dt
Step 6: Integrate Each Term
Now, we integrate each term:
H=R(a2t−2abt2+4b2t33)a2b0
Evaluating at the limits:
H=R⎛⎜
⎜⎝a2(a2b)−2ab(a2b)2+4b2(a2b)33⎞⎟
⎟⎠
Step 7: Simplify the Expression
Calculating each term:
1. a2(a2b)=a32b
2. −2ab(a2b)2=−2ab⋅a24b2=−a32b
3. 4b2(a2b)33=4b2⋅a38b33=a36b
Combining these:
H=R(a32b−a32b+a36b)=R⋅a36b
Final Answer
Thus, the total heat produced in the resistance R is:
H=a3R6
The current I is defined as the rate of change of charge with respect to time. Therefore, we can express the current as:
I=dQdt=ddt(at−bt2)
Calculating the derivative:
I=a−2bt
To find the time when the current is zero, we set I=0:
a−2bt=0
Solving for t:
t=a2b
The heat produced H in a resistor is given by the formula:
H=∫T0I2Rdt
where T is the time interval from 0 to a2b.
Substituting I=a−2bt into the heat formula:
H=∫a2b0(a−2bt)2Rdt
Expanding (a−2bt)2:
(a−2bt)2=a2−4abt+4b2t2
Thus, the heat produced becomes:
H=R∫a2b0(a2−4abt+4b2t2)dt
Now, we integrate each term:
H=R(a2t−2abt2+4b2t33)a2b0
Evaluating at the limits:
H=R⎛⎜
⎜⎝a2(a2b)−2ab(a2b)2+4b2(a2b)33⎞⎟
⎟⎠
Calculating each term:
1. a2(a2b)=a32b
2. −2ab(a2b)2=−2ab⋅a24b2=−a32b
3. 4b2(a2b)33=4b2⋅a38b33=a36b
H=R(a32b−a32b+a36b)=R⋅a36b
Thus, the total heat produced in the resistance R is:
H=a3R6
Topper's Solved these Questions
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - PRACTICE PAPPEREXERCISE - Practice Papper 250videos
PRACTICE PAPPER
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - PRACTICE PAPPEREXERCISE - Practice Papper 350videos
PRACTICE PAPPER
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - NUCLEIEXERCISE - Assertion And Reason15videos
NUCLEI
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - NCERT Exemplar11videos
RAY OPTICS AND OPTICAL INSTRUMENTS
Similar Questions
The charge Q( in coulomb) flowing through a resistance R=10Ω varies with time t (in second) as Q=2t−t2 . The total heat produced in resistance R is
View SolutionThe charger Q(in coulomb) flowing through a resistance (R=10Ω) varies with time t(in second) as (Q= 2t-t2). The total heat produced in resistance R is
View SolutionThe charge flowing in a conductor varies with times as Q=at−bt2. Then, the current
View SolutionThe charge flowing in a conductor varies with times as Q=at−bt2. Then, the current
View SolutionA charge passing through a resistor varies with time as shown in the figure. The amount of heat generated in time t is best represented (as a function of time ) by
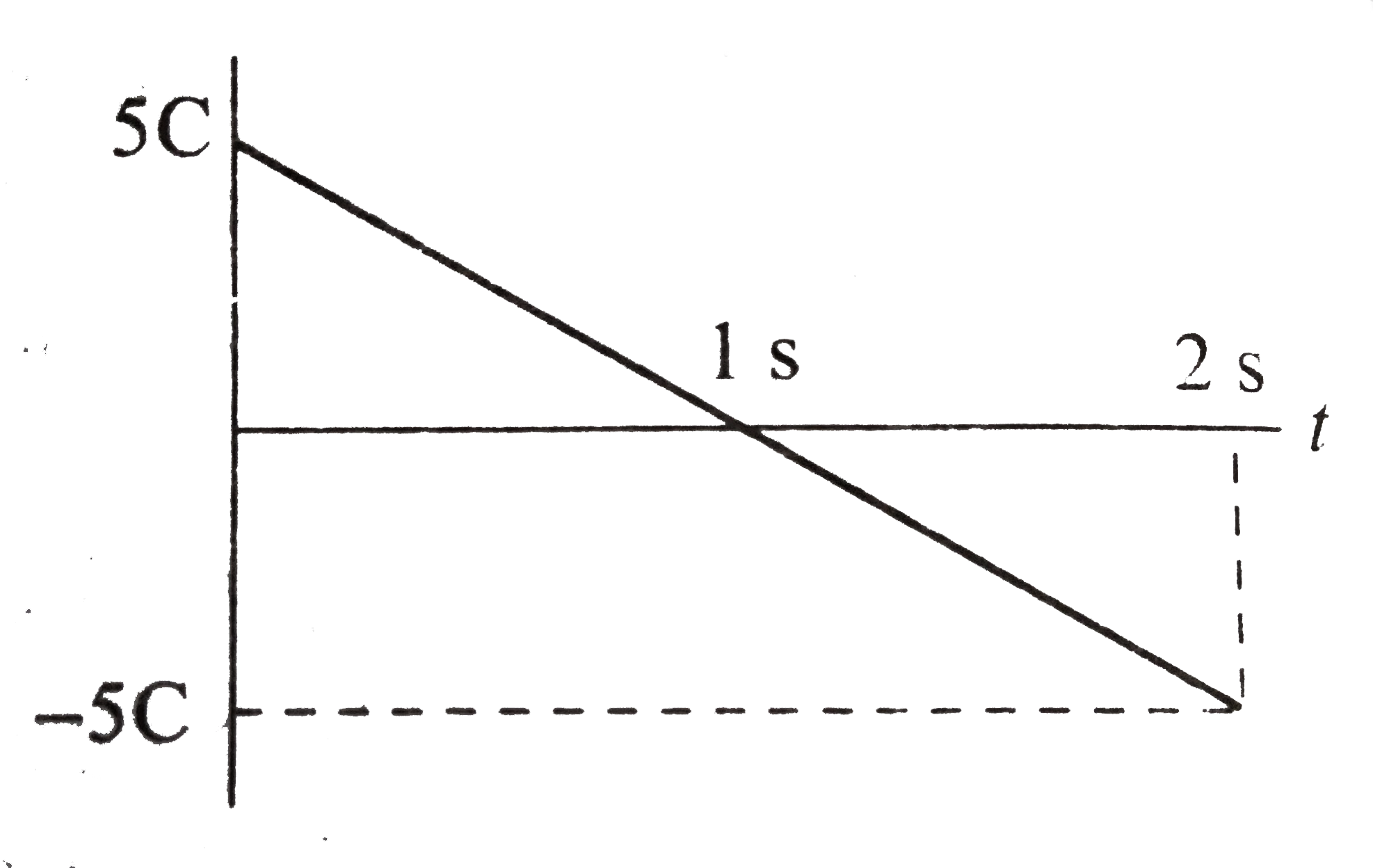 View Solution
View SolutionThe charge q flowing in a conductor of uniform cross section, varies with time as q=αt−bt2. Then, the current
View SolutionThe charge flowing in a conductor varies with time as Q=at−12bt2+16ct3 where a, b and c are positive constants. If at time t, the current in the conductor is i, which of the following graphs is correct ?
View SolutionThe linear momentum of a particle varies with time t as p=a+bt+ct2. Then, whichh of the following is correct?
View SolutionA magnetic flux through a stationary loop with a resistance R varies during the time interval τ as ϕ=at(τ−t). Find the amount of heat the generated in the loop during that time
View SolutionIn the current shows Fig x is joined to Y for a long time and then X is joined to Z. The total heat produced in R2 is
 View Solution
View Solution

