The correct M-H curve for a paramagnetic material at a constant temperature (T) is represented by
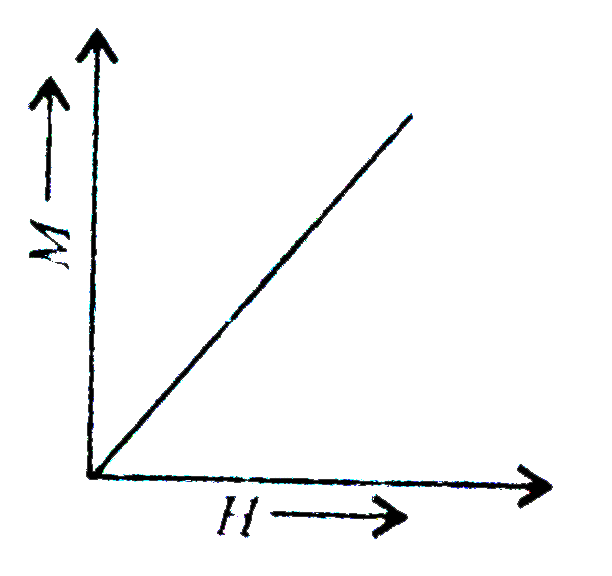
The correct Answer is:A
To determine the correct M-H curve for a paramagnetic material at a constant temperature, we can follow these steps:
1. Understanding the Variables:
- M represents the magnetization of the material.
- H represents the magnetic intensity.
2. Applying Curie's Law:
- According to Curie's Law, the magnetization (M) of a paramagnetic material is directly proportional to the magnetic field (B) and inversely proportional to the temperature (T).
- Mathematically, this can be expressed as:
M∝BT
3. Introducing the Curie Constant:
- We can introduce a constant (C), known as the Curie constant, to express the relationship:
M=C⋅BT
4. Relating B to H:
- The magnetic field B is related to the magnetic intensity H by the equation:
B=μ0⋅H
- Here, μ0 is the permeability of free space, which is a constant.
5. Substituting B in the Magnetization Equation:
- We can substitute the expression for B into the magnetization equation:
M=C⋅(μ0⋅H)T
6. Simplifying the Equation:
- This can be rearranged to:
M=(C⋅μ0T)⋅H
- Let k=C⋅μ0T, where k is a constant since C, μ0, and T are constants at constant temperature.
7. Final Relationship:
- Thus, we have:
M=k⋅H
- This indicates that M is directly proportional to H, suggesting a linear relationship.
8. Graphical Representation:
- The M-H curve will be a straight line passing through the origin, indicating that as the magnetic intensity (H) increases, the magnetization (M) also increases linearly.
9. Conclusion:
- Therefore, the correct M-H curve for a paramagnetic material at a constant temperature is represented by a straight line graph.
- M represents the magnetization of the material.
- H represents the magnetic intensity.
- According to Curie's Law, the magnetization (M) of a paramagnetic material is directly proportional to the magnetic field (B) and inversely proportional to the temperature (T).
- Mathematically, this can be expressed as:
M∝BT
- We can introduce a constant (C), known as the Curie constant, to express the relationship:
M=C⋅BT
- The magnetic field B is related to the magnetic intensity H by the equation:
B=μ0⋅H
- Here, μ0 is the permeability of free space, which is a constant.
- We can substitute the expression for B into the magnetization equation:
M=C⋅(μ0⋅H)T
- This can be rearranged to:
M=(C⋅μ0T)⋅H
- Let k=C⋅μ0T, where k is a constant since C, μ0, and T are constants at constant temperature.
- Thus, we have:
M=k⋅H
- This indicates that M is directly proportional to H, suggesting a linear relationship.
- The M-H curve will be a straight line passing through the origin, indicating that as the magnetic intensity (H) increases, the magnetization (M) also increases linearly.
- Therefore, the correct M-H curve for a paramagnetic material at a constant temperature is represented by a straight line graph.
Topper's Solved these Questions
- MAGNETISM AND MATTER BOOK - NCERT FINGERTPS ENGLISHCHAPTER - MAGNETISM AND MATTER EXERCISE - PERMANENT MAGNETS AND ELECTROMAGNETS
4 Videos
- MAGNETISM AND MATTER BOOK - NCERT FINGERTPS ENGLISHCHAPTER - MAGNETISM AND MATTER EXERCISE - HIGHER ORDER THINKING SKILLS (HOTS)
8 Videos
- MAGNETISM AND MATTER BOOK - NCERT FINGERTPS ENGLISHCHAPTER - MAGNETISM AND MATTER EXERCISE - MAGNETISATION AND MAGNETIC INTENSITY
11 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - Assertion And Reason
15 Videos
- MOVING CHARGES AND MAGNETISM BOOK - NCERT FINGERTPS ENGLISHCHAPTER - MOVING CHARGES AND MAGNETISM EXERCISE - Assertion And Reason
15 Videos
Similar Questions
When a sample of ideal gas is changed from an initial state to a final state, various curves can be plotted for the process like P-V curve, V-T curve,P-T curve etc.
For example, P-V curve for a fixed amount of an ideal gas at constant temperature is a rectangular hyperbola, V-T curve for a fixed amount of an ideal gas at constant volume is again a straight line. However, the shapes may vary if the constant parameters are also changed.
Now, answer the following questions :
From the given plot between Z and P for a real gas the correct is :

View SolutionWhen a sample of ideal gas is changed from an initial state to a final state, various curves can be plotted for the process like P-V curve, V-T curve,P-T curve etc.
For example, P-V curve for a fixed amount of an ideal gas at constant temperature is a rectangular hyperbola, V-T curve for a fixed amount of an ideal gas at constant volume is again a straight line. However, the shapes may vary if the constant parameters are also changed.
Now, answer the following questions :
Two Vander waals gases have same value 'b' but different 'a' value then which of the following statement is correct under simialr condition .
View SolutionWhen a sample of ideal gas is changed from an initial state to a final state, various curves can be plotted for the process like P-V curve, V-T curve,P-T curve etc.
For example, P-V curve for a fixed amount of an ideal gas at constant temperature is a rectangular hyperbola, V-T curve for a fixed amount of an ideal gas at constant volume is again a straight line. However, the shapes may vary if the constant parameters are also changed.
Now, answer the following questions :
Two moles of an ideal gas is changed from its initial state (16 atm, 6L) to final state (4 atm, 15L) in such a way that this change can be represented by a straight line in P-V curve.The maximum temperature attained by the gas during the above change is :(Take : R=112LatmK−1mol−1)
View SolutionWhen a sample of ideal gas is changed from an initial state to a final state, various curves can be plotted for the process like P-V curve, V-T curve,P-T curve etc.
For example, P-V curve for a fixed amount of an ideal gas at constant temperature is a rectangular hyperbola, V-T curve for a fixed amount of an ideal gas at constant volume is again a straight line. However, the shapes may vary if the constant parameters are also changed.
Now, answer the following questions :
Which of the following graphs is not possible for a fixed amount of ideal gas upon moving from initial state A to final state B :
View Solutionx1andx2 are susceptibility of a Paramagnetic material at temperatures T1,KandT2K respectively, then
View SolutionAccording to Curie's law, the magnetic susceptibilty of a paramagnetic substance at an absolute temperature T is proportional to
View SolutionAccording to Curie's law, the magnetic susceptibilty of a paramagnetic substance at an absolute temperature T is proportional to
View SolutionHow does the magnetic susceptibility of a paramagnetic material change with temperature?
View SolutionThe graph between χ and T for paramagnetic material will be represented by
View SolutionThe correct graph which shows the variation of paramagnetic susceptibility S with temperature T is
View Solution
