A block of mass 2 kg is hanging over a smooth and light pulley through a light string. The other end of the string is pulled by a constant force F = 40 N. The kinetic energy of the particle increases 40 J in a given interval of time. Then, (g=10m/s2)
tension in the string is 40 N
displacement of the block in the given interval of time is 2 m
work done by gravity is −20J
work done by tension is 80 J
The correct Answer is:A, B, D
To solve the problem, we need to analyze the forces acting on the block and the work done on it. Here's a step-by-step solution:
Step 1: Identify the forces acting on the block
The block of mass m=2kg is hanging and is subjected to two forces:
1. The gravitational force acting downwards: Fg=m⋅g=2kg⋅10m/s2=20N
2. The tension T in the string, which is equal to the force F=40N pulling the other end of the string.
Step 2: Calculate the net force acting on the block
The net force Fnet acting on the block can be calculated as:
Fnet=T−Fg=40N−20N=20N
Step 3: Calculate the acceleration of the block
Using Newton's second law, F=m⋅a, we can find the acceleration a of the block:
a=Fnetm=20N2kg=10m/s2
Step 4: Relate the change in kinetic energy to work done
The change in kinetic energy ΔKE is given as 40J. According to the work-energy theorem, the work done W on the block is equal to the change in kinetic energy:
W=ΔKE=40J
Step 5: Calculate the distance moved by the block
The work done can also be expressed as:
W=Fnet⋅d
where d is the distance moved by the block. Rearranging gives:
d=WFnet=40J20N=2m
Final Answer
The distance moved by the block is 2m.
---
The block of mass m=2kg is hanging and is subjected to two forces:
1. The gravitational force acting downwards: Fg=m⋅g=2kg⋅10m/s2=20N
2. The tension T in the string, which is equal to the force F=40N pulling the other end of the string.
The net force Fnet acting on the block can be calculated as:
Fnet=T−Fg=40N−20N=20N
Using Newton's second law, F=m⋅a, we can find the acceleration a of the block:
a=Fnetm=20N2kg=10m/s2
The change in kinetic energy ΔKE is given as 40J. According to the work-energy theorem, the work done W on the block is equal to the change in kinetic energy:
W=ΔKE=40J
The work done can also be expressed as:
W=Fnet⋅d
where d is the distance moved by the block. Rearranging gives:
d=WFnet=40J20N=2m
The distance moved by the block is 2m.
Topper's Solved these Questions
- WORK, POWER AND ENERGYBOOK - DC PANDEY ENGLISHCHAPTER - WORK, POWER AND ENERGYEXERCISE - C Comprehension Type Questions
18 Videos
- WORK, POWER AND ENERGYBOOK - DC PANDEY ENGLISHCHAPTER - WORK, POWER AND ENERGYEXERCISE - D Matrix Matching Type Questions
10 Videos
- WORK, POWER AND ENERGYBOOK - DC PANDEY ENGLISHCHAPTER - WORK, POWER AND ENERGYEXERCISE - A Only One Option is Correct
42 Videos
- WORK, ENERGY AND POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - MEDICAL ENTRACES GALLERY
33 Videos
Similar Questions
A block of mass M is hanging over a smooth and light pulley through a light string. The other end of the string is pulled by a constant force F. The kinetic energy of the block increases by 20J in 1s.
View SolutionA block of mass 10 kg is hanging over a smooth and light pulley through a light string and the other end of the string is pulled down by a constant force F. The kinetic energy of the block increases by 20 J in 1 s
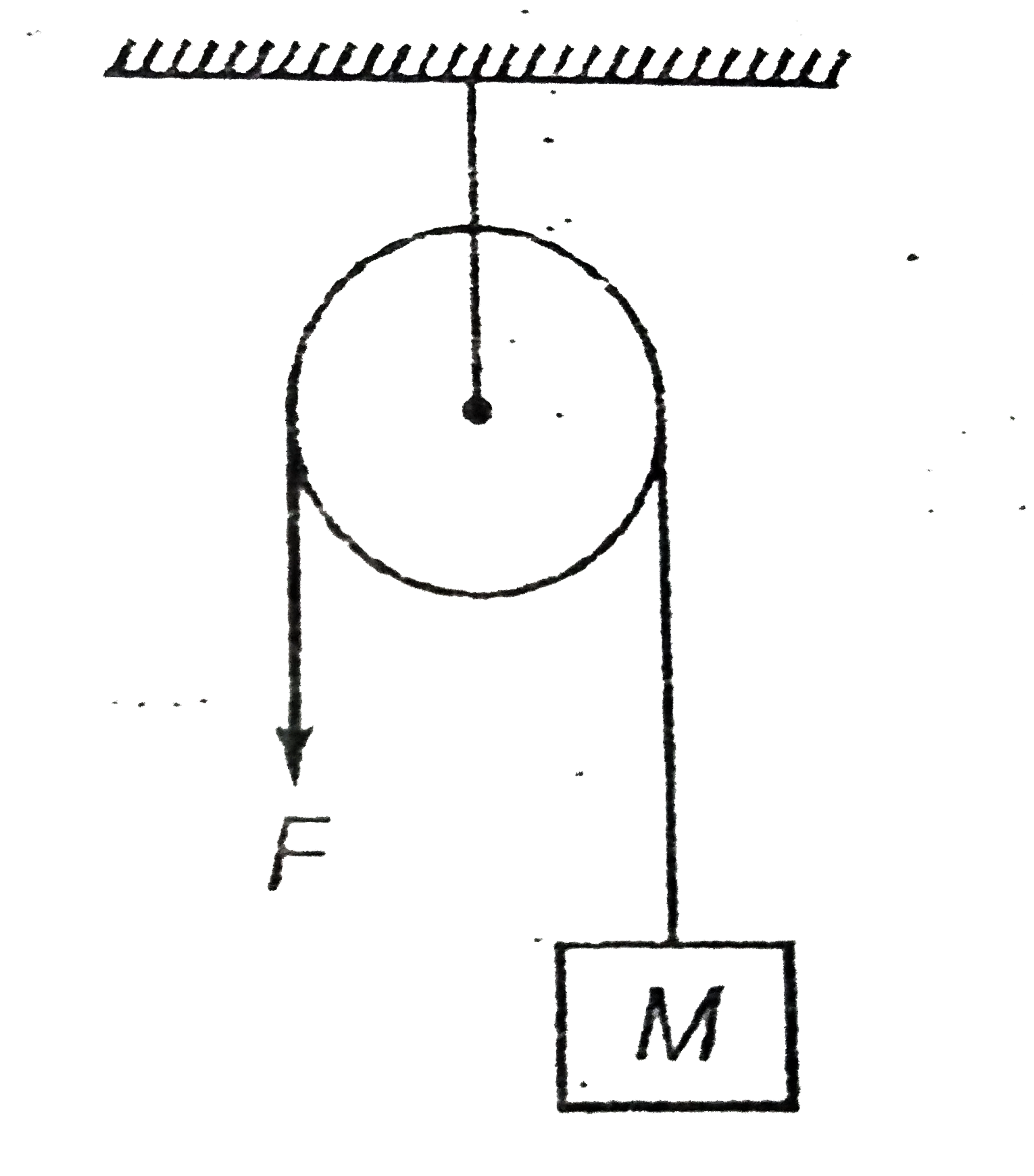
View SolutionA block of mass M hanging over a smooth and light pulley through a light string. The other end of the string is pulled by a constant force F. The kinetic of the block increases by 40J in 1s. State whether the following statements are true or false.
(a) The tension in the string is Mg .
(b) The work done the tension on the block is 40J.
(c) the tension in the string is F.
(d) The work done by the force of gravity is 40J in the above 1s .
View SolutionA block of mass 2kg is hanging over a smooth and light pulley through a light string. The other end of the string is pulled by a constant force F=40N . At t=0 the system is at rest as shown. Then in the time interval from t=0 to t=2√10 seconds, pick up the correct statement (s):(g=10m/s2)
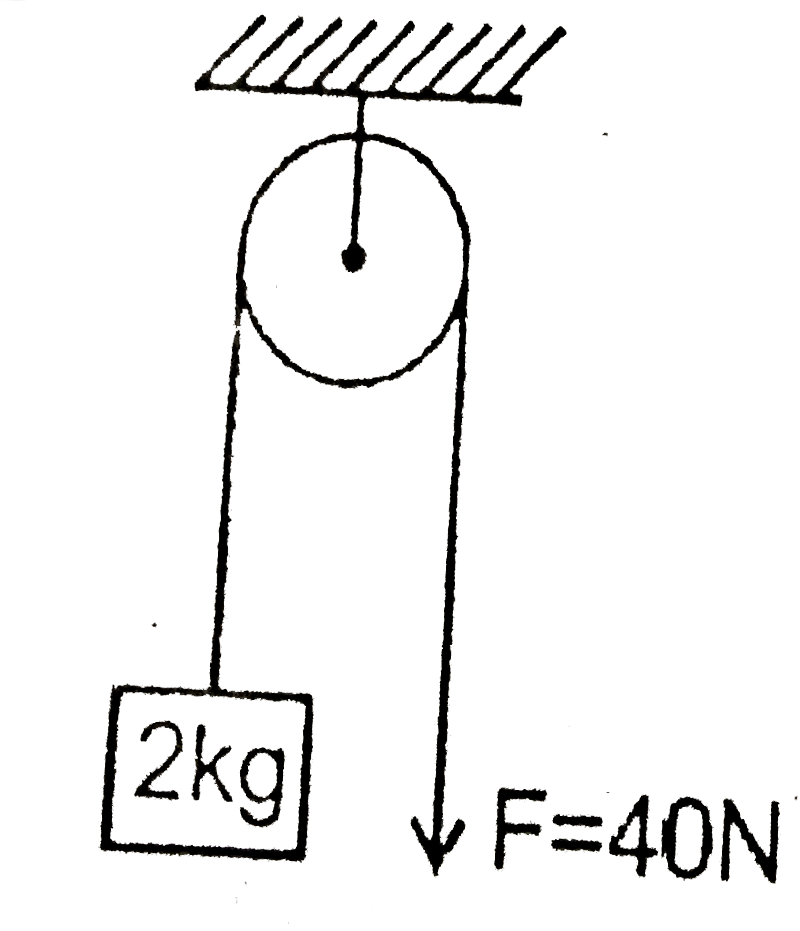
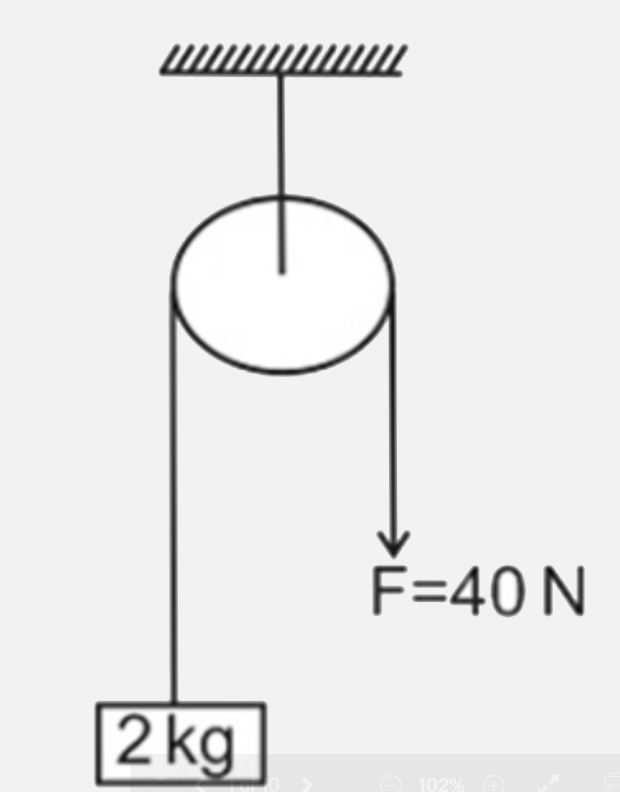
View SolutionIn the system shown in the figure, the block is pulled by applying a force of 40 N on the other end of the string. If the kinetic energy of the block increases by 40 J in a given interval of time then work done by tension on the block is (g=10ms−2)
View Solution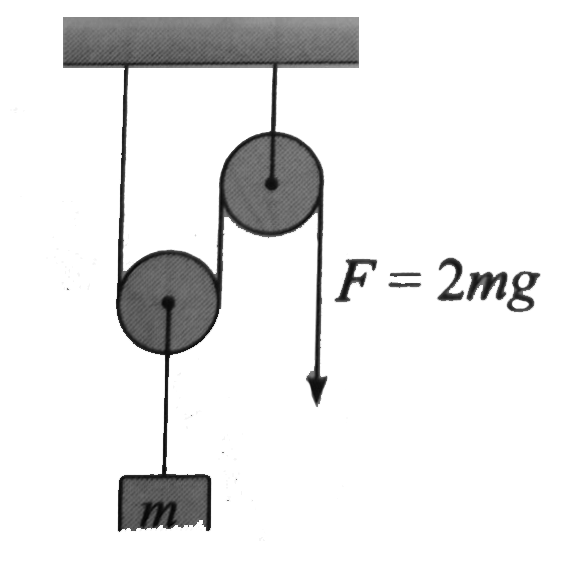
In the shown mass pulley system, pulleys and string are massless. The one end of the string is pulleed by the force F=2mg. The acceleration of the block will be
View Solution
In the shown mass pulley system, pulleys and string are massless. The one end of the string is pulleed by the force F=2mg. The acceleration of the block will be
View SolutionTwo blocks of equal mass m are tied to each other through light string. One of the blocks is pulled along the line joining them with a constant force F. Find the tension in the string joining the blocks.
View SolutionA 1m long rope, having a mass of 40g, is fixed at one end and is tied to a light string at the other end. The tension in the string in 400N. Find the wavelength in second overtone (in cm).
View SolutionA bob is hanging over a pulley inside a car through a string. The second end of the string is in the hands of a person standing in the car. The car is moving with constant acceleration a directed horizontally as shown in fig. The other end of the string is pulled with constant acceleration a vertically. The tension in the string is equal to

View Solution
