One end of a light spring of spring constant k is fixed to a wall and the other end is tied to a block placed on a smooth horizontal surface. In a displacement, the work done by the spring is +(12)kx2. The possible cases are.
the spring was initially stretched by a distance x and finally was in its natural length
the spring was initially in its natural length and finally it was compressed by a distance x
the spring was initially compressed by a distance x and finally was in its natural length
the spring was initally in its natural length and finally stretched by a distance x
The correct Answer is:A, C
To solve the problem, we need to analyze the work done by the spring in different scenarios based on its displacement. The work done by the spring is given by the formula:
W=12kx2
where k is the spring constant and x is the displacement from the spring's natural length.
1. Understanding the Spring System:
- One end of the spring is fixed to a wall, and the other end is attached to a block on a smooth horizontal surface.
- The spring can either be compressed or extended from its natural length.
2. Work Done by the Spring:
- The work done by the spring is positive when the spring is either compressed or extended, as long as the displacement and the force exerted by the spring are in the same direction.
- The formula for work done by the spring indicates that the work is dependent on the square of the displacement x.
3. Analyzing Different Cases:
- Case A: The spring is initially stretched (extended) and then released. As it returns to its natural length, the displacement and the spring force are in the same direction, resulting in positive work done.
- Case B: The spring is initially at its natural length. In this case, there is no displacement, so the work done is zero. This case does not yield positive work.
- Case C: The spring is initially compressed and then released. Similar to Case A, as it returns to its natural length, the displacement and the spring force are in the same direction, resulting in positive work done.
- Case D: Again, the spring is initially at its natural length, which results in zero work done.
4. Conclusion:
- The possible cases where the work done by the spring is positive are:
- Case A: Spring initially stretched.
- Case C: Spring initially compressed.
- Therefore, the correct answers are A and C.
Final Answer:
The correct cases where the work done by the spring is positive are:
- A: Spring was initially stretched.
- C: Spring was initially compressed.
- One end of the spring is fixed to a wall, and the other end is attached to a block on a smooth horizontal surface.
- The spring can either be compressed or extended from its natural length.
- The work done by the spring is positive when the spring is either compressed or extended, as long as the displacement and the force exerted by the spring are in the same direction.
- The formula for work done by the spring indicates that the work is dependent on the square of the displacement x.
- Case A: The spring is initially stretched (extended) and then released. As it returns to its natural length, the displacement and the spring force are in the same direction, resulting in positive work done.
- Case B: The spring is initially at its natural length. In this case, there is no displacement, so the work done is zero. This case does not yield positive work.
- Case C: The spring is initially compressed and then released. Similar to Case A, as it returns to its natural length, the displacement and the spring force are in the same direction, resulting in positive work done.
- Case D: Again, the spring is initially at its natural length, which results in zero work done.
- The possible cases where the work done by the spring is positive are:
- Case A: Spring initially stretched.
- Case C: Spring initially compressed.
- Therefore, the correct answers are A and C.
The correct cases where the work done by the spring is positive are:
- A: Spring was initially stretched.
- C: Spring was initially compressed.
Topper's Solved these Questions
- WORK, POWER AND ENERGYBOOK - DC PANDEY ENGLISHCHAPTER - WORK, POWER AND ENERGYEXERCISE - C Comprehension Type Questions
18 Videos
- WORK, POWER AND ENERGYBOOK - DC PANDEY ENGLISHCHAPTER - WORK, POWER AND ENERGYEXERCISE - D Matrix Matching Type Questions
10 Videos
- WORK, POWER AND ENERGYBOOK - DC PANDEY ENGLISHCHAPTER - WORK, POWER AND ENERGYEXERCISE - A Only One Option is Correct
42 Videos
- WORK, ENERGY AND POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - MEDICAL ENTRACES GALLERY
33 Videos
Similar Questions
One end of a light spring of spring constant k is fixed to a wall and the other end is tied to a block placed on a smooth horizontal surface. In a displacement, the work by the spring is 12kx2. The possible cases are
View SolutionOne end of a spring of force constant k is fixed to a vertical wall and the other to a blcok of mass m resting on a smooth horizontal surface. There is another wall at distance x0 from the block. The spring is then compressed by 2x0 and released. The time taken to strike the wall is
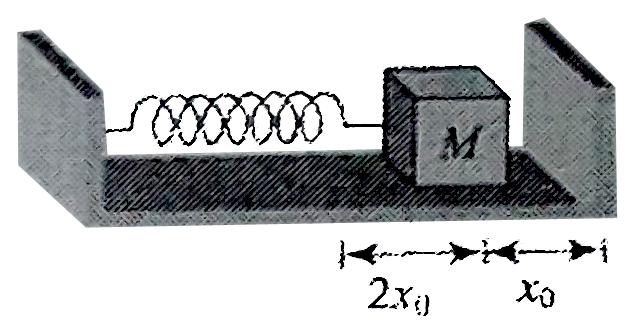
View SolutionOne end of a spring of force constant k is fixed to a vertical wall and the other to a blcok of mass m resting on a smooth horizontal surface. There is another wall at distance x0 from the block. The spring is then compressed by 2x0 and released. The time taken to strike the wall is

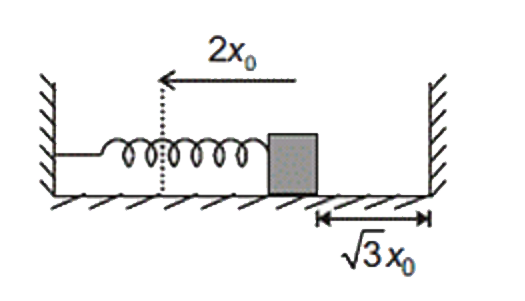
View SolutionOne end of a spring of force constant K is fixed to a vertical wall and other to a body of mass m resting on smooth horizontal surface. There is another wall at a distance of √3x0 from the body. If all the collisions are elastic and spring is compressed by 2x0and released, then the time period of oscillation is
View SolutionA block of mass m is attached to one end of a mass less spring of spring constant k. the other end of spring is fixed to a wall the block can move on a horizontal rough surface. The coefficient of friction between the block and the surface is μ then the compession of the spring for which maximum extension of the spring becomes half of maximum compression is .
View SolutionOne end of a light spring of natural length d and spring constant k is fixed on a rigid wall and the other is attached to a smooth ring of mass m which can slide without friction on a vertical rod fixed at a distance d from the wall. Initially the spring makes an angle of 37∘ with the horizontal as shown in fig. When the system is released from rest, find the speed of the ring when the spring becomes horizontal.
[ sin 37∘ = 3/5]
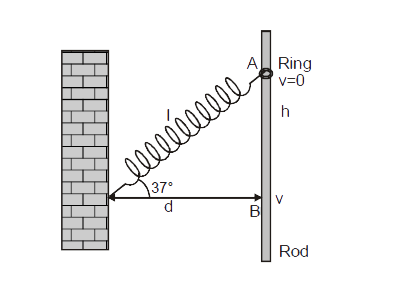
View SolutionOn end of a light spring of natural length d and spring constant k is fixed on a rigid wall and the other is attached to a smooth ring of mass m which can slide without friction on a vertical rod fixed at a distance d from the wall. Initially the spring makes an angle of 37∘ with the horizontal. as shown in figure. When the system is released from rest, find the speed of the ring when the spring becomes horizontal. (sin37∘=35).

View SolutionOne end of a light spring of natural length 4m and spring constant 170 N/m is fixed on a rigid wall and the other is fixed to a smooth ring of mass 12 kg which can slide without friction in a verical rod fixed at a distance 4 m from the wall. Initially the spring makes an angle of 37∘ with the horizontal as shown in figure. When the system is released from rest, find the speed of the ring when the spring becomes horizontal.
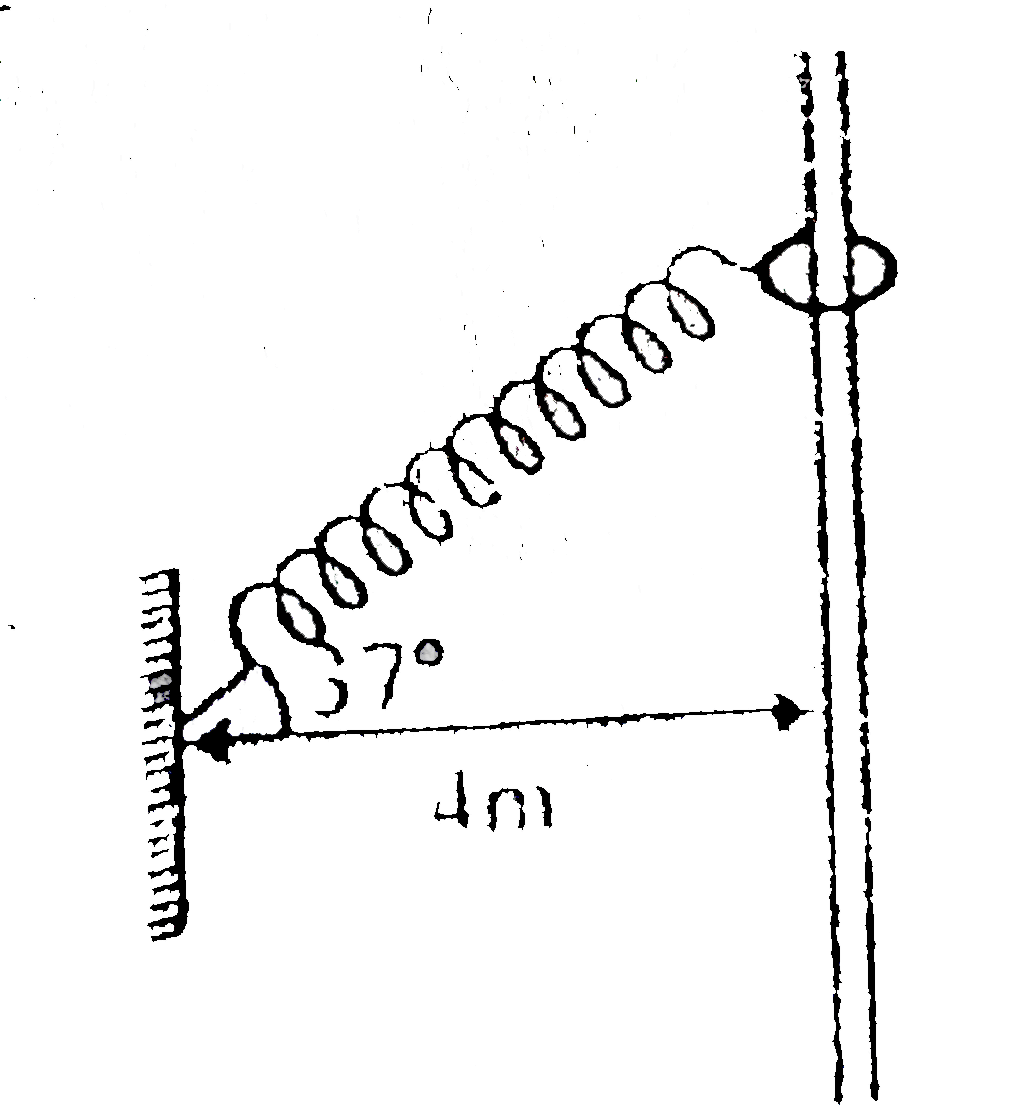
View SolutionTwo blocks, of masses M and 2M, are connected to a light spring of spring constant K that has one end fixed, as shown in figure. The horizontal surface and the pulley are frictionless. The blocks are released from when the spring is non deformed. The string is light.
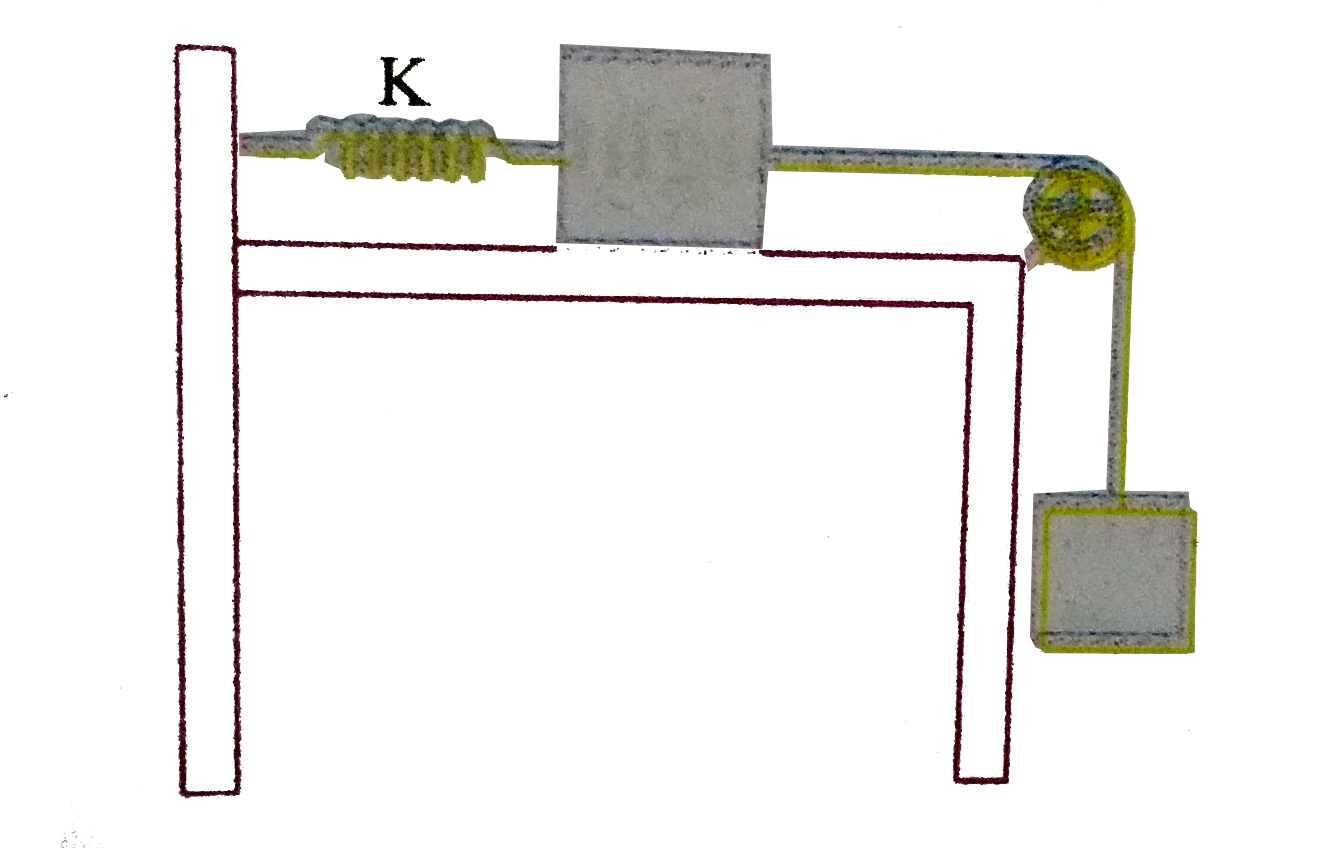 .
.
View SolutionTwo blocks, of masses M and 2M, are connected to a light spring of spring constant K that has one end fixed, as shown in figure. The horizontal surface and the pulley are frictionless. The blocks are released from when the spring is non deformed. The string is light.
 .
.
View Solution
