A resistance R carries a current i. The power lost to the surrounding is λ(θ−θ0). Here, λ is a constant, θ is temperature of the resistance and θ0 is the temperature of the atmosphere. If the corfficient of linear expansion is α. The strain in the resistance is
αλl2R
αλ/R
αl2R2λ
proporitonal to the length of the resistance wire
The correct Answer is:A
To find the strain in the resistance R that carries a current i, we will follow these steps:
1. Understanding Power Loss:
The power lost to the surroundings is given by the equation:
Ploss=λ(θ−θ0)
where λ is a constant, θ is the temperature of the resistance, and θ0 is the temperature of the atmosphere.
2. Power Generated by the Resistance:
The power generated due to the current flowing through the resistance is given by:
Pgenerated=I2R
At steady state, the power generated equals the power lost:
I2R=λ(θ−θ0)
3. Rearranging for Temperature Difference:
From the equation above, we can express the temperature difference θ−θ0:
θ−θ0=I2Rλ
4. Change in Length due to Temperature Change:
The change in length ΔL of the resistance due to the temperature change can be expressed using the coefficient of linear expansion α:
ΔL=LαΔθ
where Δθ=θ−θ0. Substituting the expression for Δθ:
ΔL=Lα(I2Rλ)
5. Calculating Strain:
The strain ϵ in the resistance is defined as the change in length per unit original length:
ϵ=ΔLL
Substituting the expression for ΔL:
ϵ=Lα(I2Rλ)L
Simplifying this gives:
ϵ=I2Rαλ
Final Answer:
The strain in the resistance is:
ϵ=I2Rαλ
The power lost to the surroundings is given by the equation:
Ploss=λ(θ−θ0)
where λ is a constant, θ is the temperature of the resistance, and θ0 is the temperature of the atmosphere.
The power generated due to the current flowing through the resistance is given by:
Pgenerated=I2R
At steady state, the power generated equals the power lost:
I2R=λ(θ−θ0)
From the equation above, we can express the temperature difference θ−θ0:
θ−θ0=I2Rλ
The change in length ΔL of the resistance due to the temperature change can be expressed using the coefficient of linear expansion α:
ΔL=LαΔθ
where Δθ=θ−θ0. Substituting the expression for Δθ:
ΔL=Lα(I2Rλ)
The strain ϵ in the resistance is defined as the change in length per unit original length:
ϵ=ΔLL
Substituting the expression for ΔL:
ϵ=Lα(I2Rλ)L
Simplifying this gives:
ϵ=I2Rαλ
The strain in the resistance is:
ϵ=I2Rαλ
Topper's Solved these Questions
Similar Questions
The radius of a ring is R and its coefficient of linear expansion is α. If the temperature of ring increases by θ then its circumfrence will increase by
View SolutionThe linear charge density on a ring of radius R is λ=λ0sin(θ) where λ0 is a constant and θ is angle of radius vector of any point on the ring with x-axis. The electric potential at centre of ring is
View SolutionA liquid in a beaker has temperature θ(t) at time t and θ0 is temperature of surroundings, then according to Newton's law of cooling the correct graph between loge(θ−θ0) and t is :
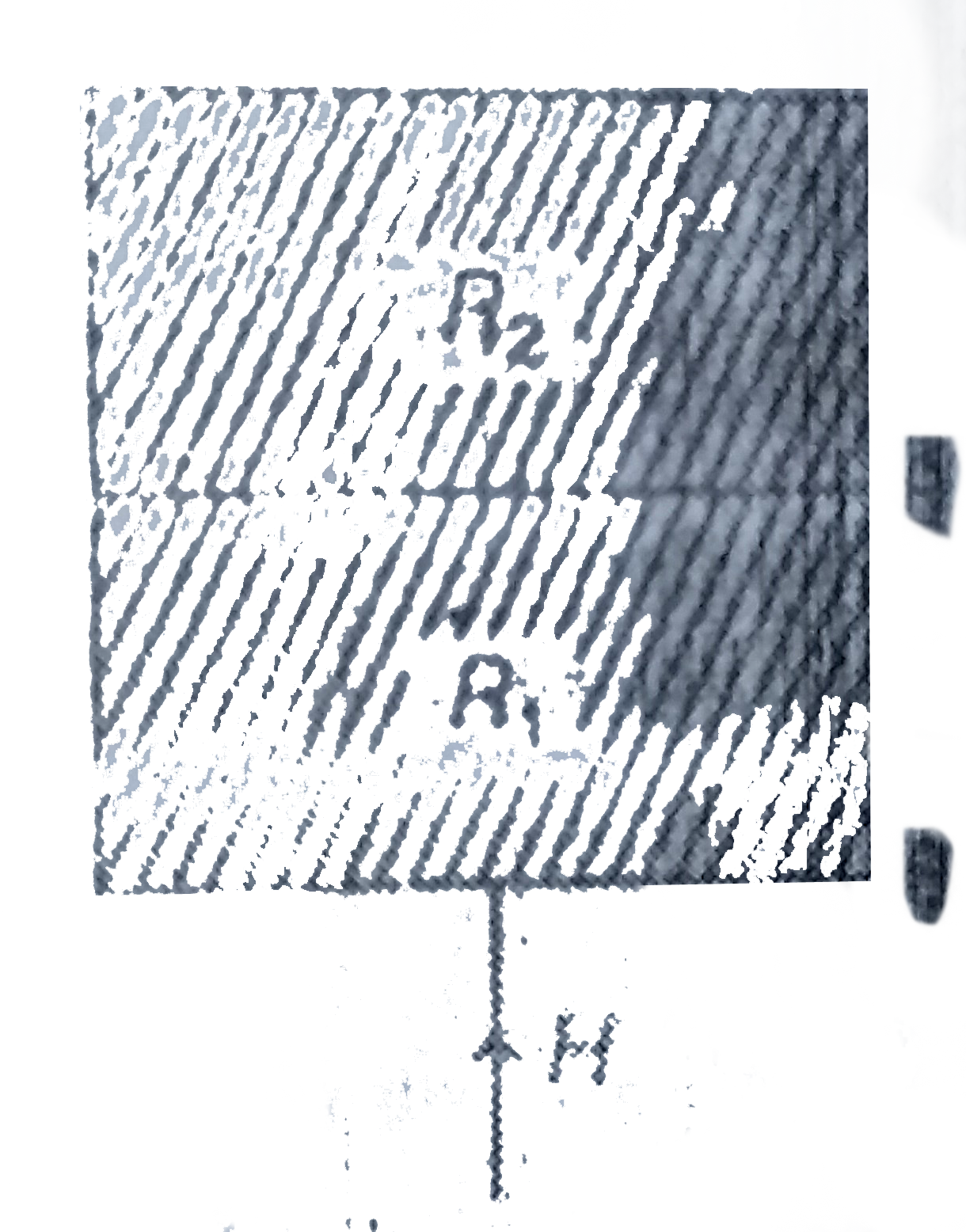
View SolutionConsider the two insulating sheets with thermal resistance R1 and R2 as shown in figure. The temperature θ is
View SolutionThemperature of a body θ is slightly more than the temperature of the surrounding θ0 its rate of cooling (R) versus temperature of body (θ) is plotted its shape would be .
View SolutionIn the relation, P=αβeαZkθP is pressure, Z is distance, k is Boltzmann constant and θ is the temperature. The dimensional formula of β will be-
View SolutionThe electrical resistance of pure platinum increases linearly with increasing temperature over a small range of temperature . This property is used in a Platinum resistance thermometer. The relation between Rθ (Resistance at θ K) and R0 (Resistance at θ0K) is given by Rθ=R0[1+a(θ−θ0)], where α= temperature coefficient of resistance. Now, if a Platinum resistance thermometer reads 0∘C when its resistance is 80Ω and 100∘C when its resistance is 90Ω find the temperature at which its resistance is 86Ω.
View SolutionThe temperature of water in calorimeter is θ and the temperature of surrounding is θ0(<θ). Observation are recorded for temperature difference (θ−θ0) and time t. The cooling curve is best represented in graph.
View SolutionIf a piece of metal is heated to temperature θ and the allowed to cool in a room which is at temperature θ0, the graph between the temperature T of the metal and time t will be closet to
View SolutionTwo equal resistance at 0∘C with temperature coefficient of resistance α1 and α2 joined in series act as a single resistance in a circuit the temperature coefficient of their single resistance will be
View Solution