The unit of electric field is not equivalent to
N/C
J/C
V/m
J/C-m
The correct Answer is:B
To solve the problem of determining which unit of electric field is not equivalent, we need to analyze the units of electric field and the options provided.
1. Understanding the Electric Field:
The electric field (E) is defined as the force (F) experienced per unit charge (q). Mathematically, this is expressed as:
E=Fq
2. Identifying the Units:
- The unit of force (F) is Newton (N).
- The unit of charge (q) is Coulomb (C).
Therefore, the unit of electric field can be expressed as:
E=NC
3. Converting Units:
We can further analyze the unit of electric field by expressing Newton in terms of Joules and meters:
- 1 Joule (J) = 1 Newton (N) × 1 meter (m)
- Thus, we can rewrite Newton as:
N=Jm
Substituting this into the electric field equation gives:
E=J/mC=JC⋅m
4. Electric Field in Terms of Potential:
The electric field can also be expressed in terms of electric potential (V) and distance (R):
E=VR
- The unit of electric potential (V) is Volt (V), and the unit of distance (R) is meter (m).
- Therefore, the unit of electric field in this context is:
E=Vm
5. Conclusion:
After analyzing the units, we can conclude that the electric field can be expressed in several equivalent forms, but we need to identify which of the given options is not equivalent to these derived units.
Final Answer:
The unit of electric field is not equivalent to the option that does not match the derived units of NC, JC⋅m, or Vm.
The electric field (E) is defined as the force (F) experienced per unit charge (q). Mathematically, this is expressed as:
E=Fq
- The unit of force (F) is Newton (N).
- The unit of charge (q) is Coulomb (C).
Therefore, the unit of electric field can be expressed as:
E=NC
We can further analyze the unit of electric field by expressing Newton in terms of Joules and meters:
- 1 Joule (J) = 1 Newton (N) × 1 meter (m)
- Thus, we can rewrite Newton as:
N=Jm
Substituting this into the electric field equation gives:
E=J/mC=JC⋅m
The electric field can also be expressed in terms of electric potential (V) and distance (R):
E=VR
- The unit of electric potential (V) is Volt (V), and the unit of distance (R) is meter (m).
- Therefore, the unit of electric field in this context is:
E=Vm
After analyzing the units, we can conclude that the electric field can be expressed in several equivalent forms, but we need to identify which of the given options is not equivalent to these derived units.
The unit of electric field is not equivalent to the option that does not match the derived units of NC, JC⋅m, or Vm.
Topper's Solved these Questions
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (B) Chapter exercises
17 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (C) Chapter exercises
50 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - Check point 2.5
20 Videos
- ELECTROMAGNETIC WAVESBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Sec C
22 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Medical entrances gallery
37 Videos
Similar Questions
Knowledge Check
The SI unit of electric flux is
ANC−1m2BNCm−2CNC−2m2DNC−1m−2
Units of electric flux are
View Solution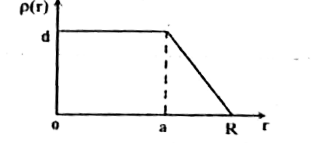
The electric field at r= R is
View SolutionThe unit of electrochemical equivalent is
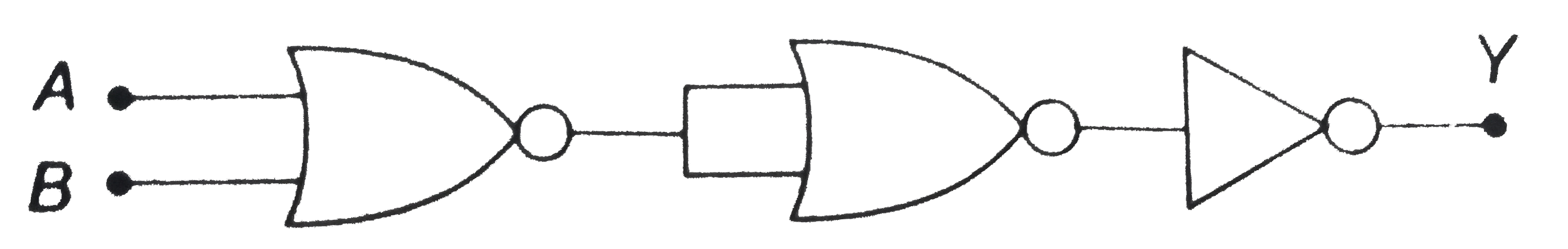
View SolutionThe given electrical network is equivalent to:
View Solutiona proton placed in an electric field would experience an electrical force equal to its weight. the magnitude of electric field intensity E would be
View SolutionConsider three charges q,−q, (in Sl units) at the vetrices of an equilateral triangle with side length b. The magnitude of electric field at the centroid of the triangle is :
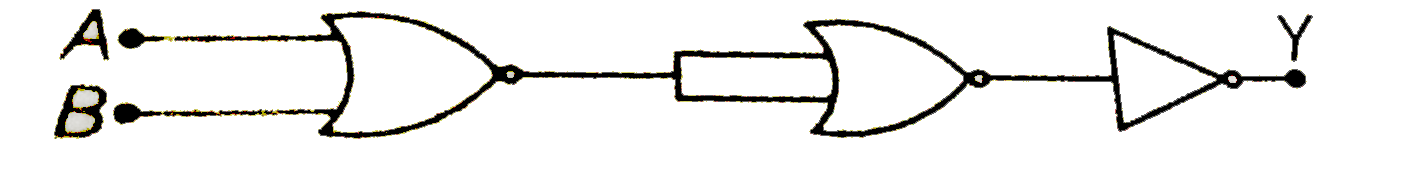
View SolutionThe given electrical network is equivalent to
View Solution
