Electric field (E) and current density (J) have relation
E∝J
E∝J2
E∝1J2
E2∝1J
The correct Answer is:A
To find the relationship between electric field (E) and current density (J), we can follow these steps:
Step 1: Understand the Definitions
- Electric Field (E): It is defined as the potential difference (V) across a conductor divided by the length (L) of the conductor.
E=VL
- Current Density (J): It is defined as the current (I) flowing through a conductor per unit cross-sectional area (A).
J=IA
Step 2: Apply Ohm's Law
According to Ohm's law, the current (I) flowing through a conductor can be expressed as:
I=VR
Where R is the resistance of the conductor. The resistance can be expressed in terms of resistivity (ρ), length (L), and area (A):
R=ρLA
Step 3: Substitute Resistance in Ohm's Law
Substituting the expression for R into Ohm's law gives:
I=VρLA=V⋅AρL
Step 4: Relate Current Density to Electric Field
Now, we can express the current density (J) in terms of the electric field (E):
1. From the definition of current density:
J=IA
Substitute I from the previous step:
J=V⋅AρL⋅A=VρL
2. Now, substitute the expression for E:
J=E⋅LρL
3. The length (L) cancels out:
J=Eρ
Step 5: Establish the Relationship
From the equation J=Eρ, we can see that:
- Current density (J) is directly proportional to the electric field (E) when resistivity (ρ) is constant.
Final Result
Thus, the relationship between electric field (E) and current density (J) is given by:
J∝E
- Electric Field (E): It is defined as the potential difference (V) across a conductor divided by the length (L) of the conductor.
E=VL
J=IA
According to Ohm's law, the current (I) flowing through a conductor can be expressed as:
Substituting the expression for R into Ohm's law gives:
Now, we can express the current density (J) in terms of the electric field (E):
J=IA
Substitute I from the previous step:
J=V⋅AρL⋅A=VρL
J=E⋅LρL
J=Eρ
From the equation J=Eρ, we can see that:
Thus, the relationship between electric field (E) and current density (J) is given by:
Topper's Solved these Questions
- CURRENT ELECTRICITYBook:DC PANDEY ENGLISHChapter:CURRENT ELECTRICITYExercise:B. Assertion and reason
Explore 18 Videos
- CURRENT ELECTRICITYBook:DC PANDEY ENGLISHChapter:CURRENT ELECTRICITYExercise:Match the columns
Explore 4 Videos
- CURRENT ELECTRICITYBook:DC PANDEY ENGLISHChapter:CURRENT ELECTRICITYExercise:Check point
Explore 70 Videos
- COMMUNICATION SYSTEMBook:DC PANDEY ENGLISHChapter:COMMUNICATION SYSTEMExercise:Subjective
Explore 11 Videos
- ELECTROMAGNETIC INDUCTIONBook:DC PANDEY ENGLISHChapter:ELECTROMAGNETIC INDUCTIONExercise:Medical entrances gallery
Explore 25 Videos
Similar Questions
Energy density in the electric field of electromagnetic wave is U.If amplitude of electric field (E0) and magnetic field (B0) is related as E0=cB0, then energy density in magnetic field is(c = speed of light)
View SolutionIn μe and μh are electron and hole mobility. E be the applied electric field, the current density τ for intristic semiconductor is equal to
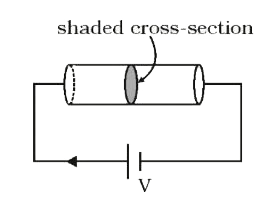
View SolutionColumn-I gives physical quantities based on a situation in which an ideal cell of e.m.f. V is connected across a cylindrical rod of uniform cross-section area and conductivity (σ ) as shown in figure. E, J, ϕ and i are electric field, current density, electric flux and current through shaded cross-section respectively as shown in figure. Physical quantities in Column-II are equal to those in column I. Match the expression in Column-I with the statements in Column-II.

View SolutionThe electric intesity E, current density j and conductivity σ are related as:
View SolutionIntensity of an electric field (E) depends on distance r due to a dipole, is related as
View SolutionA : When a steady current flows through a conductor of non - uniform cross-section , the current density , electric field and drift velcoity do not remain constant.
R : For a constant current the current density electric field and drift velocity are inversely proportional to cross - sectional area.
View SolutionA : In an electromagnetic wave the energy density in electric field is equal to energy density in magnetic field.
R : Electromagnetic waves are transverse in nature.
View SolutionThe work in rotating electric dipole of dipole moment p in an electric field E through an angle θ from the direction of electric field, is:
View SolutionAn infinite cylindrical wire of radius R and having current density varying with its radius r as, J=J0[1−(r/R)]. Then answer the following questions.
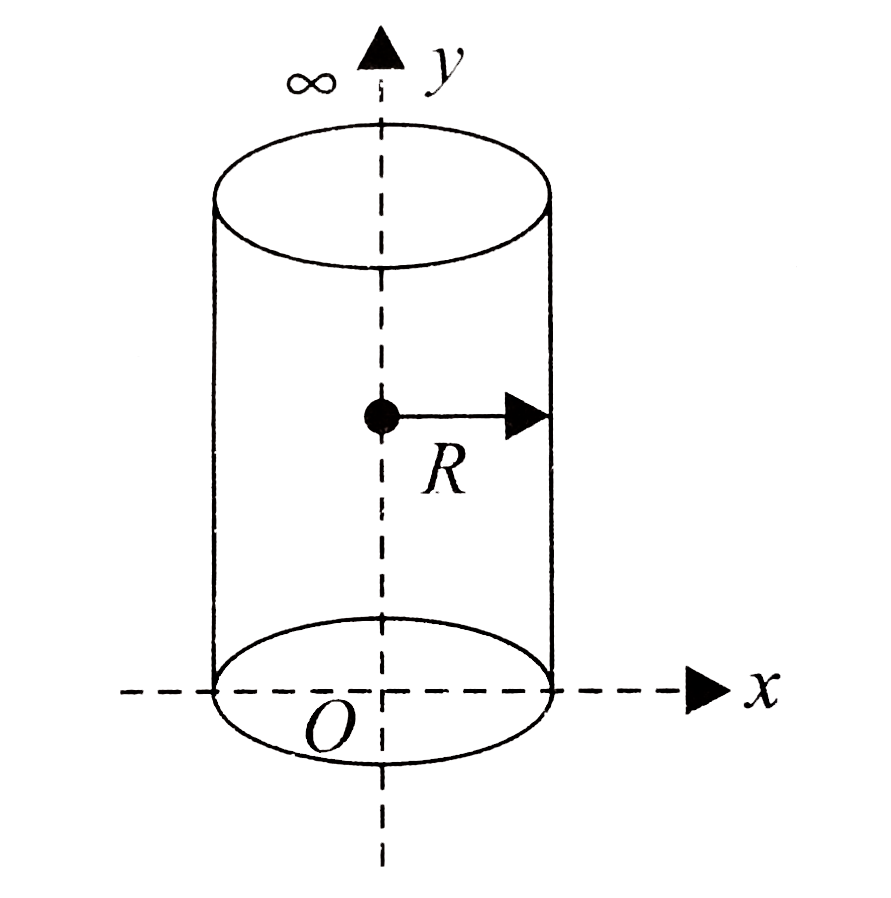
Graph between the magnetic field and radius is
View SolutionThe maximum electric field of a plane electro- mag-netic wave is 88 V/m. The average energy density is
View Solution