A voltmeter of resistance 998Ω is connected across a cell of emf 2 V and internal resistance 2Ω. Find the p.d. across the voltmeter, that across the terminals of the cell and percentage error in the reading of the voltmeter.
1.99V
3.5V
5 V
6 V
The correct Answer is:A
To solve the problem, we will follow these steps:
Step 1: Identify the given values
- EMF of the cell, e=2V
- Internal resistance of the cell, r=2Ω
- Resistance of the voltmeter, R=998Ω
Step 2: Calculate the potential difference (p.d.) across the voltmeter
The formula to calculate the potential difference across the voltmeter when connected to a cell is given by:
V=e⋅Rr+R
Substituting the known values into the formula:
V=2⋅9982+998
Calculating the denominator:
r+R=2+998=1000Ω
Now substituting back into the equation for V:
V=19961000=1.996V
Step 3: Calculate the potential difference across the terminals of the cell
The potential difference across the terminals of the cell is equal to the EMF minus the voltage drop across the internal resistance. The voltage drop across the internal resistance can be calculated using Ohm's law:
Voltage drop=I⋅r
Where I is the current flowing through the circuit. The current I can be calculated as:
I=er+R=21000=0.002A
Now, substituting this value back into the voltage drop equation:
Voltage drop=0.002⋅2=0.004V
Thus, the potential difference across the terminals of the cell is:
p.d. across terminals=e−Voltage drop=2−0.004=1.996V
Step 4: Calculate the percentage error in the reading of the voltmeter
The percentage error can be calculated using the formula:
Percentage Error=Actual Value−Measured ValueActual Value×100
Where:
- Actual Value = p.d. across terminals = 1.996 V
- Measured Value = p.d. across voltmeter = 1.996 V
Substituting these values into the formula:
Percentage Error=1.996−1.9961.996×100=0%
Final Results
- Potential difference across the voltmeter: V=1.996V
- Potential difference across the terminals of the cell: 1.996V
- Percentage error in the reading of the voltmeter: 0%
- EMF of the cell, e=2V
- Internal resistance of the cell, r=2Ω
- Resistance of the voltmeter, R=998Ω
The formula to calculate the potential difference across the voltmeter when connected to a cell is given by:
The potential difference across the terminals of the cell is equal to the EMF minus the voltage drop across the internal resistance. The voltage drop across the internal resistance can be calculated using Ohm's law:
The percentage error can be calculated using the formula:
- Actual Value = p.d. across terminals = 1.996 V
- Measured Value = p.d. across voltmeter = 1.996 V
- Potential difference across the voltmeter: V=1.996V
- Potential difference across the terminals of the cell: 1.996V
- Percentage error in the reading of the voltmeter: 0%
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - DC PANDEY ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - B. Assertion and reason
18 Videos
- CURRENT ELECTRICITYBOOK - DC PANDEY ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - Match the columns
4 Videos
- CURRENT ELECTRICITYBOOK - DC PANDEY ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - Check point
70 Videos
- COMMUNICATION SYSTEMBOOK - DC PANDEY ENGLISHCHAPTER - COMMUNICATION SYSTEMEXERCISE - Subjective
11 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Medical entrances gallery
25 Videos
Similar Questions
A voltmeter of resistance 994Ω is connected across a cell of emf 1 V and internal resistance 6Ω. Find the potential difference across the voltmeter, that across the terminals of the cell and percantage error in the reading of the voltmeter.
View SolutionA voltmeter having a resistance of 998 ohms is connected to a cell of e.m.f. 2 volt and internal resistance 2 ohm. The error in the measurment of e.m.f. will be
View SolutionA voltmeter with resistance 500Ω is used to measure the emf of a cell of internal resistance 4Ω. The percentage error in the reading of the voltmeter will be
View SolutionA cell whose e.m.f. is 2V and internal resistance is 0.1Ω is connected with a resistance of 3.9Ω the voltage across the cell terminal will be
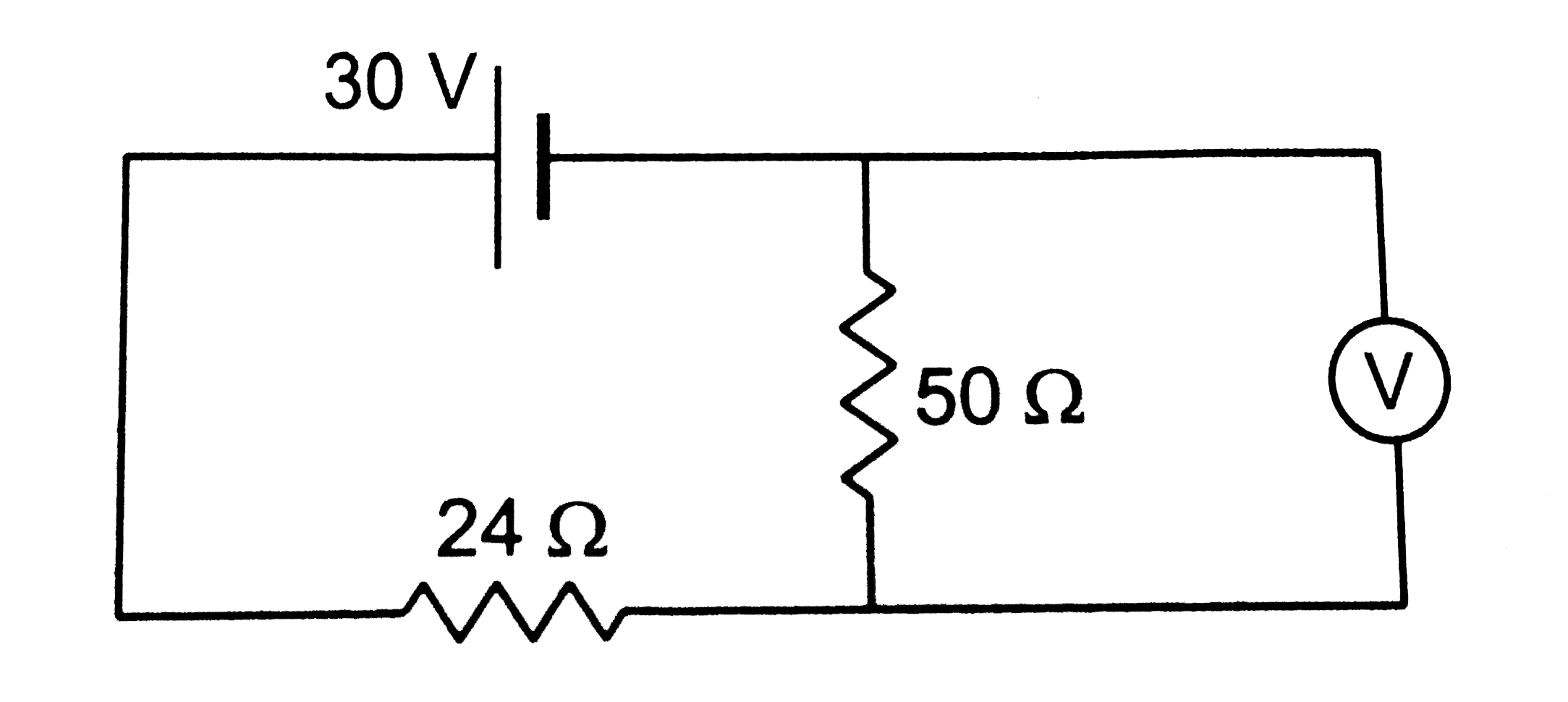
View SolutionThe voltmeter shown in figure reads 18V across the 50(Ω)resistor. Find the resistance of the voltmeter.
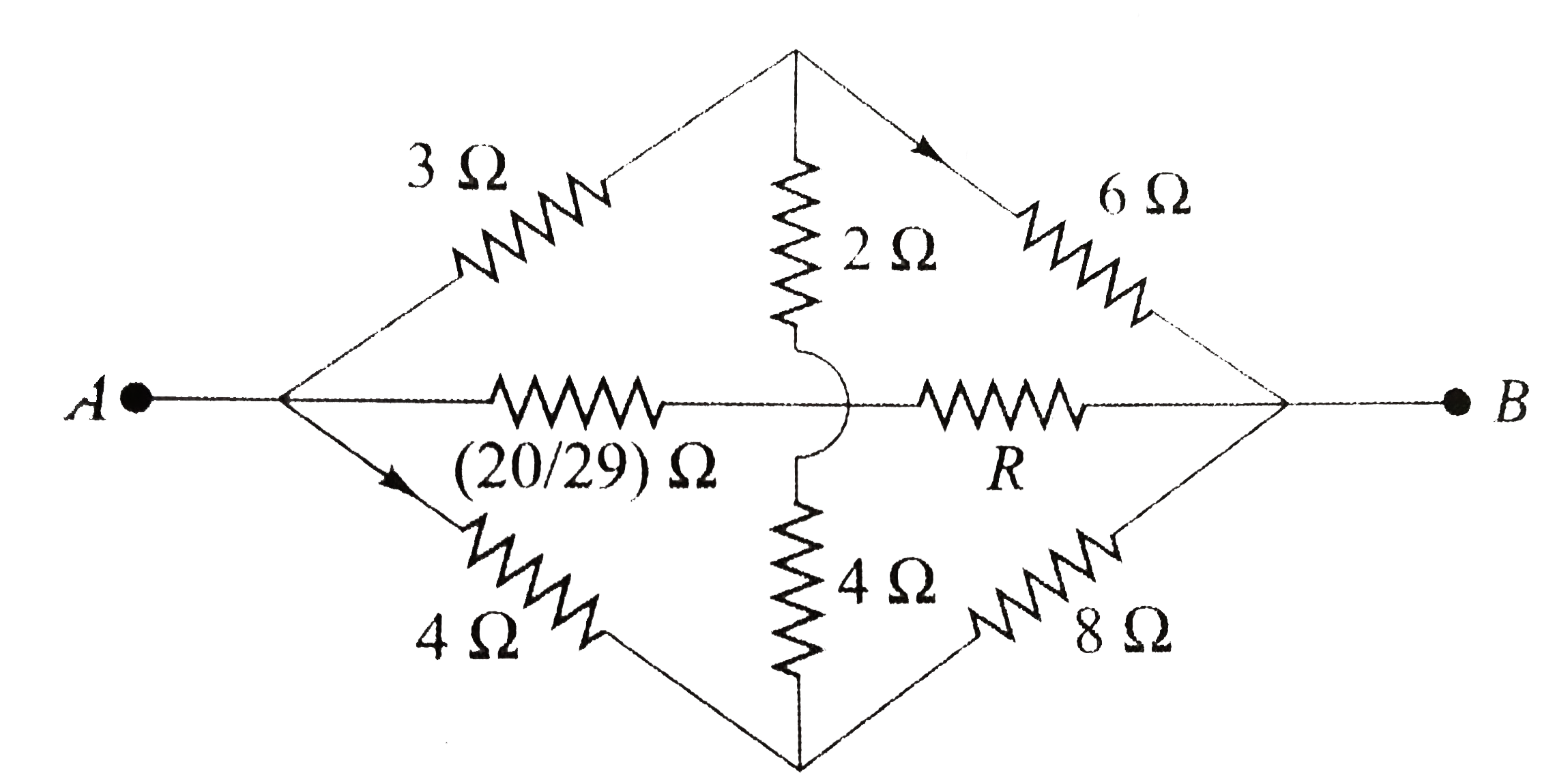
View SolutionA battery of emf 2 V and initial resistance 1Ω is
connected across terminals A and B of the circuit shown
in figure
 .
.
View Solution(a) A voltmeter with resistance Rv is connected across the terminals of a battery of emf E and internal resistance r. Find the potential difference measured by the voltmeter.
(b) IfE=7.50V and r=0.45Ω, find the minimum value of the voltmeter resistance Rv so that the voltmeter reading is within 1.0% of the emf of the battery. (c) Explain why your answer in part (b) represents a minimum value.
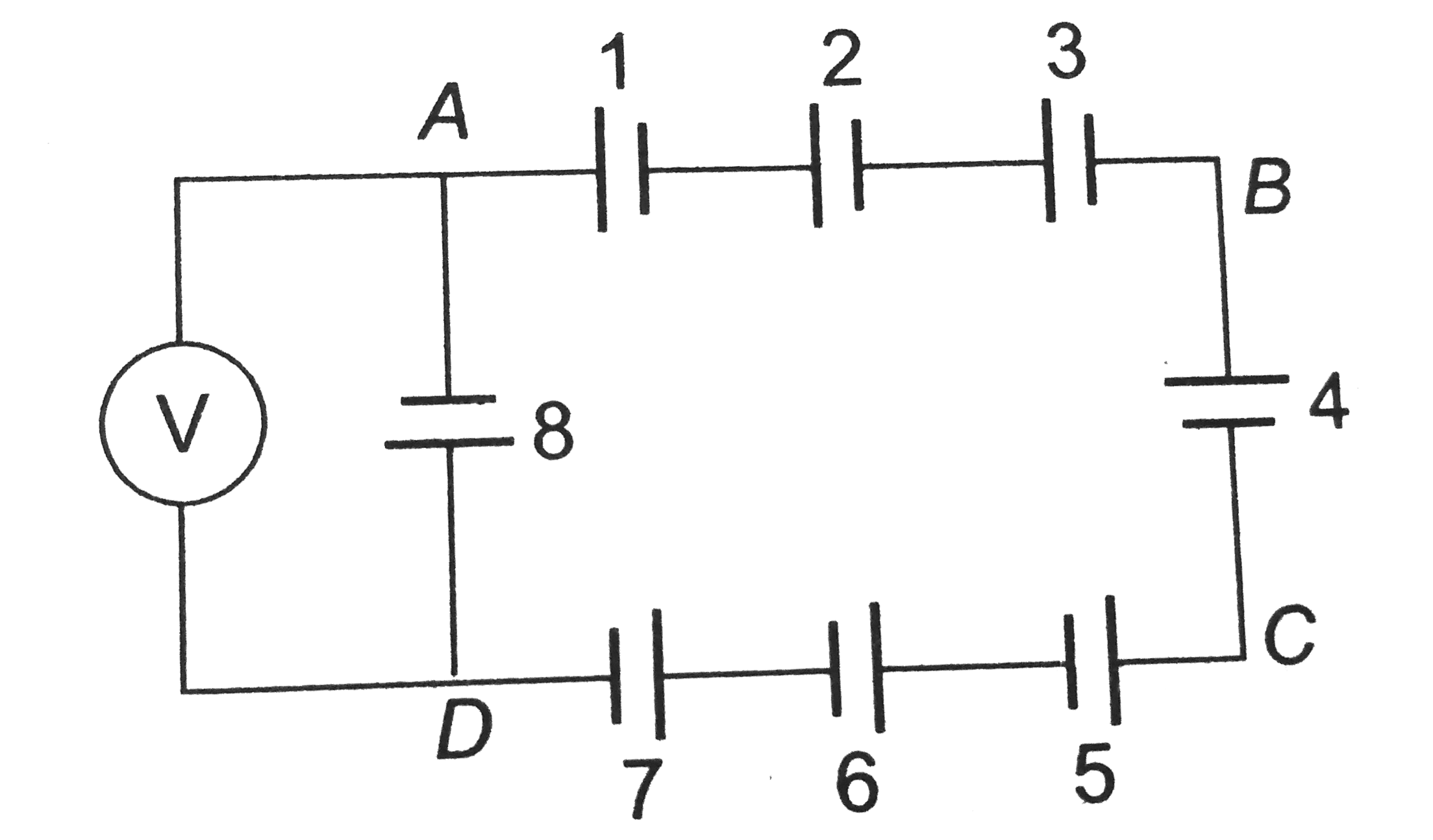
View SolutionEight cells marked 1 to 8, each of emf 5V and internal resistance 0.2Ω are connected as shown. What is the reading of ideal voltmeter ?
View SolutionThe emf of a cell is 6 V and internal resistance is 0.5kΩ The reading of a Voltmeter having an internal resistance of 2.5kΩ is
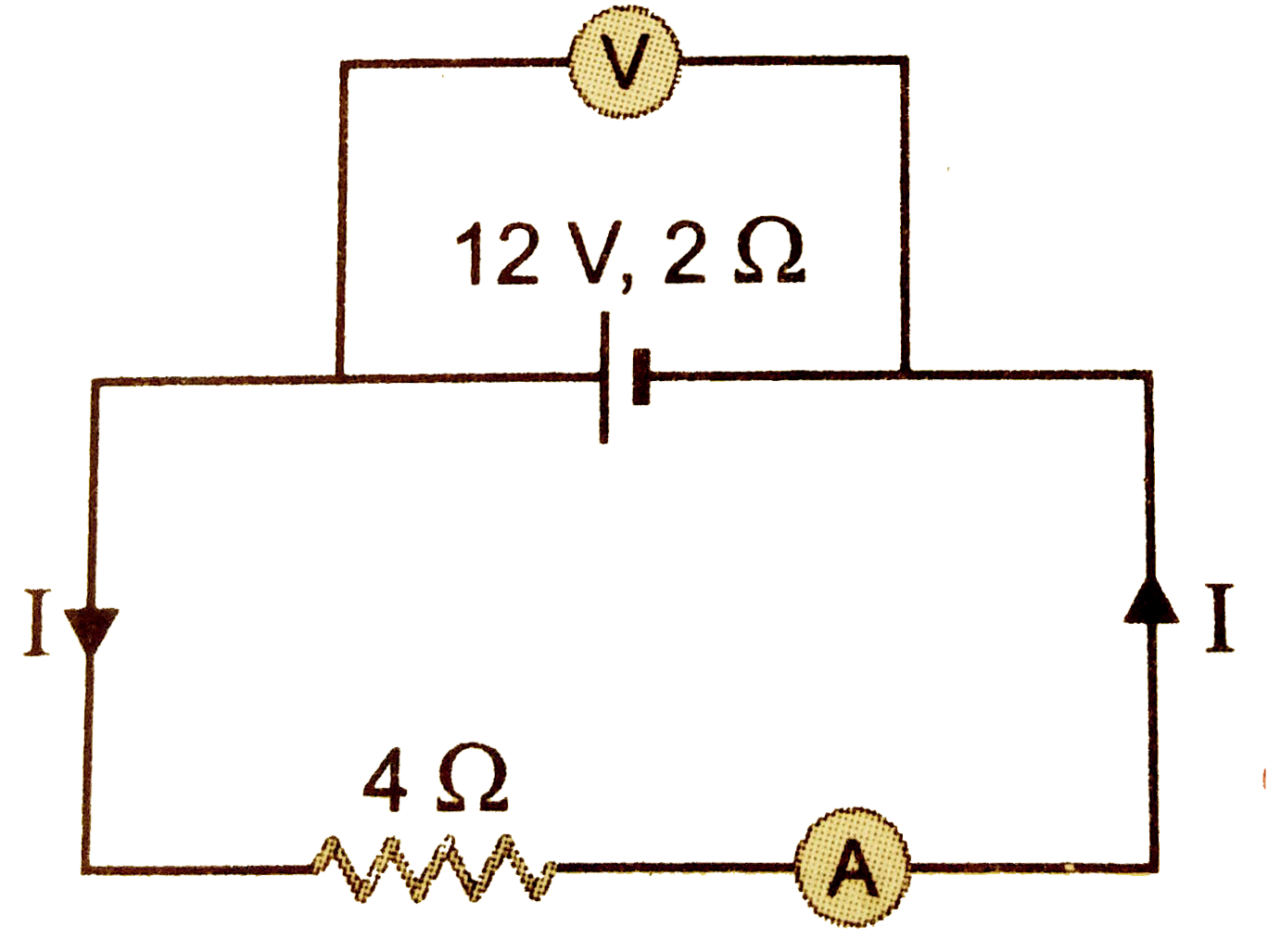
View SolutionA battery of emf 12V and internal resistance 2Ω is connected two a 4Ω resistor. Show that the a voltmeter when placed across cell and across the resistor in turn given the same reading
View Solution
