Three resistance P,Q,R each of 2Ω and an unknown resistance S from the four amrs of a Wheatstone's bridge circuit. When a resistance of 6Ω is connected in parallel to S the bridge gets balanced. What is the value of S ?
2Ω
3Ω
6Ω
1Ω
The correct Answer is:B
To solve the problem, we will follow these steps:
Step 1: Understand the Wheatstone Bridge Condition
In a Wheatstone bridge, the bridge is balanced when the ratio of the resistances in one arm is equal to the ratio of the resistances in the other arm. The condition for balance is given by:
PQ=RS′
Where S′ is the equivalent resistance of S when a 6Ω resistor is connected in parallel with it.
Step 2: Assign Values to the Known Resistors
Given that:
- P=2Ω
- Q=2Ω
- R=2Ω
Step 3: Set Up the Equation for Balance
Substituting the known values into the balance condition:
22=2S′
This simplifies to:
1=2S′
From this, we can find S′:
S′=2Ω
Step 4: Find the Equivalent Resistance S′
The equivalent resistance S′ of the resistors S and 6Ω in parallel is given by the formula:
S′=S⋅6S+6
Step 5: Set Up the Equation
Now we can set the equation we derived for S′ equal to 2Ω:
S⋅6S+6=2
Step 6: Cross Multiply to Solve for S
Cross multiplying gives us:
S⋅6=2(S+6)
Expanding this:
6S=2S+12
Step 7: Rearranging the Equation
Rearranging the equation to isolate S:
6S−2S=12
This simplifies to:
4S=12
Step 8: Solve for S
Dividing both sides by 4 gives:
S=3Ω
Final Answer
The value of the unknown resistance S is:
3Ω
---
In a Wheatstone bridge, the bridge is balanced when the ratio of the resistances in one arm is equal to the ratio of the resistances in the other arm. The condition for balance is given by:
Given that:
- P=2Ω
- Q=2Ω
- R=2Ω
Substituting the known values into the balance condition:
The equivalent resistance S′ of the resistors S and 6Ω in parallel is given by the formula:
Now we can set the equation we derived for S′ equal to 2Ω:
Cross multiplying gives us:
Rearranging the equation to isolate S:
Dividing both sides by 4 gives:
The value of the unknown resistance S is:
Topper's Solved these Questions
- CURRENT ELECTRICITYBook:DC PANDEY ENGLISHChapter:CURRENT ELECTRICITYExercise:B. Assertion and reason
Explore 18 Videos
- CURRENT ELECTRICITYBook:DC PANDEY ENGLISHChapter:CURRENT ELECTRICITYExercise:Match the columns
Explore 4 Videos
- CURRENT ELECTRICITYBook:DC PANDEY ENGLISHChapter:CURRENT ELECTRICITYExercise:Check point
Explore 70 Videos
- COMMUNICATION SYSTEMBook:DC PANDEY ENGLISHChapter:COMMUNICATION SYSTEMExercise:Subjective
Explore 11 Videos
- ELECTROMAGNETIC INDUCTIONBook:DC PANDEY ENGLISHChapter:ELECTROMAGNETIC INDUCTIONExercise:Medical entrances gallery
Explore 25 Videos
Similar Questions
Four resistors each of resistance 2 Ω are connected in parallel. What is the effective resistance ?
View SolutionThe resistance of each arm of the wheat stone bridge is 10Ω. A resistance of 10Ω is connected in series with galvanometer then the equivalent resistance across the battery will be:-
View Solution
Knowledge Check
Four resistances of 3Ω,3Ω,3Ωand4Ω respectively are used to form a Wheatstone bridge. The 4Ω resistance is short circuited with a resistance R in order to get bridge balanced. The value of R will be
A10ΩB11ΩC12ΩD13Ω
In a meter bridge, the null point is found at a distance of 40 cm from A. If now a resistance of 12 Ω is connected in parallel with S, the null point occurs at 60 cm from A. Determine the value of R.
View SolutionIn a metre bridge the null point is found at a distance of 35 cm from A. If now a resistance of 10Ω is connected in parallel with S, the null point occurs at 50 cm. Determine the values of R and S.
View SolutionThe resistance in the left and right gaps of a balanced meter bridge are R1 and R1. The balanced point is 50cm. If a resistance of 24Ω is connected in parallel to R2, the balance point is 70cm. The value of R1 or R2 is.
View SolutionA 6Ω resistance is connected in the left gap of a meter bridge. In the second gap 3Ω and 6Ω are joined in parallel. The balance point of the bridge is at _______
View SolutionIn a meter bridge, the null point is found at a distance of 40cm from A. If a resistance of 12Ω in connected in parallel with S the null point occurs at 50.0cm from A Determine the value of R and S

View SolutionIn a meter bridge,
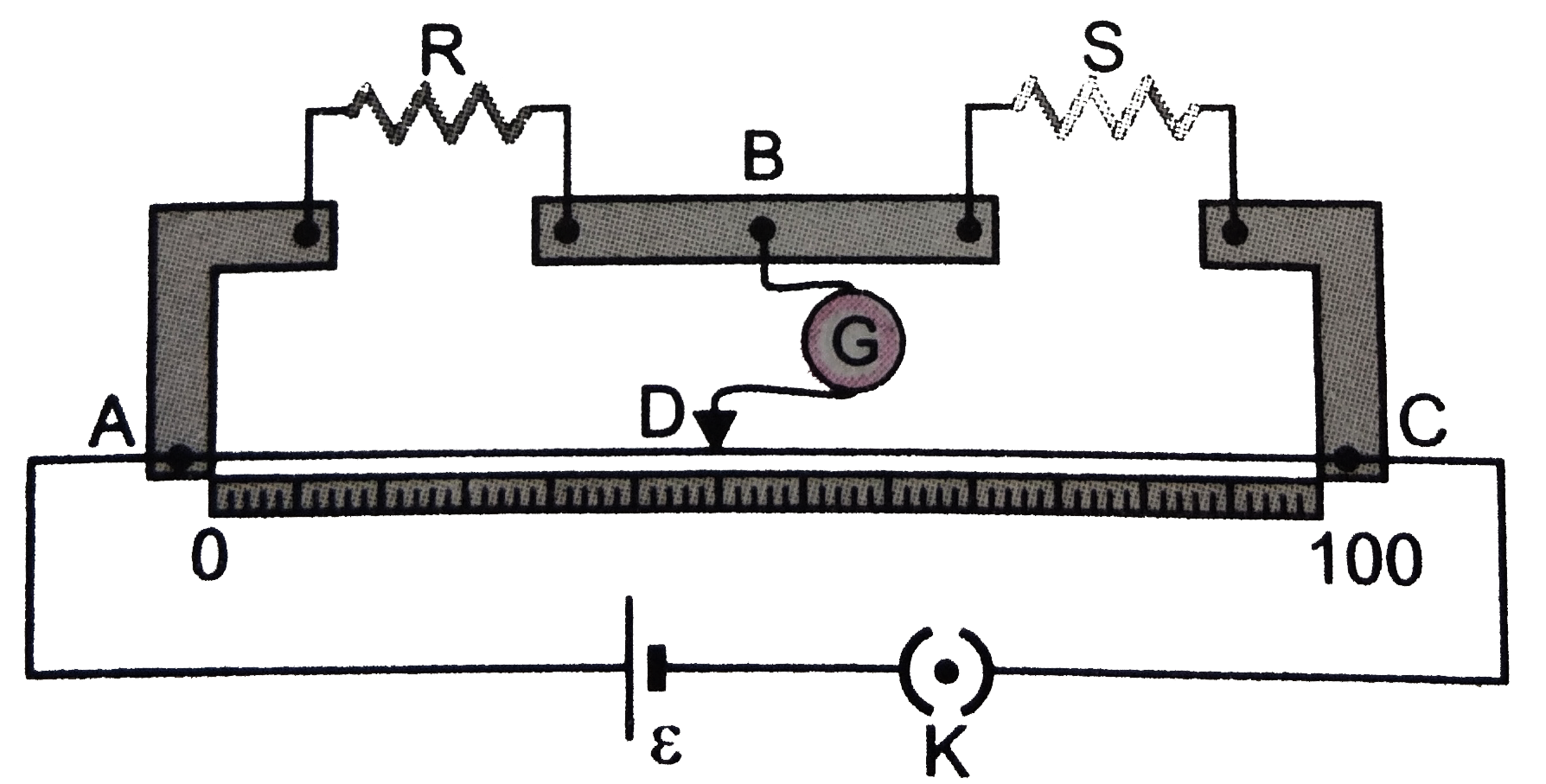
the null points is found at a distance of 33.7 cm from A. If now a resistance of 12Ω is connected in parallel with S, the null point occurs at 51.9 cm. Determine the values of R and S.
View SolutionFour resistors A, and D form a Wheatstone bridge.
The bridge is balanced , when C = 100Ω If A and B are interchanged the bridge balances for C=121Ω. The value of D is
View Solution