A wire of length 2 m carrying a current of 1 A is bend to form a circle. The magnetic moment of the coil is (in Am2)
2π
π/2
π/4
1/π
The correct Answer is:D
To find the magnetic moment of a circular coil formed by bending a wire of length 2 m carrying a current of 1 A, we can follow these steps:
Step 1: Determine the radius of the circle
The length of the wire is equal to the circumference of the circle formed when the wire is bent. The formula for the circumference C of a circle is given by:
C=2πr
where r is the radius of the circle. Given that the length of the wire L=2m, we can set up the equation:
2=2πr
Step 2: Solve for the radius r
Rearranging the equation to solve for r:
r=22π=1πm
Step 3: Calculate the area A of the circular loop
The area A of a circle is given by the formula:
A=πr2
Substituting the value of r:
A=π(1π)2=π⋅1π2=1πm2
Step 4: Calculate the magnetic moment M
The magnetic moment M of a coil is given by the formula:
M=I×A
where I is the current flowing through the wire. Given I=1A:
M=1×1π=1πAm2
Final Answer
The magnetic moment of the coil is:
M=1πAm2
---
The length of the wire is equal to the circumference of the circle formed when the wire is bent. The formula for the circumference C of a circle is given by:
Rearranging the equation to solve for r:
The area A of a circle is given by the formula:
The magnetic moment M of a coil is given by the formula:
The magnetic moment of the coil is:
Topper's Solved these Questions
- MAGNETIC FIELD AND FORCESBOOK - DC PANDEY ENGLISHCHAPTER - MAGNETIC FIELD AND FORCESEXERCISE - Assertion and reason
20 Videos
- MAGNETIC FIELD AND FORCESBOOK - DC PANDEY ENGLISHCHAPTER - MAGNETIC FIELD AND FORCESEXERCISE - Match the following
4 Videos
- MAGNETIC FIELD AND FORCESBOOK - DC PANDEY ENGLISHCHAPTER - MAGNETIC FIELD AND FORCESEXERCISE - Check point
54 Videos
- INTERFERENCE AND DIFFRACTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - INTERFERENCE AND DIFFRACTION OF LIGHTEXERCISE - Level 2 Subjective
5 Videos
- MAGNETICSBOOK - DC PANDEY ENGLISHCHAPTER - MAGNETICSEXERCISE - MCQ_TYPE
1 Videos
Similar Questions
A wire of length l meter carrying current i ampere is bent in form of circle. Magnetic moment is
View SolutionA wire of length Lmetre , carrying a current Iampere is bent in the form of a circle . The magnitude of its magnetic moment is …………. MKSunits .
View SolutionA thin wire of length l is carrying a constant current. The wire is bent to form a circular coil. If radius of the coil, thus formed, is equal to R and number of turns in it is equal to n, then which of the following graphs represent (s) variation of magnetic field induction (B) at centre of the coil
View SolutionA thin wire of length l is carrying a constant current. The wire is bent to form a circular coil. If radius of the coil, thus formed, is equal to R and number of turns in it is equal to n, then which of the following graphs represent (s) variation of magnetic field induction (B) at centre of the coil
View SolutionA conductor of length L and carrying current i is bent to form a coil of two turns.Magnetic field at the centre of the coil will be
View SolutionA wire of certain length carries a current l.It is bent to form a circle of one turn and the magnetic field at the centre is B1. If it is bent to form a coil of four turns, then magnetic field at centre is B2.The ratio of B1 and B2is
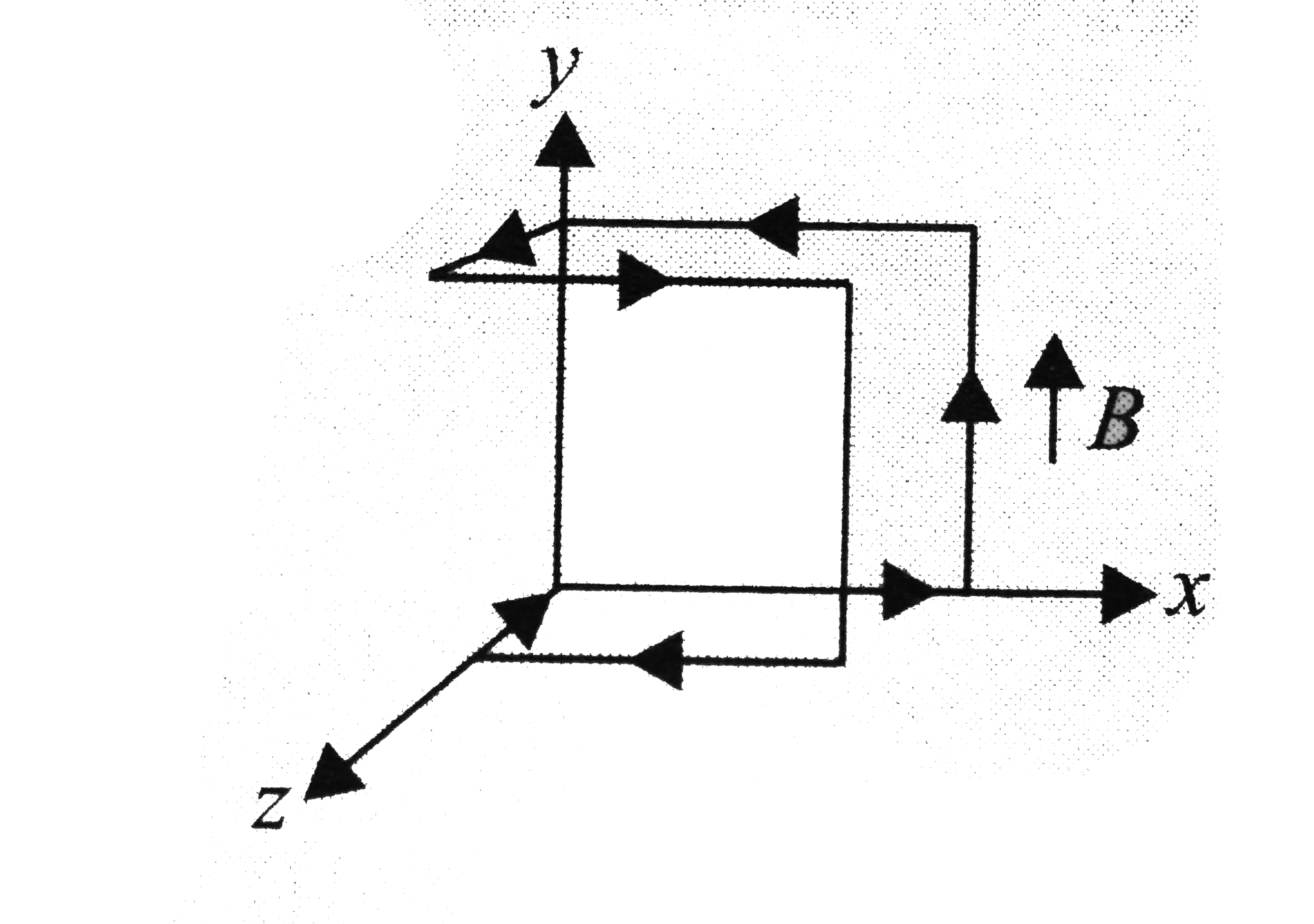
View SolutionFigure shows a bent coil with all edges of length 1m and carrying a current 1 A.
a. Find the magnetic moment of the loop.
b. Find the torque acting on the loop.
View SolutionA magnetic wire of dipole moment 4πAm2 is bent in the form of semicircle. The new magnetic moment is
View SolutionA straight wire of length 0.5 metre and carrying a current of 1.2 ampere is placed in a uniform magnetic field of induction 2 tesla. If the magnetic field is perpendicular to the length of the wire , the force acting on the wire is
View SolutionA uniform conducting wire of length length 10a and resistance R is wound up into four turn as a current carrying coil in the shape of equilateral triangle of side a. If current I is flowing 4 through the coil then the magnetic moment of the coil is
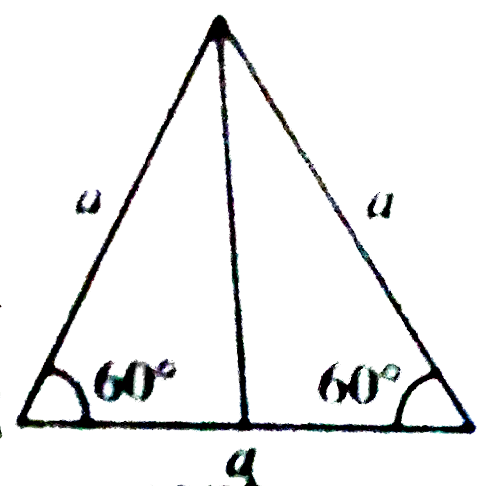
View Solution
