The time constant of C-R circuit is
1CR
CR
CR
RC
The correct Answer is:C
To find the time constant of a C-R (Capacitor-Resistor) circuit, we can follow these steps:
1. Understand the Components: In a C-R circuit, we have a capacitor (C) and a resistor (R) connected in series. The behavior of the circuit is characterized by how the capacitor charges and discharges through the resistor.
2. Definition of Time Constant: The time constant (τ) of a C-R circuit is defined as the product of the resistance (R) and the capacitance (C). Mathematically, this is expressed as:
τ=R×C
3. Units of Time Constant: The time constant τ has the same units as time. Since resistance (R) is measured in ohms (Ω) and capacitance (C) is measured in farads (F), the unit of τ will be seconds (s).
4. Current and Charge Equations: The equations governing the current (I) and charge (Q) in the circuit can be expressed as:
- For current:
I=I0e−tRC
- For charge:
Q=Q0(1−e−tRC)
Here, I0 and Q0 are the initial current and charge, respectively.
5. Conclusion: The time constant τ, which is equal to R multiplied by C, is a crucial parameter in determining how quickly the capacitor charges or discharges in the circuit.
Final Answer:
The time constant of a C-R circuit is given by:
τ=R×C
τ=R×C
- For current:
I=I0e−tRC
- For charge:
Q=Q0(1−e−tRC)
Here, I0 and Q0 are the initial current and charge, respectively.
The time constant of a C-R circuit is given by:
τ=R×C
Similar Questions
The time constant of L-R circuit is doubled if
View SolutionA charged capacitor is discharged through a resistance. The time constant of the circuit is η. Then the value of time constant for the power dissipated through the resistance will be
View SolutionIn an LR circuit, current at t=0 is 20A . After 2s it reduced to 18A. The time constant of the circuit is (in second)
View SolutionA coil carrying a steady current is short-circuited. The current in it decreases α times in time t0. The time constant of the circuit is
View SolutionTime constant of a C-R circuit is 2ln(2) second. Capacitor is discharged at time t=0. The ratio of charge on the capacitor at time t=2s and t=6s is
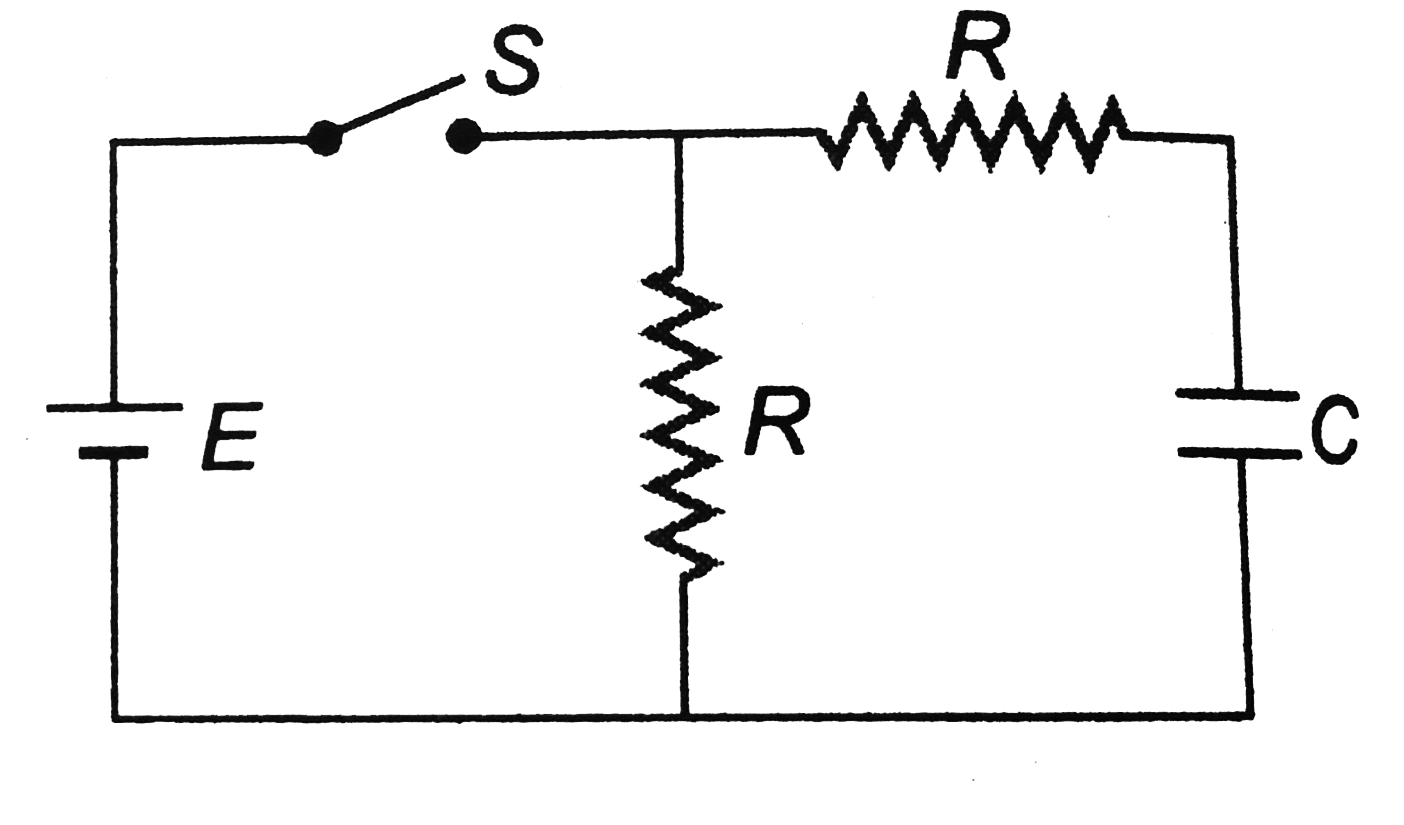
View SolutionIn the transient circuit shown, the time constant of the circuit is :
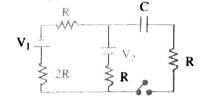
View SolutionA capacitor C is connected to two equal resistances as shown in the figure. Consider the following statemets
i. At the time of charging of capacitor time constant of the circuit is CR
ii. At the time of discharging of the capacitor the time constant of the circuit is CR
iii. At the time of discharging of the capacitor the time constant of the circuit is 2CR
iv At the time of charging of the capacitor the time constant of the circuit is 2CR
View SolutionThe current in an L−R circuit builds upto 3/4th of its steady state value in 4sec. Then the time constant of this circuit is
View SolutionIn a series L-R growth circuit, if maximum current and maximum voltage across inductor of inductane 3mH are 2A and 6V respectively, the the time constant of the circuit is
View SolutionIn a series L-R growth circuit, if maximum current and maximum voltage across inductor of inductane 3mH are 2A and 6V respectively, the the time constant of the circuit is
View Solution
