A horizontal rod is supported at both ends and loaded at the middle. If L and Y are length and Young's modulus repectively, then depression at the middle is directly proportional to
L
L2
Y
1Y
The correct Answer is:A
To solve the problem of finding the relationship between the depression (deflection) at the middle of a horizontal rod supported at both ends and loaded at the middle, we can use the formula for deflection in a beam. Here’s a step-by-step solution:
Step 1: Understand the Setup
We have a horizontal rod (beam) of length L that is supported at both ends. A load W is applied at the midpoint of the rod. This setup causes the rod to sag or deflect in the middle.
Hint: Visualize the rod and the load applied at its center to understand how it will sag.
Step 2: Identify the Relevant Formula
The deflection δ (depression at the middle) for a beam loaded at the center can be expressed using the following formula:
δ=WL348YI
where:
- W is the load applied at the center,
- L is the length of the beam,
- Y is Young's modulus of the material,
- I is the moment of inertia of the beam's cross-section.
Hint: Remember that the moment of inertia I depends on the shape of the beam's cross-section.
Step 3: Simplify the Formula
For a rectangular cross-section, the moment of inertia I can be expressed as:
I=bt312
where b is the breadth and t is the thickness of the beam. Substituting this into the deflection formula gives:
δ=WL348Y(bt312)=WL3⋅1248Ybt3=WL34Ybt3
Hint: Focus on how the parameters W, L, Y, b, and t affect the deflection.
Step 4: Analyze the Proportionality
From the derived formula, we can see that the deflection δ is directly proportional to L3 and W, and inversely proportional to Y, b, and t3:
δ∝WL3Ybt3
Hint: Identify which variables are directly proportional and which are inversely proportional.
Step 5: Conclusion
Since the question specifically asks for the relationship of depression at the middle with respect to Young's modulus Y, we conclude:
δ∝1Y
This means that the depression at the middle is directly proportional to 1Y.
Final Answer: The depression at the middle is directly proportional to 1Y.
We have a horizontal rod (beam) of length L that is supported at both ends. A load W is applied at the midpoint of the rod. This setup causes the rod to sag or deflect in the middle.
The deflection δ (depression at the middle) for a beam loaded at the center can be expressed using the following formula:
δ=WL348YI
where:
- W is the load applied at the center,
- L is the length of the beam,
- Y is Young's modulus of the material,
- I is the moment of inertia of the beam's cross-section.
For a rectangular cross-section, the moment of inertia I can be expressed as:
I=bt312
where b is the breadth and t is the thickness of the beam. Substituting this into the deflection formula gives:
δ=WL348Y(bt312)=WL3⋅1248Ybt3=WL34Ybt3
From the derived formula, we can see that the deflection δ is directly proportional to L3 and W, and inversely proportional to Y, b, and t3:
δ∝WL3Ybt3
Since the question specifically asks for the relationship of depression at the middle with respect to Young's modulus Y, we conclude:
δ∝1Y
This means that the depression at the middle is directly proportional to 1Y.
Topper's Solved these Questions
- MECHANICAL PROPERTIES OF SOLIDSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - Assignment (SECTION - C)
2 Videos
- MECHANICAL PROPERTIES OF SOLIDSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - Assignment (SECTION - D)
3 Videos
- MECHANICAL PROPERTIES OF SOLIDSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - Assignment (SECTION - A)
31 Videos
- MECHANICAL PROPERTIES OF FLUIDSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - SECTION - J
9 Videos
- Mock test 03BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - Mock test 03EXERCISE - EXAMPLE
44 Videos
Similar Questions
A metallic rod of length l and cross-sectional area A is made of a material of Young's modulus Y. If the rod is elongated by an amount y,then the work done is proportional to
View SolutionA uniform rod of mass m is hinged at one end and other end is connected to a light extensible string having length L.If the Youngs modulus and crosssectional area of the wire is Y and A respectively then the elongation in the string is
View Solution
Knowledge Check
A wire of length L and area of cross-section A, is stretched by a load. The elongation produced in the wire is I. If Y is the Young's modulus of the material of the wire, then the force constant of the wire is
AYLABYlACYALDYAl
A steel wire of length 2L and cross-section area A is stretched, wetl within elastic limit horizontally between two pillars, a mass m is suspended from the mid-point of wire such that depression at the middle is x. Strain in the wire is proportional to
View SolutionIf the depression d at the end of a loaded bar is given by d=Mgl33yi where M is the mass , l is the length and y is the young's modulus, then i has the dimensional formula
View SolutionA point mass m is suspended at the end of a massless wire of length l and cross section. If Y is the Young's modulus for the wire, obtain the frequency of oscillation for the simple harmonic motion along the vertical line.
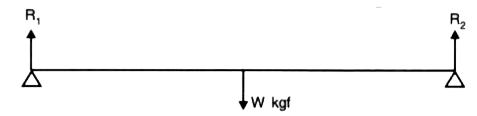
View SolutionIn Fig.1.35 a uniform bar of length l m is supported at its ends and loaded by a weight W kgf at its middle. In equilibrium find the reaction R1andR2 at the ends.
View SolutionA wire of length l and cross-sectional are A is suspended at one of its ends from a ceiling. What will be its strain energy due to its own weight, if the density and Young's modulus of the material of the wire be d and Y?
View SolutionThe cable of a uniformly loaded suspension bridge hangs in the form of a parabola. The roadway which is horizontal and 100m long is supported by vertical wires attached to the cable, the longest wire being 30m and the shortest being 6m. Find the length of the supporting wire attached to the roadway 18m from the middle.
View SolutionA block of mass M is suspended from a wire of length L, area of cross-section A and Young's modulus Y. The elastic potential energy stored in the wire is
View Solution