A a certain instant of time a ballon is moving downwards with speed of 5m/s. It has a constant retardation of 5m/s2. A man in the ballon drops a stone at that instant. It hits the ground after 2s. Then the speed with which the stone hits the ground is.
The correct Answer is:99 m/s
To solve the problem step by step, let's break it down:
Step 1: Understand the initial conditions
The balloon is moving downwards with a speed of 5 m/s. This means that the initial velocity of the stone when it is dropped is also 5 m/s downwards.
Step 2: Identify the acceleration
The balloon has a constant retardation (deceleration) of 5 m/s². This means that the acceleration of the balloon is -5 m/s² (upwards). However, once the stone is dropped, it will only experience the acceleration due to gravity, which is approximately -10 m/s² (downwards).
Step 3: Set up the equation for the stone's motion
When the stone is dropped, its initial velocity (u) is -5 m/s (downwards), and it will accelerate downwards due to gravity at -10 m/s². We need to find the final velocity (v) of the stone after it falls for 2 seconds (t = 2 s).
Step 4: Apply the kinematic equation
We will use the kinematic equation:
v=u+at
Where:
- v = final velocity
- u = initial velocity
- a = acceleration
- t = time
Step 5: Substitute the values into the equation
Here, u=−5m/s, a=−10m/s2, and t=2s.
Substituting these values into the equation:
v=−5+(−10)×2
v=−5−20
v=−25m/s
Step 6: Interpret the result
The negative sign indicates that the stone is moving downwards. Therefore, the speed with which the stone hits the ground is 25 m/s.
Final Answer
The speed with which the stone hits the ground is 25 m/s.
---
The balloon is moving downwards with a speed of 5 m/s. This means that the initial velocity of the stone when it is dropped is also 5 m/s downwards.
The balloon has a constant retardation (deceleration) of 5 m/s². This means that the acceleration of the balloon is -5 m/s² (upwards). However, once the stone is dropped, it will only experience the acceleration due to gravity, which is approximately -10 m/s² (downwards).
When the stone is dropped, its initial velocity (u) is -5 m/s (downwards), and it will accelerate downwards due to gravity at -10 m/s². We need to find the final velocity (v) of the stone after it falls for 2 seconds (t = 2 s).
We will use the kinematic equation:
v=u+at
Where:
- v = final velocity
- u = initial velocity
- a = acceleration
- t = time
Here, u=−5m/s, a=−10m/s2, and t=2s.
v=−5+(−10)×2
v=−5−20
v=−25m/s
The negative sign indicates that the stone is moving downwards. Therefore, the speed with which the stone hits the ground is 25 m/s.
The speed with which the stone hits the ground is 25 m/s.
Topper's Solved these Questions
- MOTION IN A PLANEBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOTION IN A PLANEEXERCISE - Assignement section -A Objective (one option is correct)
50 Videos
- MOTION IN A PLANEBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOTION IN A PLANEEXERCISE - Assignement section -B Objective (one option is correct)
29 Videos
- MOTION IN A PLANEBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOTION IN A PLANEEXERCISE - llustration 1 :
1 Videos
- MOCK_TEST_17BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOCK_TEST_17EXERCISE - Example
15 Videos
- MOTION IN A STRAIGHT LINEBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - ASSIGNMENT (SECTION - D)
15 Videos
Similar Questions
Knowledge Check
In the question number 52, the speed with which the stone hits the ground is
A15 m s−1B90 m s−1C99 m s−1D49 m s−1
A man in a balloon throws a stone downwards with a speed of 5m/s with respect to balloon. The balloon is moving upwards with a constant acceleration of 5m/s2. Then velocity of the stone relative to the man after 2 seconds is.

View SolutionA stone is dropped from top of a cliff.It is seen to hit the ground below after 4.2 s. How high is the cliff?
View SolutionA balloon is moving upwards with velocity 10m/s. It releases a stone which comes down to the ground in 11s. The height of the balloon from the ground at the moment when the stone was dropped is (g=10m/s2)
View SolutionA balloon is moving along with constant upward acceleration of 1m/s2. A stone is thrown from the balloon downwards with speed 10m/s with respect to the balloon. At the time of projectile balloon is at height 120m from the ground and is moving with speed 20m/s. Find the time required to fall on the ground by the stone after the projection.
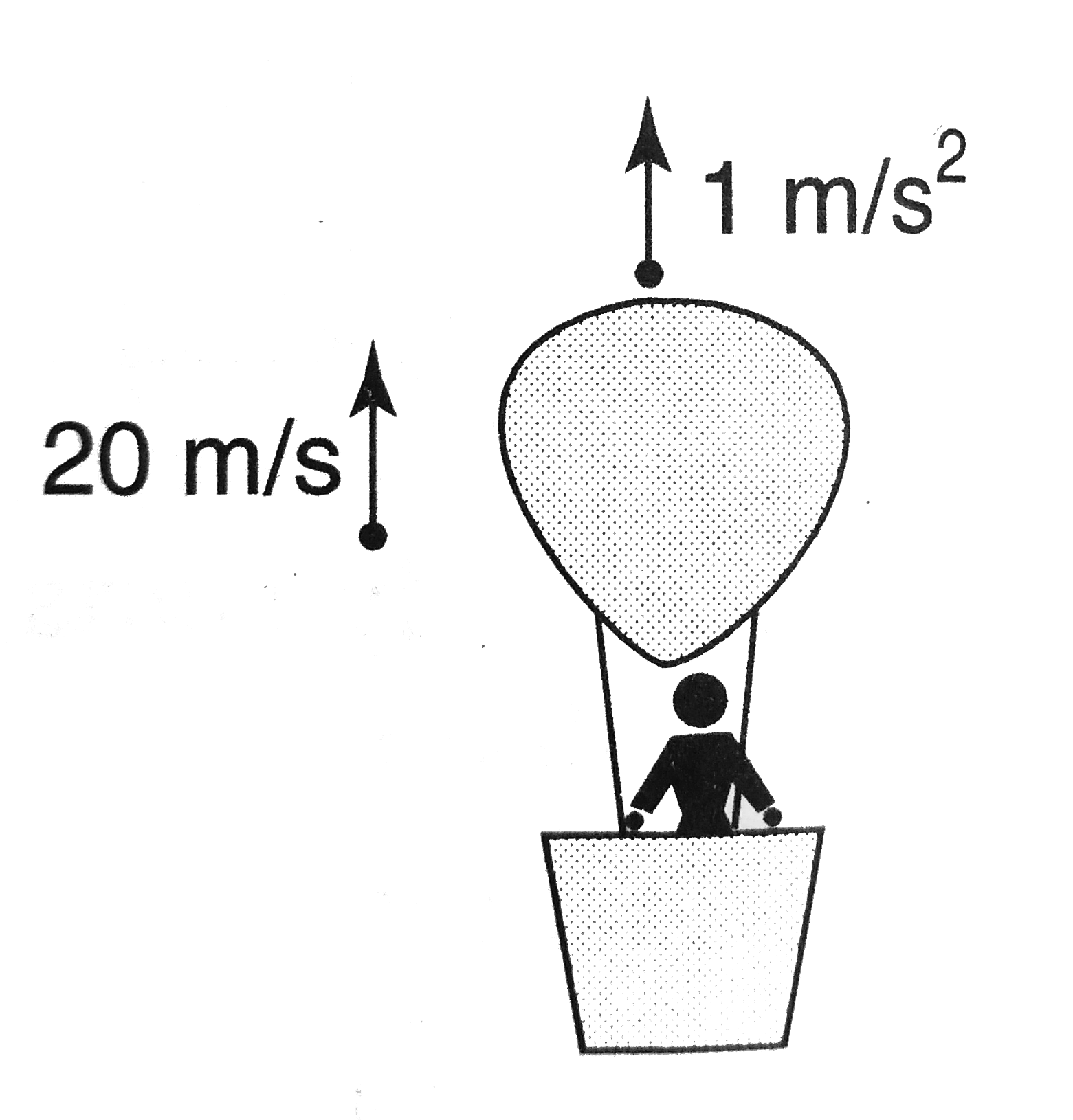
View SolutionA ball of mass 2 kg is projected horizontally with a velocity 20m/s from a building of height 15m. The speed with which body hits the ground is :
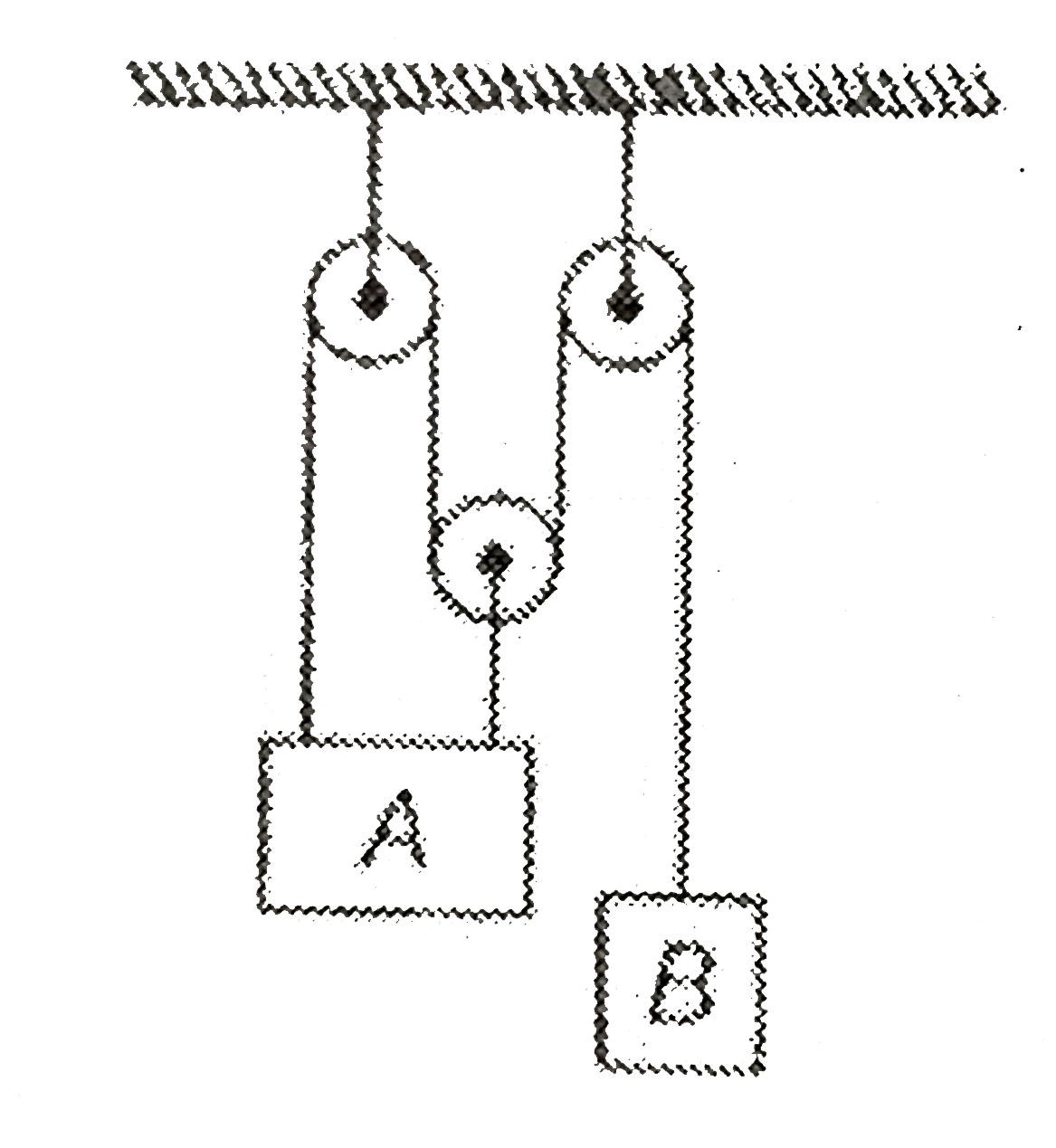
View SolutionAt a given instant. A is moving with velocity of 5m/s upward. What is velocity of B at that time:
View SolutionA person drops a stone from a building of height 20 m. At the same instant the front end of a truck passes below the building moving with constant acceleration of 1m/s2 and velocity of 2m/s at that instant. Length of the truck if the stone just misses to hit its rear part is :-
View Solution
