The angualr displacement of an object having uniform cirular motion is rad in every 3s. Find its frequency of revolution.
The correct Answer is:
To solve the problem, we need to find the frequency of revolution of an object in uniform circular motion given its angular displacement and the time taken for that displacement.
1. Identify the Given Values:
- Angular displacement (θ) = π/4 radians
- Time (t) = 3 seconds
2. Calculate Angular Velocity (ω):
- Angular velocity (ω) is defined as the angular displacement divided by the time taken.
- Formula:
ω=θt
- Substituting the values:
ω=π/43=π12 radians/second
3. Relate Angular Velocity to Frequency:
- The relationship between angular velocity (ω) and frequency (f) is given by:
ω=2πf
- Rearranging the formula to find frequency:
f=ω2π
4. Substitute the Value of Angular Velocity:
- Now, substitute the value of ω we calculated:
f=π/122π
- Simplifying this:
f=π12⋅12π=124 Hz
5. Final Answer:
- The frequency of revolution is:
f=124 Hz
- Angular displacement (θ) = π/4 radians
- Time (t) = 3 seconds
- Angular velocity (ω) is defined as the angular displacement divided by the time taken.
- Formula:
ω=θt
- Substituting the values:
ω=π/43=π12 radians/second
- The relationship between angular velocity (ω) and frequency (f) is given by:
ω=2πf
- Rearranging the formula to find frequency:
f=ω2π
- Now, substitute the value of ω we calculated:
f=π/122π
- Simplifying this:
f=π12⋅12π=124 Hz
- The frequency of revolution is:
f=124 Hz
Topper's Solved these Questions
- MOTION IN A PLANEBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOTION IN A PLANEEXERCISE - Assignement section -A Objective (one option is correct)
50 Videos
- MOTION IN A PLANEBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOTION IN A PLANEEXERCISE - Assignement section -B Objective (one option is correct)
29 Videos
- MOTION IN A PLANEBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOTION IN A PLANEEXERCISE - llustration 1 :
1 Videos
- MOCK_TEST_17BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOCK_TEST_17EXERCISE - Example
15 Videos
- MOTION IN A STRAIGHT LINEBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - ASSIGNMENT (SECTION - D)
15 Videos
Similar Questions
If the displacement of an object during its motion is 100 m in a time interval of 20 s, then calculate the average velocity of the object with which it was moving through out his journey.
View SolutionA particle goes uniformly in circular motion with an angualr speed π8rads−1 . What is its time period ?
View SolutionA particle is executing SHM given by x=Asin(πt+ϕ). The initial displacement of particle is 1cm and its initial velocity is πcm/sec. Find the amplitude of motion and initial phase of the particle.
View SolutionAssertion : The total displacement moved by a point located on the periphery of a wheel of radius R in one revolution is 2πR. Wheel is rolling.
Reason : In rolling motion of a wheel, every point on its periphery comes in contact with the surface once in one revolution.
View SolutionThe displacement x (in metre ) of a particle in, simple harmonic motion is related to time t ( in second ) as
x=0.01cos(πt+π4)
the frequency of the motion will be
View SolutionA particle executing simple harmonic motion of amplitude 5 cm has maximum speed of 31.4 cm / s . The frequency of its oscillation is
View SolutionThe displacement of a particel moving with uniform acceleration after r's given by s = 10t+4.9t2 ? What is the magnitude the final velocity at t = 3 s and the uniform acceleration .
View SolutionThe displacement (s)- velocity ( v ) graph of a particle if it starts moving from rest with a uniform acceleration which is parallel to its instantaneous direction of motion is
View SolutionA particle executes simple harmonic motion about the point x=0. At time t=0, it has displacement x=2cm and zero velocity. If the frequency of motion is 0.25s−1, find (a) the period, (b) angular frequency, ( c) the amplitude, (d) maximum speed, (e) the displacement from the mean position at t=3sand (f) the velocity at t=3s.
View SolutionAn object of mass 0.8kg is attached to one end of a spring and the system is set into simple harmonic motion. The displacement x of the object as a fuction of time is shown in the figure. With the aid of the data, determine
(a) the amplitude A of the motion,
(b) the angular frequency ω,
( c) the spring constant K,
(d) the speed of the object at t=1.0s and
(e) the magnitude of the object's acceleration at t=1.0s.
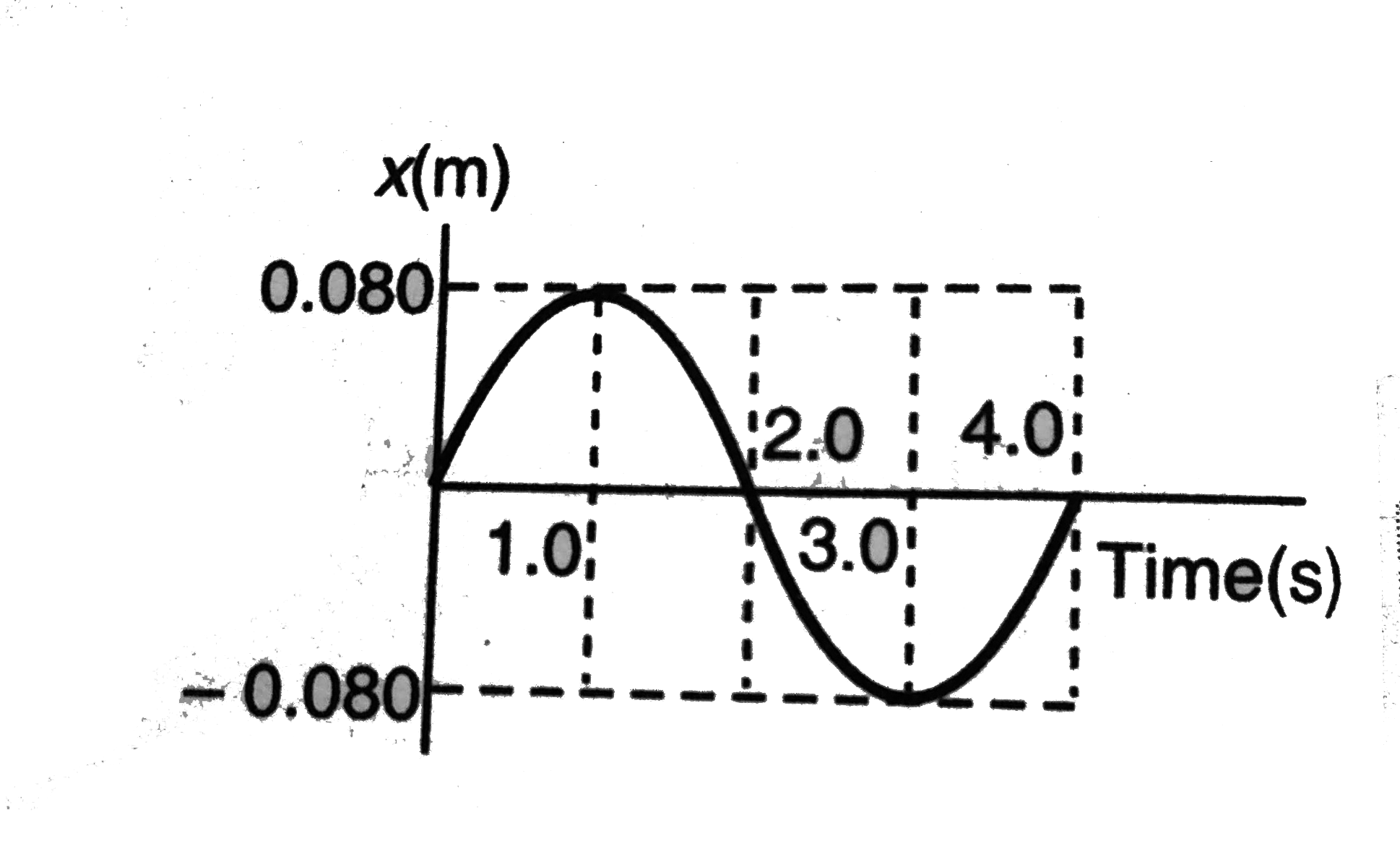
View Solution
