If two liquids of same mass but densities ρ1 and ρ2 respectively are mixed, then the density of the mixture is:
p−1p2m1+m2m1p2+m2P1
p−1p2m1+m2p1+p2
2p1p2p1+p2
m1p1+m2p2(m1+m2)
The correct Answer is:A
To find the density of a mixture of two liquids with the same mass but different densities, we can follow these steps:
Step 1: Define the Variables
Let:
- Mass of each liquid = m
- Density of the first liquid = ρ1
- Density of the second liquid = ρ2
Step 2: Calculate the Volumes of Each Liquid
Using the formula for density, which is given by:
Density=MassVolume
We can rearrange this to find the volume:
Volume=MassDensity
So, the volumes of the two liquids are:
- Volume of the first liquid, V1=mρ1
- Volume of the second liquid, V2=mρ2
Step 3: Calculate the Total Volume of the Mixture
The total volume of the mixture V is the sum of the volumes of the two liquids:
V=V1+V2=mρ1+mρ2
Step 4: Simplify the Total Volume
Factoring out m from the total volume:
V=m(1ρ1+1ρ2)
Step 5: Calculate the Total Mass of the Mixture
The total mass of the mixture M is:
M=m+m=2m
Step 6: Calculate the Density of the Mixture
The density ρ of the mixture is given by:
ρ=Total MassTotal Volume=MV
Substituting the values we found:
ρ=2mm(1ρ1+1ρ2)
Step 7: Simplify the Expression for Density
Cancelling m from the numerator and denominator:
ρ=21ρ1+1ρ2
This can be rewritten as:
ρ=2ρ1ρ2ρ1+ρ2
Conclusion
Thus, the density of the mixture of the two liquids is:
ρ=2ρ1ρ2ρ1+ρ2
Let:
- Mass of each liquid = m
- Density of the first liquid = ρ1
- Density of the second liquid = ρ2
Using the formula for density, which is given by:
Density=MassVolume
We can rearrange this to find the volume:
Volume=MassDensity
So, the volumes of the two liquids are:
- Volume of the first liquid, V1=mρ1
- Volume of the second liquid, V2=mρ2
The total volume of the mixture V is the sum of the volumes of the two liquids:
V=V1+V2=mρ1+mρ2
Factoring out m from the total volume:
V=m(1ρ1+1ρ2)
The total mass of the mixture M is:
M=m+m=2m
The density ρ of the mixture is given by:
ρ=Total MassTotal Volume=MV
Substituting the values we found:
ρ=2mm(1ρ1+1ρ2)
Cancelling m from the numerator and denominator:
ρ=21ρ1+1ρ2
This can be rewritten as:
ρ=2ρ1ρ2ρ1+ρ2
Thus, the density of the mixture of the two liquids is:
ρ=2ρ1ρ2ρ1+ρ2
Topper's Solved these Questions
Similar Questions
If two liquids of same volume but different densities ρ1 and ρ2 are mixed, then the density of the mixture is:
View SolutionIf equal masses of two liquids of densities d1andd2 are mixed together, the density of the mixture is
View SolutionEqual masses of two substance of densities ρ1andρ2 are mixed together. What is the density of the mixture?
View SolutionTwo liquids of densities 2ρ and ρ having their volumes in the ratio 3:2 are mixed together. Density of the mixture will be
View SolutionFig shows the same block of wood floating in three different liquids A,B and C of densities ρ1,ρ2 and ρ3 respectively. Which liquid has the highest density? Give reason for your answer.

View SolutionTwo substances of densities ρ1 and ρ2 are mixed in equal volume and the relative density of mixture is 4. When they are mixed in equal masses, the relative density of the mixture is 3. the values of ρ1 and ρ2 are:
View SolutionEqual masses of water and a liquid of density 2g/cm3 are mixed together. The density of mixture is:
View SolutionA vessel is filled with two different liquids of densities ρand2ρ respectively as shown in the figure. The velocity of flow of liquid through a hole at height h2 from bottom is
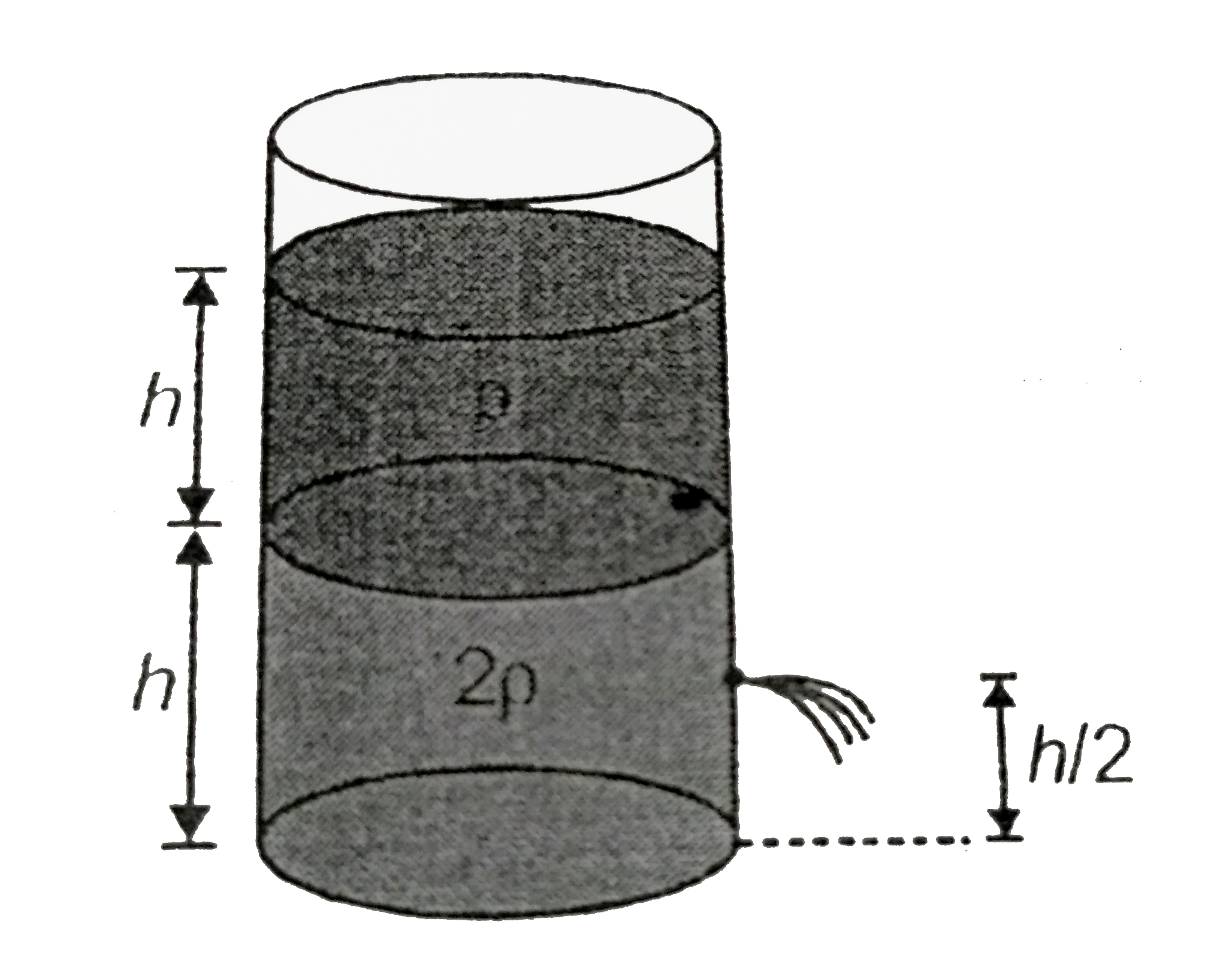
View Solution
A jar filled with two non-mixing liquid 1 and 2 having densities ρ1 and ρ2 respectively. A solid ball, made of a material of density ρ3 is dropped in the jar. It come to equilibrium in the position shown in the figure. Which of the following is true for ρ1,ρ2 and ρ3?
View SolutionA solid sphere having volume V and density ρ floats at the interface of two immiscible liquids of densites ρ1 and ρ2 respectively. If ρ1<ρ<ρ2, then the ratio of volume of the parts of the sphere in upper and lower liquid is
View Solution
