One mole of a diatomic gas is heated under a "kibolinsky process" in which gas pressure is temperature controlled according to law P=45CT3/2. Where C is kibolinsky constant. In this process, gas is heated by 300K temperature. Answer the following questions.
Under above a process the molar specific heat of gas is:
R2
R
2R
None of these
To find the molar specific heat of the diatomic gas under the given "kibolinsky process," we can follow these steps:
Step 1: Understand the Process
The problem states that the pressure P is related to temperature T by the equation:
P=45CT32
where C is a constant. We also know that the gas is heated by 300K.
Step 2: Use the Ideal Gas Law
The ideal gas law states:
PV=nRT
For one mole of gas (n=1), we can rewrite this as:
PV=RT
Step 3: Relate Pressure and Volume
From the given relation for pressure, we can express volume V in terms of temperature T:
V=RTP
Substituting the expression for P:
V=RT45CT32=5RT4CT32=5R4CT−12
Step 4: Determine Work Done
The work done W during a process where PVη=constant is given by:
W=nRΔT1−η
Here, η=3 (as per the power of T in the pressure equation). Thus:
W=1⋅R⋅3001−3=300R−2=−150R
Step 5: Change in Internal Energy
For a diatomic gas, the change in internal energy ΔU is given by:
ΔU=F2nRΔT
For a diatomic gas, F=5 (degrees of freedom):
ΔU=52⋅1⋅R⋅300=1500R2=750R
Step 6: Apply the First Law of Thermodynamics
According to the first law of thermodynamics:
Q=ΔU+W
Substituting the values we found:
Q=750R−150R=600R
Step 7: Calculate Molar Specific Heat
The molar specific heat Cm is defined as the heat added per mole per unit temperature change:
Cm=QΔT=600R300=2R
Final Answer
Thus, the molar specific heat of the gas under the given process is:
Cm=2R
The problem states that the pressure P is related to temperature T by the equation:
P=45CT32
where C is a constant. We also know that the gas is heated by 300K.
The ideal gas law states:
PV=nRT
For one mole of gas (n=1), we can rewrite this as:
PV=RT
From the given relation for pressure, we can express volume V in terms of temperature T:
V=RTP
Substituting the expression for P:
V=RT45CT32=5RT4CT32=5R4CT−12
The work done W during a process where PVη=constant is given by:
W=nRΔT1−η
Here, η=3 (as per the power of T in the pressure equation). Thus:
W=1⋅R⋅3001−3=300R−2=−150R
For a diatomic gas, the change in internal energy ΔU is given by:
ΔU=F2nRΔT
For a diatomic gas, F=5 (degrees of freedom):
ΔU=52⋅1⋅R⋅300=1500R2=750R
According to the first law of thermodynamics:
Q=ΔU+W
Substituting the values we found:
Q=750R−150R=600R
The molar specific heat Cm is defined as the heat added per mole per unit temperature change:
Cm=QΔT=600R300=2R
Thus, the molar specific heat of the gas under the given process is:
Cm=2R
Topper's Solved these Questions
Similar Questions
One mole of a diatomic gas is heated under a "kibolinsky process" in which gas pressure is temperature controlled according to law P=45CT3/2. Where C is kibolinsky constant. In this process, gas is heated by 300K temperature. Answer the following questions.
The amount of workdonw by gas under above heating is:
View SolutionOne mole of a diatomic gas is heated under a "kibolinsky process" in which gas pressure is temperature controlled according to law P=45CT3/2. Where C is kibolinsky constant. In this process, gas is heated by 300K temperature. Answer the following questions.
The amount of heat supplied to the gas under above heating is:
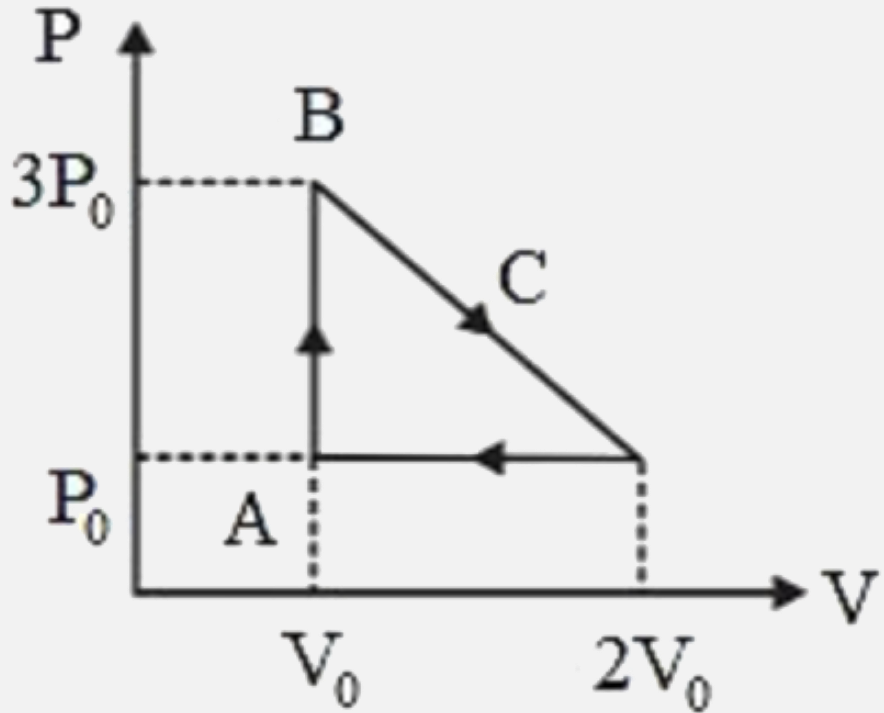
View SolutionOne mole of monoatomic gas is taken round the cyclic process. Heat absorbed by gas in process AB is
View SolutionOne mole of a diatomic gas undergoes a thermodynamic process, whose process equation is P∝V2. The molar specific heat of the gas is
View SolutionFor a process, relation between temperature and volume is TV3=constant. If a monoatomic gas follows this process, find molar specific heat for this process
View SolutionOne mole of an ideal monoatomic gas is taken through a polytropic process P2T = constant. The heat required to increase the temperature of the gas by ΔT is
View SolutionTwo moles of an ideal mono-atomic gas undergoes a thermodynamic process in which the molar heat capacity 'C' of the gas depends on absolute temperature as C=RTT0, where R is gas consant and T0 is the initial temperature of the gas. (V0 is the initial of the gas). Then answer the following questions:
The equation of process is
View SolutionOne moles of a monatomic gas is mixed with three moles of a diatomic gas. The molar specific heat of the mixture at constant volume is
View SolutionOne mole of an ideal monatomic gas undergoes the process p=αT1/2, where α is a constant.
(a) Find the work done by the gas if its temperature increases by 50K.
(b) Also, find the molar specific heat of the gas.
View SolutionOne mole of an ideal monatomic gas undergoes the process p=αT1/2, where α is a constant.
(a) Find the work done by the gas if its temperature increases by 50K.
(b) Also, find the molar specific heat of the gas.
View Solution
