_S01_020_Q01.png)
In a given U-tube (open at one-end) find out relation between p and pa
Given d2=2×13.6gm/cm3,d1=13.6gm/cm3
Pressure in a liquid at same level is same i.e., at A−A−,
Pa+d2yg+xd1g=P
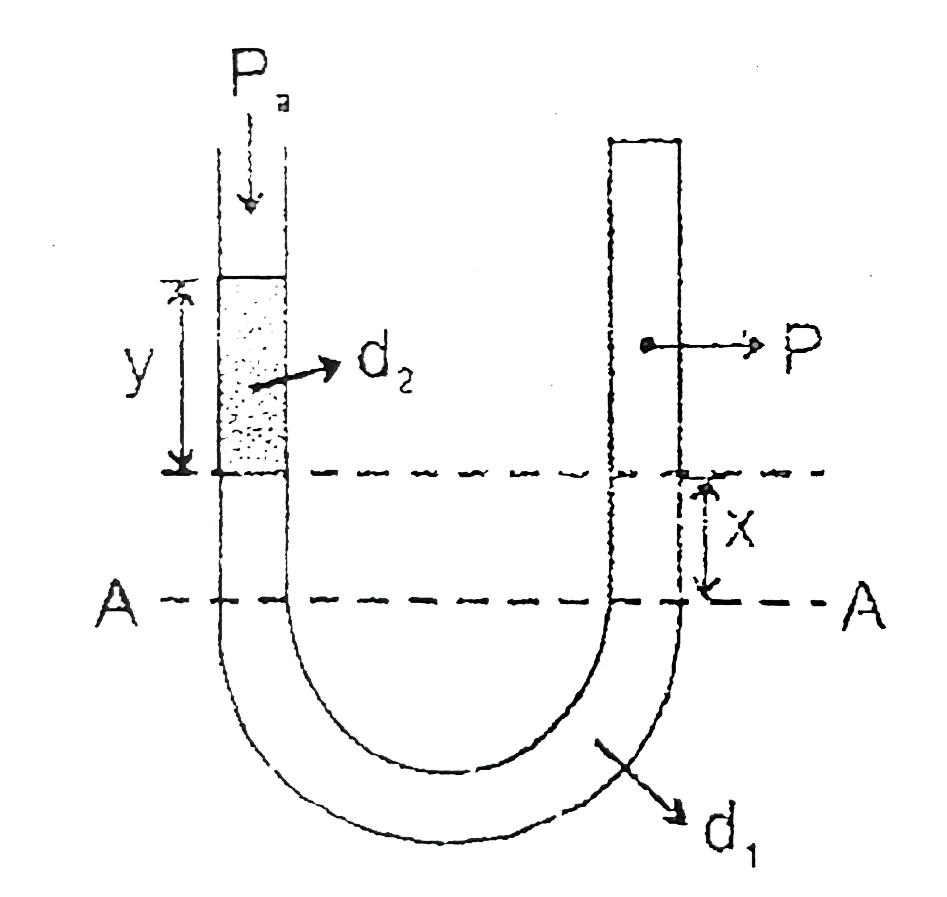
In C.G.S.
Pa+13.6×2×25×g+13.6×26×g=P
Pa+13.6×g[50+26]=P
2Pa=P[Pa=13.6×g×76]
Topper's Solved these Questions
- BOOK - RESONANCE ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Board Level Exercise18videos
FLUID MECHANICS
- BOOK - RESONANCE ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Exercise- 1 PART - I Section (A)3videos
FLUID MECHANICS
- BOOK - RESONANCE ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Advanced Level Problems8videos
FLUID MECHANICS
- BOOK - RESONANCE ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Exercise52videos
ELECTROSTATICS
- BOOK - RESONANCE ENGLISHCHAPTER - FULL TEST 1EXERCISE - Exercise30videos
FULL TEST 1
Similar Questions
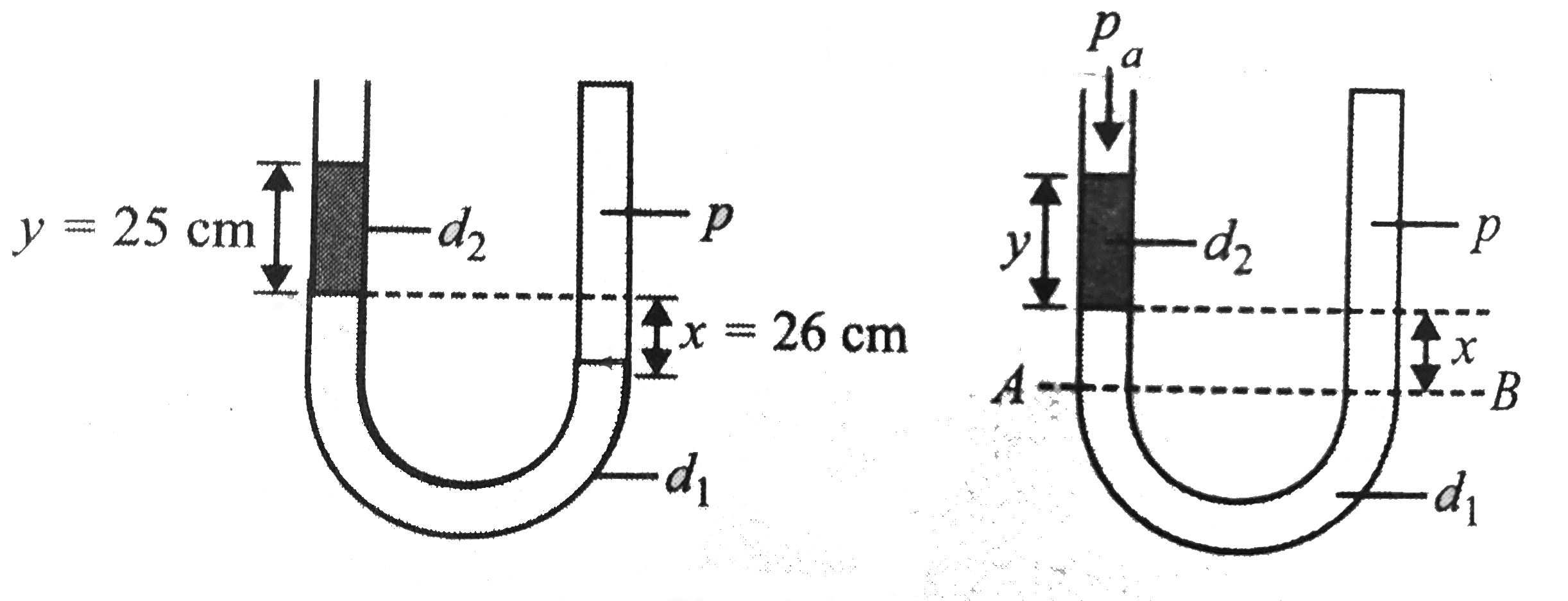
In a given U tube open at left end and closed at right end, if the pessure above the liquid the right arm is p find the value of p. Given d2=2×13.6g/cm3,d1=13.6g/cm3. Atmosphere pressure pa=76cm of Hg.
 View Solution
View SolutionIf a is the G.M. of 2 and14, find a.
View SolutionFind the reflection of the point P(-1,3) in the line x = 2
View SolutionA cube of 1 kg is floating at the interface of two liquids of density ρ1=3/4gm/cm3andρ2=2.5gm/cm3 The cube has (3/5)th part in ρ2 and (2/5)th part in ρ1 .Find the density of the cube .
 View Solution
View SolutionA cubical block of iron of side13.6cm is floating in mercury in a vessel .Water is poured in the vessel until it just covers the iron block. What is the height of water column? (ρHg=13.6gmcm3, ρFe=7.2gmcm3, ρH_2O=1gmcm3)
View SolutionAn ideal gas is collected by downward displacement of water. Select the correct expression for Pgas according to the diagram [dHg=13.6g/cm3]:
 View Solution
View SolutionThe nucleus of an atom is spherical. The relation between radius of the nucleus and mass number A is given by 1.25×10−13×A13cm. If radius of atom is oneÅ and the mass number is 64, then the fraction of the atomic volume that is occupied by the nucleus is (x)×10−13. Calculate x
View SolutionGiven two independent events A and B such that P(A)=0.3 and P(B)=0.6 . Find: P(A∩B)
View SolutionGiven two independent events A and B such that P(A)=0.3 and P(B)=0.6 . Find: P(A∪B)
View SolutionGiven two independent events A and B such that P(A)=0.3 and P(B)=0.6 . Find: P(B/A)
View Solution

