Water and liquid are filled up behind a square wall of side l. Find out
a. pressures at A,B and C
b. forces in part AB and BC
c. total force and point of application of force (neglect atmospheric pressure in every calculation)
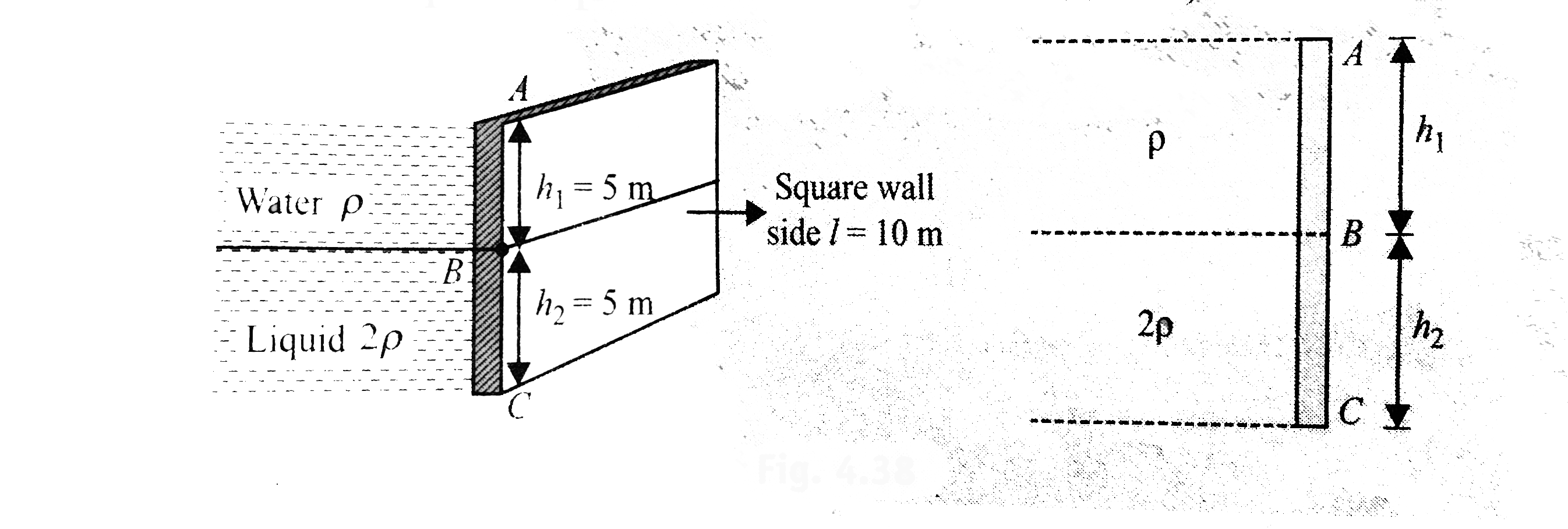
(a) As there is no liquid above 'A',
So pressure at A,PA=0
Pressure at B,PB=ρgh1
Pressure at C,PC=ρgh1+2ρgh2
(b) Force at A=0
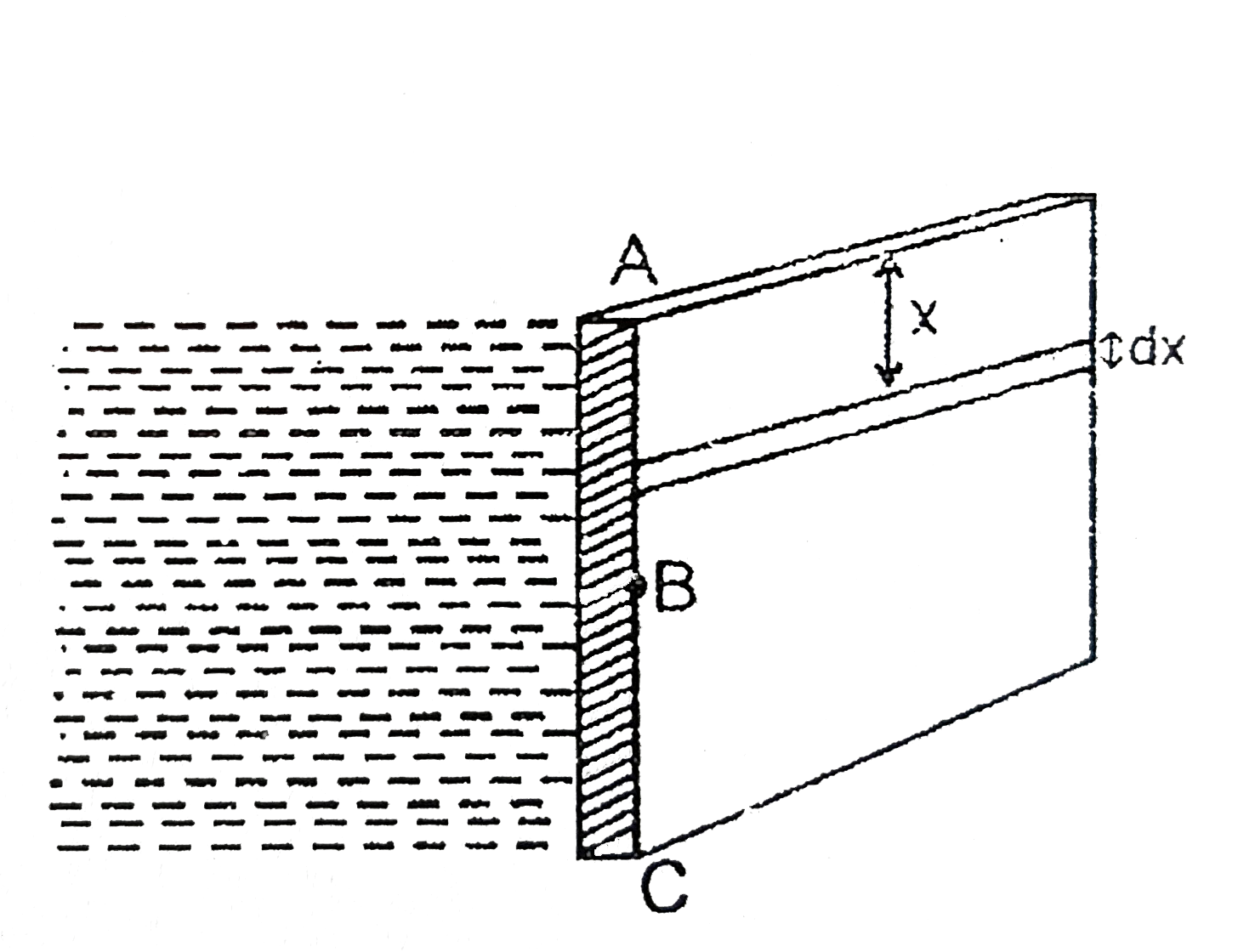
Take a strip of width 'dx' at a depth 'x' in part AB.
Pressure is equal to ρgx.
Force on strip =pressure×area
dF=ρpxldx
Total force upto B
F=h1∫0ρgxldx=ρgxlh212=1000×10×10×5×52
=1.25×108N
In part BC for force ltake a elementary strip of width dx in portion BC. Pressure is equal to
=ρgh1+2ρg(x−h1)
Force on elementry strip =pressure×area
dF=[ρgh1+2ρg(x−h1)]ldx
Total force on part BC
F=t∫h1[ρgh1+2ρg(x−h1)]ldx

=[ρgh1+2ρg[x22−h1x]]lh1l
=ρgh1h2l+2ρgl[l2−h212−h1l+h21]
ρgh1h2l+2ρgl2[l2+h21−2h1l]
ρgh1h2+ρgl(l−h1)2
ρgh2l[h1+h2]=ρgh2l2
=1000×10×5×10×10
5×106N
Topper's Solved these Questions
- FLUID MECHANICSBOOK - RESONANCE ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Board Level Exercise
18 Videos
- FLUID MECHANICSBOOK - RESONANCE ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Exercise- 1 PART - I Section (A)
3 Videos
- FLUID MECHANICSBOOK - RESONANCE ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Advanced Level Problems
8 Videos
- ELECTROSTATICSBOOK - RESONANCE ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Exercise
52 Videos
- FULL TEST 1BOOK - RESONANCE ENGLISHCHAPTER - FULL TEST 1EXERCISE - Exercise
30 Videos
Similar Questions
Water and liquid are filled up behind a square wall of side. Find out
a. pressures at A,B and C
b. forces in part AB and BC
c. total force and point of application of force (neglect atmospheric pressure in every calculation)
View Solution_S01_024_Q01.png)
Water and liquid is filled up behind a square wall of side l
Find out (a). Pressure at A, B and C
(b). Forces in part AB and BC
(c). Total force and point of application of force. (Neglect atmosphere pressure in every calculation.)
View Solution_S01_031_Q01.png)
In a given arrangement (a) find out velocity of water coming out of C
(b). Find out pressure A, B and C
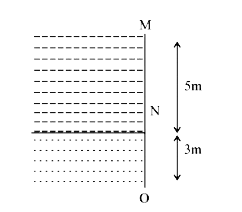
View SolutionTwo liquids of densities p1andp2(p2=6p1) are filled up behind a square wall of side 8 m as shown in figure. The liquids have height as shown. The ratio of the forces due to these liquids exerted on upper part MN to that at the lower part NO is (Assume that the liquids are not mixing)
View SolutionMercury is completely filled in a rectangular take of height 72 cm. The atmospheric pressure at the place is 72 cm. of Hg. Find the distance in cm of point of application from bottom of tank of the net force , on the inner surface of the side vertical wall of tank.
View SolutionFour charges of +q, +q +q and +q are placed at the corners A, B, C and D of s square. The resultant force on the charge at D
View SolutionTwo liquids of densities ρ1 and ρ2(ρ2=3ρ1) are filled up behind a square wall of side 6 m as shown in figure. Each liquid has a height of 3 m. The ratio of the forces due to these liquids exerted on upper part MN to that at the lower part NO is (Assume that the liquids are not mixing)2
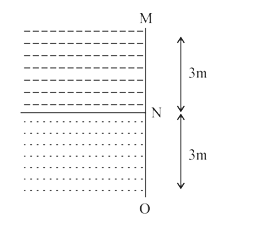
View SolutionTwo liquids of densities ρ1 and ρ2(ρ2=3ρ1) are filled up behind a square wall of side 6 m as shown in figure. Each liquid has a height of 3 m. The ratio of the forces due to these liquids exerted on upper part MN to that at the lower part NO is (Assume that the liquids are not mixing)2

View SolutionWater is filled in a rectangular tank of size 3m×2m×1m. a) Find the total force exerted by the water on the bottom surface of the tank. b) Consider a vertical side of area 2m×1m. Take a horizontal strip of wide δx metre in this side, situated at a depth of x metre from the surface of water.Find the force by the water on this strip. c) Find the torque of the force calculate in part b) about the bottom edge of this side. d) Find the total force by the water on this side. e) Find the total torque by the water on the side about the bottom edge. neglect the atmospheric pressure and take =10ms−2.
View SolutionName a machine for each of the following use :
(a) to multiple the force,
(b) to change the point of application of force,
(c) to change the direction of force,
(d) to obtain the gain in speed.
View Solution
