The capacitance of a parallel plate condenser is C0 If a dielectric of relative permittivity εr and thickness equal to one fourth the plate separation is placed between the plates, then its capacity becomes C. The value of CC0 will be -
5εr4εr+1
4εr3εr+1
3εr2εr+1
2εrεr+1
The correct Answer is:B
To solve the problem, we need to find the ratio CC0 where C is the capacitance of a parallel plate capacitor with a dielectric inserted, and C0 is the original capacitance without the dielectric.
1. Identify the Original Capacitance:
The original capacitance C0 of a parallel plate capacitor is given by the formula:
C0=Aϵ0D
where A is the area of the plates, ϵ0 is the permittivity of free space, and D is the separation between the plates.
Hint: Remember that capacitance is dependent on the area of the plates and the distance between them.
2. Insert the Dielectric:
A dielectric with relative permittivity ϵr and thickness D4 is inserted between the plates. The remaining distance without the dielectric will be D−D4=3D4.
3. Capacitance with Dielectric:
The capacitor can be viewed as two capacitors in series:
- Capacitor 1 (with dielectric):
C1=ϵrϵ0AD4=4ϵrϵ0AD
- Capacitor 2 (without dielectric):
C2=ϵ0A3D4=4ϵ0A3D
Hint: When a dielectric is inserted, the capacitance changes based on the thickness of the dielectric and the remaining air gap.
4. Calculate the Equivalent Capacitance:
The total capacitance C of the two capacitors in series is given by:
1C=1C1+1C2
Substituting the values of C1 and C2:
1C=D4ϵrϵ0A+3D4ϵ0A
Hint: When capacitors are in series, the reciprocal of the total capacitance is the sum of the reciprocals of the individual capacitances.
5. Combine the Terms:
Finding a common denominator:
1C=D4ϵ0A(1ϵr+3)
Thus,
C=4ϵ0AD(1ϵr+3)
6. Express C in Terms of C0:
We know that C0=Aϵ0D, so we can express C as:
C=C0⋅4ϵr1+3ϵr
7. Find the Ratio CC0:
Finally, the ratio CC0 becomes:
CC0=4ϵr1+3ϵr
Final Answer:
CC0=4ϵr1+3ϵr
The original capacitance C0 of a parallel plate capacitor is given by the formula:
C0=Aϵ0D
where A is the area of the plates, ϵ0 is the permittivity of free space, and D is the separation between the plates.
A dielectric with relative permittivity ϵr and thickness D4 is inserted between the plates. The remaining distance without the dielectric will be D−D4=3D4.
The capacitor can be viewed as two capacitors in series:
- Capacitor 1 (with dielectric):
C1=ϵrϵ0AD4=4ϵrϵ0AD
- Capacitor 2 (without dielectric):
C2=ϵ0A3D4=4ϵ0A3D
The total capacitance C of the two capacitors in series is given by:
1C=1C1+1C2
Substituting the values of C1 and C2:
1C=D4ϵrϵ0A+3D4ϵ0A
Finding a common denominator:
1C=D4ϵ0A(1ϵr+3)
Thus,
C=4ϵ0AD(1ϵr+3)
We know that C0=Aϵ0D, so we can express C as:
C=C0⋅4ϵr1+3ϵr
Finally, the ratio CC0 becomes:
CC0=4ϵr1+3ϵr
CC0=4ϵr1+3ϵr
Topper's Solved these Questions
- CAPACITANCEBOOK - RESONANCE ENGLISHCHAPTER - CAPACITANCEEXERCISE - Exercise - 3
26 Videos
- CAPACITANCEBOOK - RESONANCE ENGLISHCHAPTER - CAPACITANCEEXERCISE - High Level Problems
16 Videos
- CAPACITANCEBOOK - RESONANCE ENGLISHCHAPTER - CAPACITANCEEXERCISE - Exercise - 1
79 Videos
- ATOMIC PHYSICSBOOK - RESONANCE ENGLISHCHAPTER - ATOMIC PHYSICSEXERCISE - Advanved level problems
17 Videos
- COMMUNICATION SYSTEMSBOOK - RESONANCE ENGLISHCHAPTER - COMMUNICATION SYSTEMSEXERCISE - Exercise 3
13 Videos
Similar Questions
The capacity of a parallel plate condenser without any dielectric is C. If the distance between the plates is doubled and the space between the plates is filled with a substance of dielectric constant 3, the capacity of the condenser becomes:
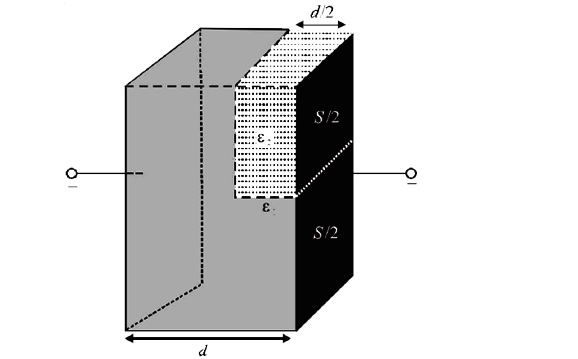
View SolutionA parallel plate capacitor having plates of area S and plate separation d, has capacitance C1 in air. When two dielectrics of different relative primitivities (ε1=2 and ε2=4) are introduced between the two plates as shown in the figure, the capacitance becomes C2. The ratio C2C1 is
View SolutionThe capacity of a parallel plate capacitor formed by the plates of same area A is 0.02μF with air as dielectric. Now one plate is replaced by a plate of area 2A and dielectric (k=2) is introduced between the plates, the capacity is
View SolutionA parallel plate capacitor having plates of area S and plate separation d, has capacitance C1 in air. When two dielectrics of different relative primitivities (ε1=2ε0 and ε2=4ε0) are introduced between the two plates as shown in the figure, the capacitance becomes C2. The ratio C2C1 is

View SolutionThe capacitance of a capacitor becomes4/3 times its original value if a dielectric slab of thickness t=d/2 is inserted between the plates (d is the separation between the plates). What is the dielectric constant of the slab?
View SolutionThe capacitance of a capacitor between4/3 times its original value if a dielectric slab of thickness t=d/2 is inserted between the plates (d is the separation between the plates). What is the dielectric consant of the slab?
View SolutionThe capacitance of a capacitor between4/3 times its original value if a dielectric slab of thickness t=d/2 is inserted between the plates (d is the separation between the plates). What is the dielectric consant of the slab?
View SolutionThe capacity and the energy stored in a parallel plate condenser with air between its plates are respectively C0 and W0. If the air is replaced by glass (dielectric constant =5) between the plates, the capacity of the plates and the energy stored in it will respectively be
View SolutionThe two metallic plates of radius r are placed at a distance d apart and its capacity is C. If a plate of radius r/2 and thickness dof dielectric constant 6 is placed between the plates of the condenser, then its capacity will be
View SolutionSeparation between the plates of a parallel plate capacitor is d and the area of each plates is A. When a slab of material of dielectric constant k and thickness t(t<d) is introduced between the plates. Its capacitance becomes
View Solution
