A dielectric slab of dielectric constant 3 is inserted into an uncharged capacitor C1. The slab covers the entire volume of the capacitor now, this capacitor and an identical uncharged capacitor C2, with air between the plates are placed in series and connected to a battery. after the capacitor are fully charged, the ratio of the electric field inside them, E1E∘ is:
(a)13
(b)1
(c)3
(d)9
The correct Answer is:A
To solve the problem, we need to find the ratio of the electric fields inside two capacitors, one with a dielectric slab and the other with air between the plates, after they are connected in series to a battery.
1. Identify the Capacitors:
- Let C1 be the capacitor with a dielectric slab of dielectric constant K=3.
- Let C2 be the identical capacitor with air between the plates.
2. Capacitance Calculation:
- The capacitance of C1 with the dielectric is given by:
C1=K⋅C=3C
- The capacitance of C2 (with air) remains:
C2=C
3. Total Capacitance in Series:
- The total capacitance Ctotal for capacitors in series is given by:
1Ctotal=1C1+1C2
- Substituting the values:
1Ctotal=13C+1C=1+33C=43C
- Therefore, the total capacitance is:
Ctotal=3C4
4. Voltage Across Each Capacitor:
- Let the voltage across the series combination be V.
- The voltage across C1 (with dielectric) is:
V1=QC1=Q3C
- The voltage across C2 (with air) is:
V2=QC2=QC
5. Relation Between Voltages:
- Since the total voltage V=V1+V2:
V=Q3C+QC
- To combine these, find a common denominator:
V=Q3C+3Q3C=4Q3C
6. Electric Field Calculation:
- The electric field E in a capacitor is given by:
E=Vd
- For C1:
E1=V1d=Q3Cd=Q3Cd
- For C2:
E2=V2d=QCd=QCd
7. Finding the Ratio:
- The ratio of the electric fields is:
E1E2=Q3CdQCd=13
Final Answer:
The ratio of the electric field inside the two capacitors is:
E1E2=13
- Let C1 be the capacitor with a dielectric slab of dielectric constant K=3.
- Let C2 be the identical capacitor with air between the plates.
- The capacitance of C1 with the dielectric is given by:
C1=K⋅C=3C
- The capacitance of C2 (with air) remains:
C2=C
- The total capacitance Ctotal for capacitors in series is given by:
1Ctotal=1C1+1C2
- Substituting the values:
1Ctotal=13C+1C=1+33C=43C
- Therefore, the total capacitance is:
Ctotal=3C4
- Let the voltage across the series combination be V.
- The voltage across C1 (with dielectric) is:
V1=QC1=Q3C
- The voltage across C2 (with air) is:
V2=QC2=QC
- Since the total voltage V=V1+V2:
V=Q3C+QC
- To combine these, find a common denominator:
V=Q3C+3Q3C=4Q3C
- The electric field E in a capacitor is given by:
E=Vd
- For C1:
E1=V1d=Q3Cd=Q3Cd
- For C2:
E2=V2d=QCd=QCd
- The ratio of the electric fields is:
E1E2=Q3CdQCd=13
The ratio of the electric field inside the two capacitors is:
E1E2=13
Topper's Solved these Questions
- BOOK - VMC MODULES ENGLISHCHAPTER - CAPACITORSEXERCISE - LEVEL 240videos
CAPACITORS
- BOOK - VMC MODULES ENGLISHCHAPTER - CAPACITORSEXERCISE - JEE Main (Archive) LEVEL-136videos
CAPACITORS
- BOOK - VMC MODULES ENGLISHCHAPTER - CAPACITORSEXERCISE - LEVEL 0 (LONG ANSWER TYPE)3videos
CAPACITORS
- BOOK - VMC MODULES ENGLISHCHAPTER - BASIC MATHEMATICS & VECTORSEXERCISE - Impeccable50videos
BASIC MATHEMATICS & VECTORS
- BOOK - VMC MODULES ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - IN-CHAPTER EXERCISE-F10videos
CURRENT ELECTRICITY
Similar Questions
A dielectric slab is inserted between the plates of an isolated capacitor. The force between the plates will
View SolutionA capacitor of capacitance 2μF has a dielectric slab of dielectric constant 8 between its plates. The slab covers the entire volume between the plates. This capacitor is connected to a battery if EMF 20 V and fully charged Now with the battery still connected, the slab is removed from the capactor. during the removal, the work done by the battery is (in mJ).
View SolutionA parallel plate capacitor has a dielectric slab of dielectric constant K between its plates that covers 1/3 of the area of its plates, as shown in the figure. The total capacitance of the capacitor is C while that of the portion with dielectric in between is C1. When the capacitor is charged, the plate area covered by the dielectric gets charge Q1 and the rest of the area gets charge Q2. The electric field in the dielectric is E1 and that in the other portion is E2. Choose the correct option/options, ignoring edge effects.
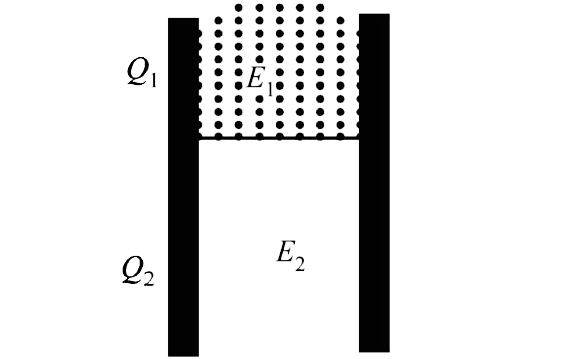 View Solution
View SolutionA capacitor of 60 pF charged to 20 volt. Now battery is removed and then this capacitor is connected to another identical uncharged capacitor. Find heat loss in nJ
View SolutionA capacitor of 60 pF charged to 20 volt. Now battery is removed and then this capacitor is connected to another identical uncharged capacitor. Find heat loss in nJ
View SolutionIn a parallel-plate capacitor, the region between the plates is filled by a dielectric slab. The capacitor is connected to a cell and the slab is taken out. Then
View SolutionA dielectric slab is inserted between the plates of an isolatted charged capacitor. Which of the following quantities will remain the same?
View SolutionA dielectric slab is inserted between the plates of a capacitor. The charge on the capacitor is Q and the magnitude of the induced charge on each surface of the dielectric is Q'.
View SolutionTwo identical capacitor C1 and C2 are connected in series with a battery. They are fully charged. Now a dielectric slab is inserted between the plates of C2. The potential across C1 will :
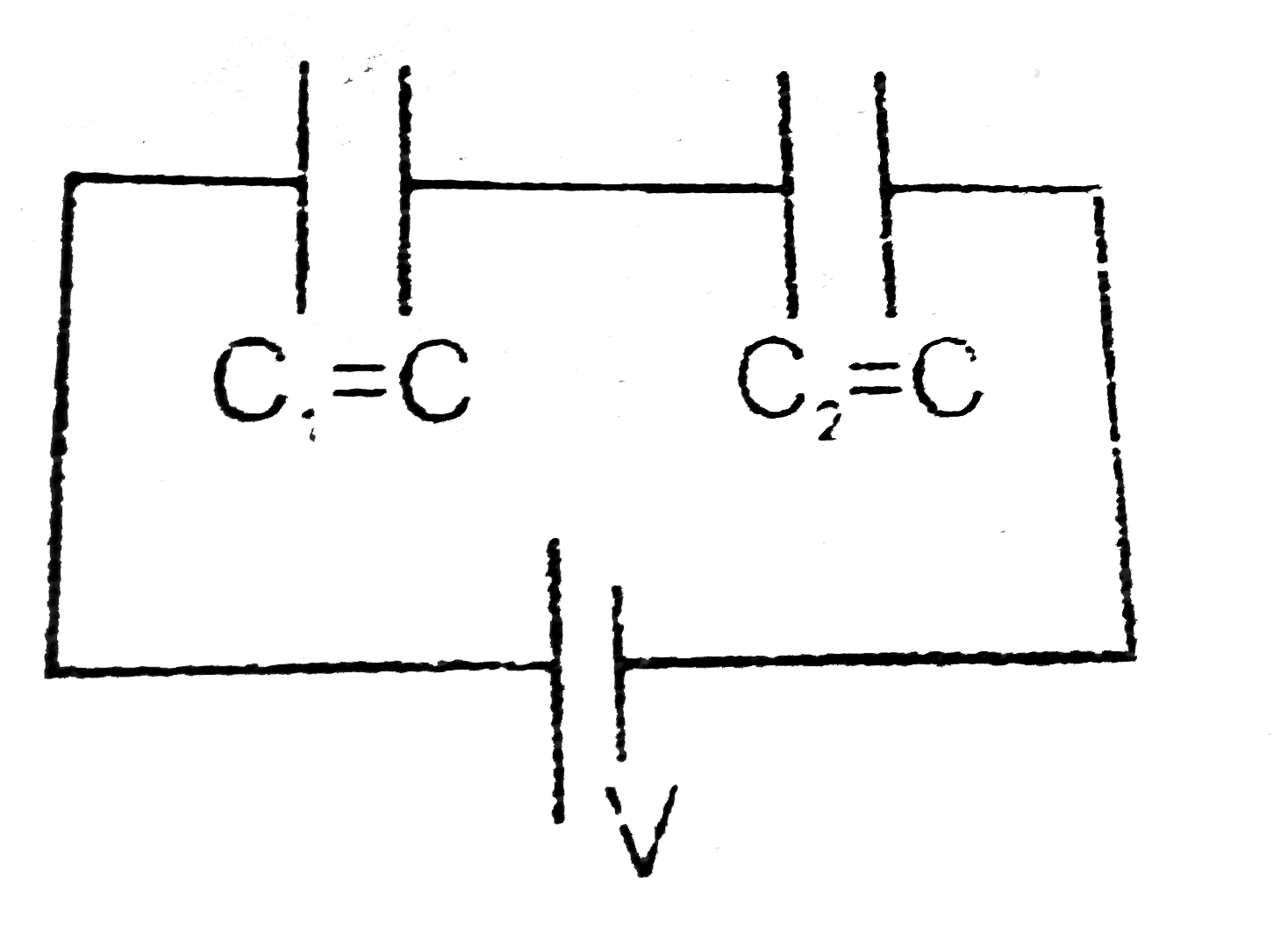 View Solution
View SolutionA capacitor and a bulb are connected in series with a source of alternating emf. If a dielectric slab is inserted between the plates of the capacitor, then
View Solution

