If acceleration a(t)=3t2 and initial velocity u=0 m/s , then the velocity of the particle after time t=4 s
73 cm/s
80 cm/s
64 cm/s
90 cm/s
The correct Answer is:A
To find the velocity of the particle after time t=4 seconds, given the acceleration a(t)=3t2 and the initial velocity u=0 m/s, we can follow these steps:
1. Understand the Relationship Between Acceleration and Velocity:
The acceleration a(t) is the derivative of velocity v(t) with respect to time t:
a(t)=dvdt
Given a(t)=3t2, we can write:
dvdt=3t2
2. Separate Variables:
We can rearrange the equation to separate the variables v and t:
dv=3t2dt
3. Integrate Both Sides:
We will integrate both sides. The left side will be integrated with respect to v from 0 to v, and the right side will be integrated with respect to t from 0 to 4:
∫v0dv=∫403t2dt
4. Calculate the Right Side Integral:
The integral of 3t2 with respect to t is:
∫3t2dt=t3
Evaluating this from 0 to 4:
(t3)40=43−03=64−0=64
5. Set Up the Equation:
Now, substituting back into our equation:
v−0=64
Therefore, the final velocity v is:
v=64 m/s
Final Answer:
The velocity of the particle after t=4 seconds is 64 m/s.
---
The acceleration a(t) is the derivative of velocity v(t) with respect to time t:
a(t)=dvdt
Given a(t)=3t2, we can write:
dvdt=3t2
We can rearrange the equation to separate the variables v and t:
dv=3t2dt
We will integrate both sides. The left side will be integrated with respect to v from 0 to v, and the right side will be integrated with respect to t from 0 to 4:
∫v0dv=∫403t2dt
The integral of 3t2 with respect to t is:
∫3t2dt=t3
Evaluating this from 0 to 4:
(t3)40=43−03=64−0=64
Now, substituting back into our equation:
v−0=64
Therefore, the final velocity v is:
v=64 m/s
The velocity of the particle after t=4 seconds is 64 m/s.
Topper's Solved these Questions
- MOTION IN A STRAIGHT LINE & PLANE BOOK - VMC MODULES ENGLISHCHAPTER - MOTION IN A STRAIGHT LINE & PLANE EXERCISE - EFFICIENT
50 Videos
- MOTION IN A STRAIGHT LINE & PLANE BOOK - VMC MODULES ENGLISHCHAPTER - MOTION IN A STRAIGHT LINE & PLANE EXERCISE - IMPECCABLE
52 Videos
- MOTION IN A STRAIGHT LINE & PLANE BOOK - VMC MODULES ENGLISHCHAPTER - MOTION IN A STRAIGHT LINE & PLANE EXERCISE - IMPECCABLE
52 Videos
- MOCK TEST 9BOOK - VMC MODULES ENGLISHCHAPTER - MOCK TEST 9EXERCISE - PHYSICS (SECTION 2)
5 Videos
- Motion in Straight Line BOOK - VMC MODULES ENGLISHCHAPTER - Motion in Straight Line EXERCISE - IN-CHAPTER EXERCISE-J
10 Videos
Similar Questions
The acceleration of paticle varies with time as :
a(t)=3t2+4
If the initial velocity of particle is 2m/s, find the velocity of particle at t=3sec.
View SolutionThe velocity of a particle is zero at time t = 2s, then
View SolutionFollowing are three equations of motion
S(g)=ut+12at2v(s)=√u2+2asv(t)=u+at
Where ,S,u,t,a,v are respectively the displacement ( dependent variable ), initial ( constant ), time taken ( independent variable ), acceleration ( constant ) and final velocity ( dependent variable ) of the particel after time t.
Find the displacement of the particle when its velocity becomes 10m/s if acceleration is 5m/s2 all through -
View SolutionFollowing are three equations of motion
S(g)=ut+12at2v(s)=√u2+2asv(t)=u+at
Where ,S,u,t,a,v are respectively the displacement ( dependent variable ), initial ( constant ), time taken ( independent variable ), acceleration ( constant ) and final velocity ( dependent variable ) of the particel after time t.
Find the velocity of a particle after 10 seconds if its acceleration is zero in interval (0 to 10s)
View SolutionFollowing are three equations of motion
S(g)=ut+12at2v(s)=√u2+2asv(t)=u+at
Where ,S,u,t,a,v are respectively the displacement ( dependent variable ), initial ( constant ), time taken ( independent variable ), acceleration ( constant ) and final velocity ( dependent variable ) of the particel after time t.
Find the displacement of a particle after 10 seconds starting from rest with a uniform acceleration of 2m/s2
View SolutionA particle moves vertically with an upwards initial speed v0=10.5ms−1. If its acceleration varies with time as shown in a-t graph in figure, find the velocity of the particles at t = 4s.
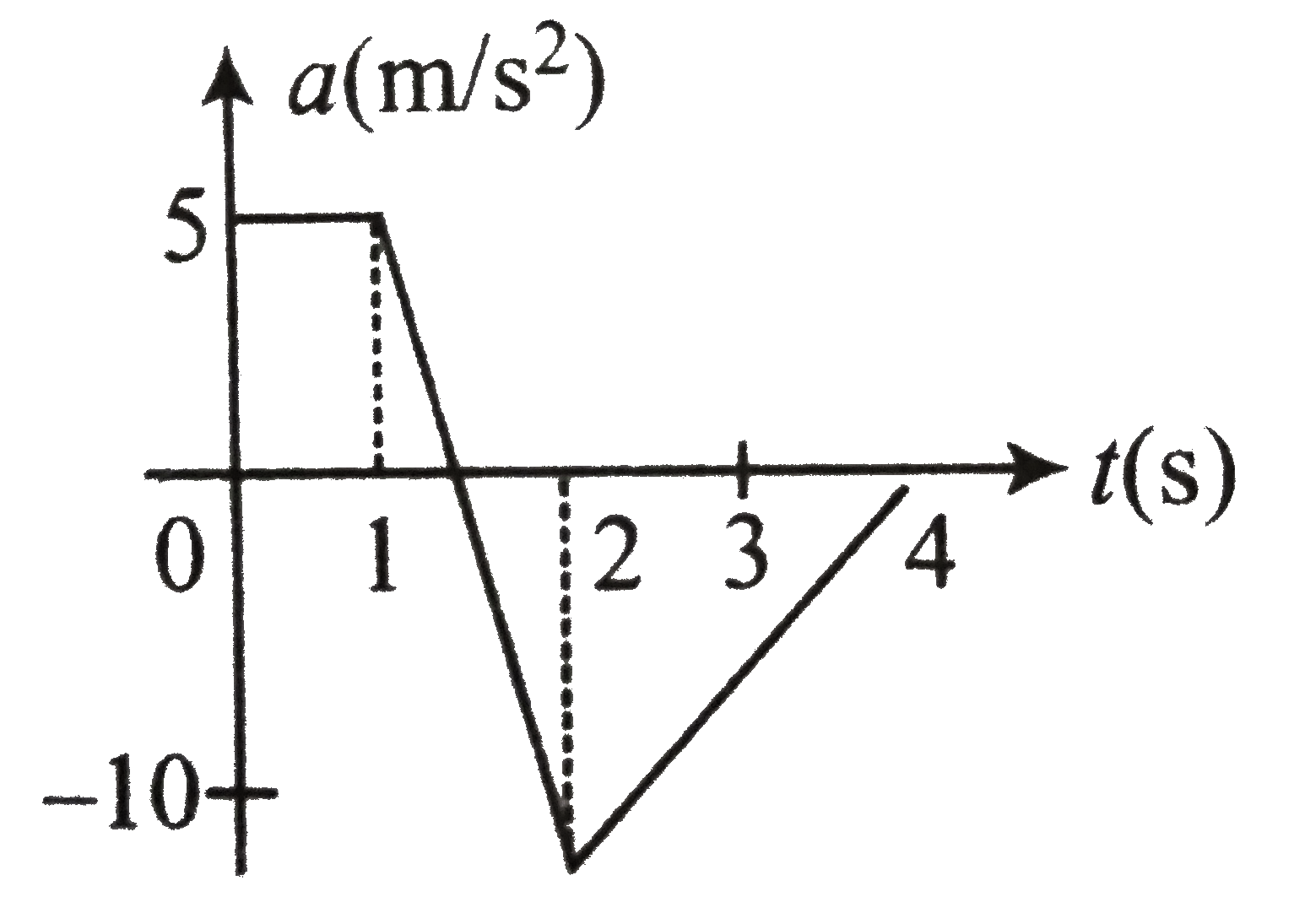
View SolutionAcceleration of a particle at any time t is →a=(2tˆi+3t2ˆj)m/s2.If initially particle is at rest, find the velocity of the particle at time t=2s
View Solutionvelocity-time graph of a particle moving in a straight line is as shown in figure. At time t=0, velocity of the particle is zero. Find
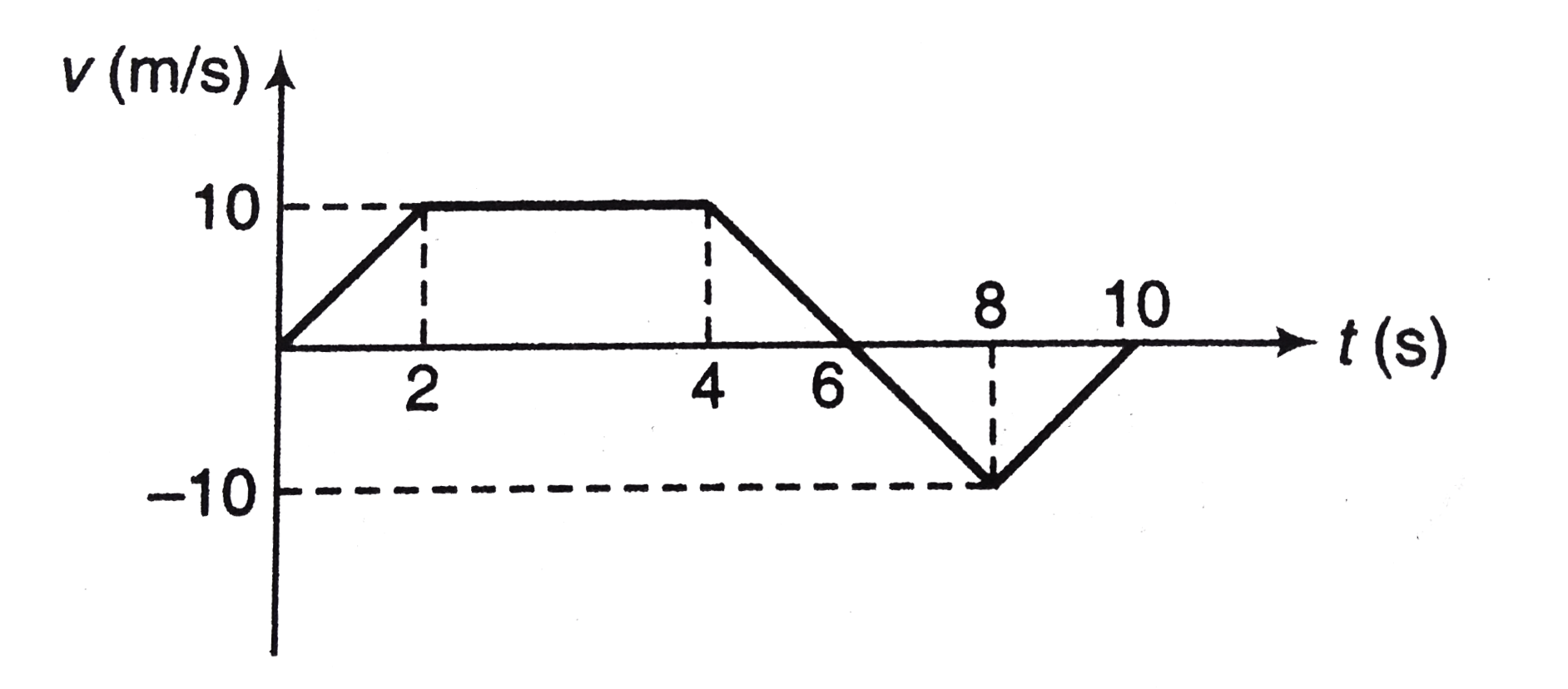
(a) average acceleration in a time interval from t=6s to t=12s,
(b) velocity of the particle at t=14s.
View SolutionThe velocity of a particle moving with constant acceleration at an instant t0 is 10m/s After 5 seconds of that instant the velocity of the particle is 20m/s. The velocity at 3 second before t0 is:-
View SolutionThe acceleration-time graph of a particle moving along x-axis is shown in the figure. If the particle starts with velocity 3 m/s at t = 0, find the velocity of particle at t = 4 s.

View Solution