The graph between the angular momentum J and angular velocity ω will be :-
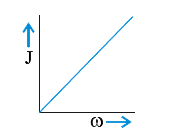
The correct Answer is:A
To determine the graph between angular momentum J and angular velocity ω, we start with the fundamental relationship between these two quantities.
1. Understanding the Relationship:
The angular momentum J of a rotating object is given by the formula:
J=Iω
where I is the moment of inertia and ω is the angular velocity.
2. Identifying the Equation Form:
The equation J=Iω can be compared to the standard linear equation form:
y=mx+c
Here, J corresponds to y, ω corresponds to x, I is the slope m, and the intercept c is 0.
3. Analyzing the Intercept:
Since the equation has no constant term (the intercept c=0), the graph will pass through the origin (0,0). This means that when ω=0, J will also be 0.
4. Determining the Slope:
The slope of the graph is equal to the moment of inertia I. This means that the graph will be a straight line with a slope equal to I.
5. Sketching the Graph:
- The graph will start at the origin (0,0).
- It will be a straight line that increases linearly as ω increases.
- The line will have a positive slope, indicating that as angular velocity increases, angular momentum also increases proportionally.
6. Selecting the Correct Graph:
Based on the above analysis, we can conclude that the correct graph will be a straight line passing through the origin with a positive slope.
Conclusion:
The correct option is Option A, which represents the linear relationship between angular momentum J and angular velocity ω with no intercept.
The angular momentum J of a rotating object is given by the formula:
J=Iω
where I is the moment of inertia and ω is the angular velocity.
The equation J=Iω can be compared to the standard linear equation form:
y=mx+c
Here, J corresponds to y, ω corresponds to x, I is the slope m, and the intercept c is 0.
Since the equation has no constant term (the intercept c=0), the graph will pass through the origin (0,0). This means that when ω=0, J will also be 0.
The slope of the graph is equal to the moment of inertia I. This means that the graph will be a straight line with a slope equal to I.
- The graph will start at the origin (0,0).
- It will be a straight line that increases linearly as ω increases.
- The line will have a positive slope, indicating that as angular velocity increases, angular momentum also increases proportionally.
Based on the above analysis, we can conclude that the correct graph will be a straight line passing through the origin with a positive slope.
The correct option is Option A, which represents the linear relationship between angular momentum J and angular velocity ω with no intercept.
Topper's Solved these Questions
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBOOK - VMC MODULES ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - IMPECCABLE
56 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBOOK - VMC MODULES ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - ENABLE
50 Videos
- SYSTEM OF A PARTICLES & ROTATIONAL MOTIONBOOK - VMC MODULES ENGLISHCHAPTER - SYSTEM OF A PARTICLES & ROTATIONAL MOTIONEXERCISE - IN-CHAPTER EXERCISE F
10 Videos
- UNITS, MEASUREMENTS & ERRORSBOOK - VMC MODULES ENGLISHCHAPTER - UNITS, MEASUREMENTS & ERRORSEXERCISE - IN - CHAPTER EXERCISE - B
10 Videos
Similar Questions
Angular momentum is
View SolutionAngular momentum is
View SolutionAngular momentum is
View SolutionThe angular momentum is?
View SolutionRelation between magnetic moment and angular velocity is -
View SolutionThe angular momentum of d electron is
View SolutionThe graph between wave number (→v) and angular frequency (ω) is
View SolutionThe angular momentum of p electron is
View SolutionThe rate of change of angular momentum is called
View SolutionAngular momentum is conserved
View Solution
