Two curves C_(1)equiv[f(y)]^(2//3)+[f(x)]^(1//3)=0 and C_(2)equiv[f(y)]^(2//3)+[f(x)]^(2//3)=12, satisfying the relation "(x-y)f(x+y)-(x+y)f(x-y)=4xy(x^(2)-y^(2))
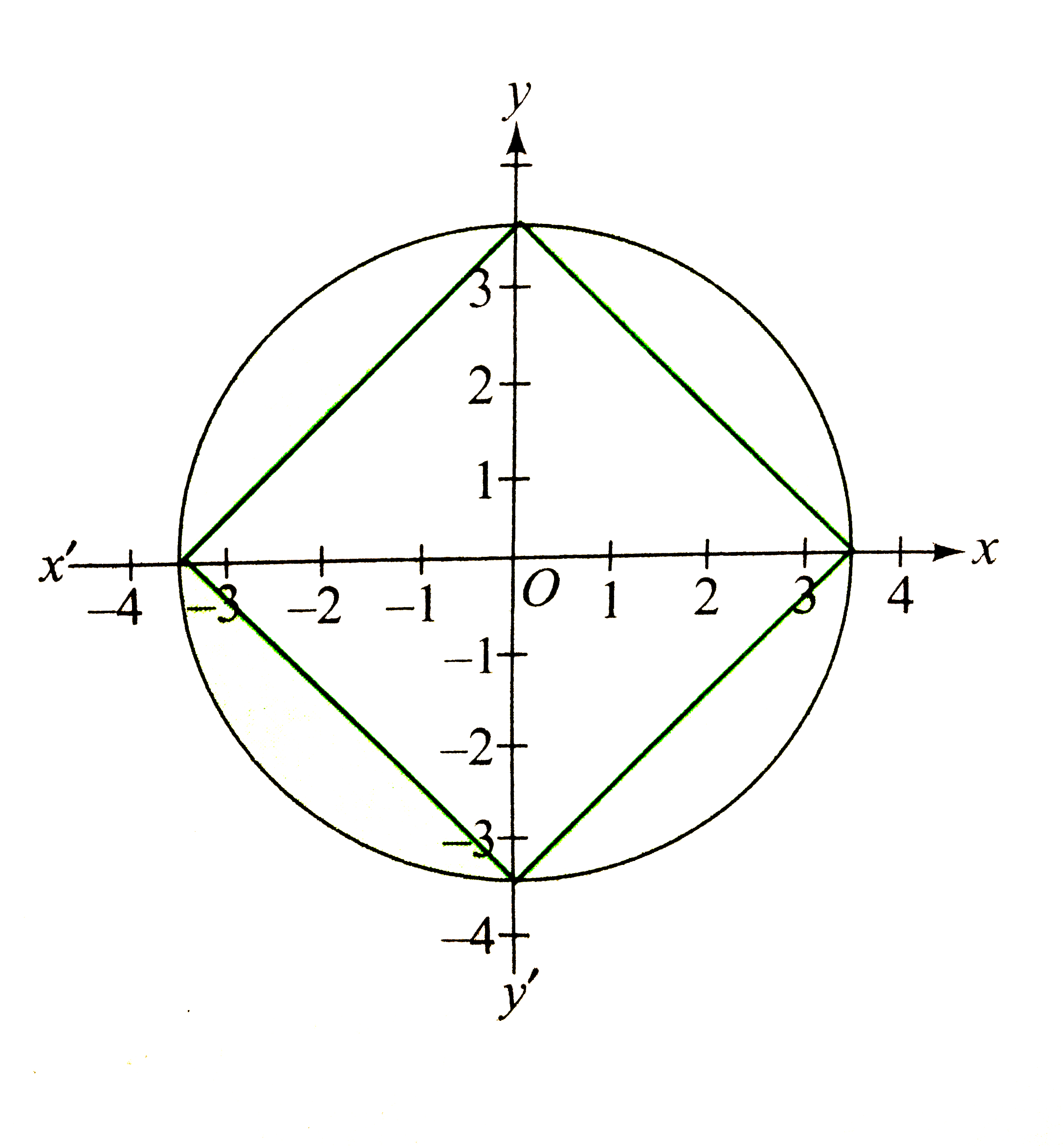
The area bounded by the curve C_(2) and |x|+|y|=sqrt(12) isSolution in Tamil
12π−24 sq. units
6−√12 sq. units
2√12−6 sq. units
None of these
The correct Answer is:A
Required area = Area of circle- Area of square
=12π−24 sq. units.
Topper's Solved these Questions
- BOOK - CENGAGECHAPTER - AREAEXERCISE - Exercise (Matrix)4videos
AREA
- BOOK - CENGAGECHAPTER - AREAEXERCISE - Exercise (Numerical)16videos
AREA
- BOOK - CENGAGECHAPTER - AREAEXERCISE - Exercise (Multiple)10videos
AREA
- BOOK - CENGAGECHAPTER - APPLICATIONS OF DERIVATIVESEXERCISE - Subjective Type2videos
APPLICATIONS OF DERIVATIVES
- BOOK - CENGAGECHAPTER - AREA UNDER CURVESEXERCISE - Question Bank10videos
AREA UNDER CURVES
Similar Questions
Match the following lists :
 08:07View Solution
08:07View SolutionThe area enclosed by the curve C:y=x√9−x2(x≥0) and the x-axis is___.
05:23View SolutionLet A(k) be the area bounded by the curves y=x2−3 and y=kx+2 The range of A(k) is (10√53,∞) The range of A(k) is (20√53,∞) If function k→A(k) is defined for k∈[−2,∞ ), then A(k) is many-one function. The value of k for which area is minimum is 1.
View SolutionProve that for λ>1, the equation xlogx+x=λ has least one solution in [1,λ].
View SolutionThe area of the region (s) enclosed by the curves y=x2 and y=√|x| is
View SolutionIf a function satisfies (x−y)f(x+y)−(x+y)f(x−y)=2(x2y−y3)∀x,y∈Randf(1)=2, then
06:07View SolutionIf a curve y=f(x) satisfies y''x^(2)+x(y')^(2)=2xy',f(0)=0 and f'(1)=1 then f(x) is
10:55View SolutionTwo curves C1≡[f(y)]2/3+[f(x)]1/3=0andC2≡[f(y)]2/3+[f(x)]2/3=12, satisfying the relation (x−y)f(x+y)−(x+y)f(x−y)=4xy(x2−y2)
The area bounded by C1andC2 is04:20View SolutionTwo curves C1≡[f(y)]2/3+[f(x)]1/3=0andC2≡[f(y)]2/3+[f(x)]2/3=12, satisfying the relation (x−y)f(x+y)−(x+y)f(x−y)=4xy(x2−y2)
The area bounded by the curve C2and|x|+|y|=√12 is12:34View SolutionTwo curves C1≡[f(y)]2/3+[f(x)]1/3=0andC2≡[f(y)]2/3+[f(x)]2/3=12, satisfying the relation (x−y)f(x+y)−(x+y)f(x−y)=4xy(x2−y2)
The area bounded by C1andx+y+2=0 is04:51View SolutionIf C1≡y=11+x2andC2≡y=x22 be two curve lying in XY plane. Then
06:16View Solutionमान लीजिये f:R→R में एक अवकलनीय फलन है जो प्रतिबन्ध को सन्तुष्ट करता है
f.(x+y3)=2+f(x)+f(y)3,∀x,y∈Rतथा f'(2)=2
यदि g(x)=|f(|x|)−3|,Ax∈R हो, तो g(x) के लिएView SolutionIf f(x+y3)=2+f(x)+f(y)3 for all x,y f'(2)=2 then find f(x)
02:39View SolutionTwo curves C1≡[f(y)]2/3+[f(x)]1/3=0andC2≡[f(y)]2/3+[f(x)]2/3=12, satisfying the relation (x−y)f(x+y)−(x+y)f(x−y)=4xy(x2−y2)
The area bounded by the curve C1andC2 isView SolutionTwo curves C1≡[f(y)]2/3+[f(x)]1/3=0andC2≡[f(y)]2/3+[f(x)]2/3=12, satisfying the relation (x−y)f(x+y)−(x+y)f(x−y)=4xy(x2−y2)
The area bounded by the curve C2and|x|+|y|=√12 is02:13View Solution

