Determine resultant amplitude after super position of given four waves with help of phasor diagram. y1=15sinωtmm,y2=9sin(ωt−π/2)mm,y3=7sin(ωt+π/2)mm & y4=13sinωtmm.Solution in Gujrati
The correct Answer is:2.83mm
_E01_105_S01.png)
A=2√2=2.828mm≅2.83mm
Topper's Solved these Questions
- BOOK - ALLEN CHAPTER - WAVES AND OSCILLATIONSEXERCISE - Part-1(Exercise-04)[B]13videos
WAVES AND OSCILLATIONS
- BOOK - ALLEN CHAPTER - WAVES AND OSCILLATIONSEXERCISE - Part-1(Exercise-05)[A]28videos
WAVES AND OSCILLATIONS
- BOOK - ALLEN CHAPTER - WAVES AND OSCILLATIONSEXERCISE - Part-1(Exercise-03)31videos
WAVES AND OSCILLATIONS
- BOOK - ALLEN CHAPTER - SEMICONDUCTORSEXERCISE - Part-3(Exercise-4)50videos
SEMICONDUCTORS
Similar Questions
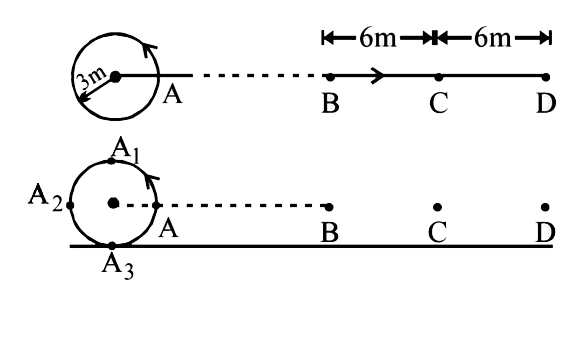
A source of sound is moving along a circular orbit of radius 3meter with an angular velocity of 10rad/s. A sound detector located far away from the source is executing linear simple harmonic motion along the line BD with an amplitude BC=CD=6meters. The frequency of oscillation of the detector is 5π per second. The source is at the point A when the detector is at the point B. If the source emits a continuous sound wave of frequency 340Hz, Find the maximum and the minimum frequencies recorded by the detector.
03:12View SolutionTube A has both ends open while tube B has one closed, otherwise they are identical. The ratio of fundamental frequency of tube AandB is
01:58View SolutionStatement-1 : Sound travels faster in moist air
Statement-2 : The density of moist air is less then density of dry air.03:23View SolutionAt absolute zero temperature Si acts as ……..
03:44View SolutionFind the resultant amplitude and the phase difference between the resultant wave and the first wave , in the event the following waves interfere at a point , y_(1) = ( 3 cm) sin omega t , y_(2) = ( 4 cm) sin (omega t + (pi)/( 2)), y_(3) = ( 5 cm ) sin ( omega t + pi)
02:16View SolutionThe amplitude of the vibrating particle due to superposition of two SHMs,
y1=sin(ωt+π3)andy2=sinωt is :04:34View SolutionFour simple harmonic vibrations y_(1)=8 sin omega t , y_(2)= 6 sin (omega t+pi//2) , y_(3)=4 sin (omega t+pi) , y_(4)=2sin(omegat+3pi//2) are susperimposed on each other. The resulting amplitude and phase are respectively.
02:29View SolutionTwo waves are represented by y_(1)= a sin (omega t + ( pi)/(6)) and y_(2) = a cos omega t . What will be their resultant amplitude
02:50View SolutionThe resultant ampiltude of a vibrating particle by the superposition of the two waves y_(1) = a sin ( omega t + (pi)/(3)) and y_(2) = a sin omega t is :
03:00View SolutionThe equations for displacement of two light waves forming interference pattern are y_(1) = 4 sin omega t and y_(2) =3 sin (omega t+ (pi)/(2)) . Determine the amplitude of the resultant wave.
07:36View SolutionEquations of two light waves are y1= 4 sin ω t and y2=3sin(ωt+π2). What is the amplitude of the resultant wave as they superpose on each other?
View SolutionWhen the waves y_(1) = A sin omega t and y_(2) = A cos omega t are superposed, then resultant amplitude will be
02:43View SolutionThe displacement of two interfering light waves are y1=4sinωt and y2=3cosωt. The amplitude of the resultant wave is (y1 and y2 are in CGS system)
03:50View Solutiony1=4sinωt तथा y2=3sin(ωt+π2) तरंगो के अध्यारोपण से बनने वाली तरंग का परिणामी आयाम होगा
01:55View SolutionThe displacement of two interfering light waves are y_(1)=4 sin omega t" and "y_(2)= 3 cos (omega t) . The amplitude of the resultant waves is (y_(1)" and "y_(2) are in CGS system)
View SolutionTwo waves are represented by y1=asin(ωt+π6)andy2=acosωt. What will be their resultant amplitude
View Solution